Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-11T08:19:44.225Z Has data issue: false hasContentIssue false
  • English
  • Français

A Stochastic Maximin Fixed-Point Equation Related to Game Tree Evaluation

Part of: Distribution theory - Probability Theory of computing

Published online by Cambridge University Press:  14 July 2016

Gerold Alsmeyer  and
Matthias Meiners
Gerold Alsmeyer*
Affiliation:
Westfälische Wilhelms-Universität Münster
Matthias Meiners*
Affiliation:
Westfälische Wilhelms-Universität Münster
*
∗Postal address: Institut für Mathematische Statistik, Fachbereich Mathematik und Informatik, Westfälische Wilhelms-Universität Münster, Einsteinstrasse 62, D-48149 Münster, Germany.
∗Postal address: Institut für Mathematische Statistik, Fachbereich Mathematik und Informatik, Westfälische Wilhelms-Universität Münster, Einsteinstrasse 62, D-48149 Münster, Germany.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

After suitable normalization the asymptotic root value W of a minimax game tree of order b ≥ 2 with independent and identically distributed input values having a continuous, strictly increasing distribution function on a subinterval of R appears to be a particular solution of the stochastic maximin fixed-point equation W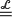 ξ max1≤i≤bmin1≤j≤bWi,j, where Wi,j are independent copies of W and 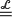denotes equality in law. Moreover, ξ= g'(α) > 1, where g(x) := (1 − (1 − x)b)b and α denotes the unique fixed point of g in (0, 1). This equation, which takes the form F(t) = g(F(t/ξ)) in terms of the distribution function F of W, is studied in the present paper for a reasonably extended class of functions g so as to encompass more general stochastic maximin equations as well. A complete description of the set of solutions F is provided followed by a discussion of additional properties such as continuity, differentiability, or existence of moments. Based on these results, it is further shown that the particular solution mentioned above stands out among all other ones in that its distribution function is the restriction of an entire function to the real line. This extends recent work of Ali Khan, Devroye and Neininger (2005). A connection with another class of stochastic fixed-point equations for weighted minima and maxima is also discussed.

Keywords

Minimax game treestochastic fixed pointmaximin equationmomentsweighted minimaweighted maxima

MSC classification

Secondary: 60E05: Distributions 68Q25: Analysis of algorithms and problem complexity
Type
Research Article
Information
Journal of Applied Probability , Volume 44 , Issue 3 , September 2007 , pp. 586 - 606
Copyright
Copyright © Applied Probability Trust 2007 

References

[1] Aldous, D. and Bandhyopadhyay, A. (2005). A survey of max-type recursive distributional equations. Ann. Appl. Probab. 15, 1047–1110.CrossRefGoogle Scholar
[2] Ali Khan, T., Devroye, L. and Neininger, R. (2005). A limit law for the root value of minimax trees. Electron. Commun. Prob. 10, 273–281.Google Scholar
[3] Alsmeyer, G. and Rösler, U. (2007). A stochastic fixed-point equation for weighted minima and maxima. To appear in Ann. Inst. H. Poincaré Prob. Statist. Google Scholar
[4] Jagers, P. and Rösler, U. (2004). Stochastic fixed points for the maximum. In Mathematics and Computer Science, III, eds Drmota, M. et, Birkhäuser, Basel, pp. 325–338.CrossRefGoogle Scholar
[5] Meiners, M. (2006). Über stochastische Maximin-Fixpunktgleichungen. , University of Münster.Google Scholar
[6] Neininger, R. and Rüschendorf, L. (2005). Analysis of algorithms by the contraction method: additive and max-recursive sequences. In Interacting Stochastic Systems, eds Deuschel, J. D. and Greven, A., Springer, Heidelberg, pp. 435–450.Google Scholar
[7] Pearl, J. (1980). Asymptotic properties of minimax trees in game-searching procedures. Artificial Intelligence 14, 113–138.Google Scholar