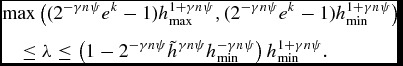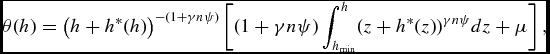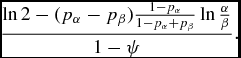1. INTRODUCTION
This paper studies an economic model of marriage which is entirely based on the biological differences between men and women. The two most important differences are that, in nature, women know for sure whom their children are, while men don’t; and that men can potentially have children with a large number of women, while the converse is not true for women.Footnote 1
Because of the first of these biological differences, there are gains from trade between men and women. Women can sell to men a guarantee that her children are his – a property I call legitimacy. Men are willing to pay for legitimacy because they can raise their utility by investing in their own children. This will hold provided men derive utility from the quantity and quality of children.
However, to provide such a guarantee, the woman must credibly commit to mate only with her husband – which is the key feature of the traditional marriage contract.Footnote 2 Furthermore, the second biological difference between men and women implies that women have an opportunity cost of marrying. Instead, they could mate with men with the most desirable characteristics, and improve the genotype of their offsprings. Because these men’s gametes are not scarce, they have no cost of mating with as many women as possible, and they benefit from it as long as they derive utility from having illegitimate children.Footnote 3 By marrying, a woman foregoes the superior genetic material of the most attractive menFootnote 4; on the other hand she benefits from the father’s investment in the children’s human capital and from any transfer from her husband. This trade-off will hold as long as men have different observable traits that are genetically heritable and valued by the parents in their children. In the model, it is assumed that children of more desirable men (the alpha men) are more productive in acquiring human capital.
This paper derives the implications of these observations for the pattern of matching in marriage markets, the dynamics of human capital accumulation, and the evolution of the gene pool. A key consequence of the trade-off faced by women is that marriage markets will naturally tend to be hypergamous – that is, a marriage is more likely to be viable, the greater the man’s human capital, and the lower the woman’s human capital. The reason is that the utility loss from marrying a beta man instead of an alpha man is not transferable; therefore, the greater a woman’s human capital, the lower her marginal utility of consumption, and the larger the transfer that she must get from a man in order to be compensated for her foregone mating opportunities. The opposite logic is at work for men: the larger their human capital, the lower their marginal utility of consumption, and the greater their willingness to pay for legitimate children.
The paper is organized as follows. In Section 2 the model is set up, and we derive the equilibrium conditions for a “state of nature” where marriage does not exist, and for a society where marriage exists. We use a model of the intergenerational transmission of human capital with sexual reproduction, endogenous mating and household formation, and heritable genetic differences between people (alphas vs. betas). People derive utility from consumption, and the quantity and quality of their children. Their income is proportional to their human capital, which depends on their genes and on their parents’ investment. They allocate their income between their consumption and their children’s human capital accumulation. A key result is that in the State of Nature, only the alpha men mate; the beta men are driven out of the market as they cannot credibly buy legitimacy from women. We then derive a condition for marriage to yield a positive surplus relative to each party remaining single. This condition exhibits hypergamy: it is more likely to hold, the greater the man’s human capital, and the smaller the woman’s human capital.
Section 3 derives and discusses the model’s predictions for the mating pattern. We characterize the equilibrium assignment of husbands to wives, and perform comparative statics with respect to this assignment. A perfectly competitive marriage market is assumed. It is shown that perfect assortative matching arises and that this is due to the public good aspect of the children in the woman’s and the man’s utility functionFootnote 5. Because of the hypergamy effect, we can also show that the equilibrium can only be of two types. In the “Victorian” type, all agents marry somebody of the same rank in the distribution of income. In the “SATC” type, women marry men who have a greater rank than themselves. There is a mass of unmarried men at the bottom of the distribution of human capital, and a mass of single women at the top of that distribution.Footnote 6 It is shown that the economy switches from a Victorian to an SATC equilibrium as inequality goes up; one interpretation is that less skilled women underbid more skilled ones for their husbands, which in equilibrium drives the skilled woman’s share in bargaining down. As a result, the most skilled women end up better-off unmarried, and mating with alpha men. The same mechanism explains why the equilibrium may be SATC even though all homogamous marriages would be viable: starting from a homogamous assignment, less skilled women would successfully underbid more skilled ones by accepting a lower share of the surplus, thus driving them out of the marriage market. This suggests that perfect competition in marriage markets may reduce the number of marriages relative to other institutional arrangements for matching husbands and wives together.Footnote 7
The model sheds light on how marriage affects the returns to human capital for men and women. In the State of Nature, these returns are larger for women than for men because they use their human capital both to invest in their children and to increase their own consumption. When marriage exists, this effect is equalized between men and women, but additional interesting effects arise. The returns to human capital depend on how the surplus is split between men and women at different levels of human capital: when inequality is large, competition for mates from low-skill women generates a downward profile of the woman’s share in output as her human capital goes up. This tends to reduce the returns to human capital for women relative to men. Another effect arises if the equilibrium is SATC: a man has a lower quality spouse than a woman with the same level of human capital; therefore his marginal utility of consumption and his return to human capital are higher. Finally, in an SATC equilibrium, acquiring human capital may make a man eligible for marriage, while it may eliminate the benefits of marriage for a woman. This, too, tends to reduce the return to human capital for women relative to men. On the other hand, in an SATC equilibrium beta men at the bottom of the distribution of skills are single and therefore have the same low return to human capital as in the State of Nature. Following this analysis, we may speculate that the decline of marriage may have something to do with men losing ground relative to women in higher education, relative to an initial situation where they did acquire more education than womenFootnote 8.
These predictions are consistent with the microeconomic evidence provided by Bertrand et al. (Reference Bertrand, Pan and Kamenica2013). They show that the distribution of earnings shares in a couple is highly skewed toward the zone where the wife’s share is below 1/2 – with a sharp drop as one moves from below 1/2 to above 1/2. They also provide evidence of greater marital instability and lower marital satisfaction in couples where the wife earns more than her husband. This is consistent with the hypergamy property studied in this paperFootnote 9. This phenomenon alone should, in the aggregate, be conducive to an SATC type equilibrium, more so as the earnings gap between women and men is being closed. However, the aggregate evidence is not so clear-cut. The Bureau of Labor Statistics (2013, Table 4) reports that in the United States the marriage rate increases systematically with men’s educational level, while it peaks at high school for women and then falls somewhat, but by a small amount. Furthermore, the marriage rate of women with less than a bachelor’s degree is significantly higher than for men of equal education (and the gap falls with the educational level), while the difference almost vanishes for women and men with a college degree. Similarly, the marriage rate by income groups rises much more steeply with income for men than for women, being virtually flat for the latter above 45,000$, and higher for women earning less than 10,000$ than for the next bracket (Figure 1). Nevertheless we do not observe a falling marriage rate for top female earners. The divergence predicted by the SATC equilibrium is more salient if one looks at divorce rates, which tend to fall with income for men but to go up with women, with the exception of the very top income bracket (Figure 2). Finally, for younger generations we do observe a greater proportion of never married women in high income groups than in low income groupsFootnote 10, while the corresponding figure is lower for men (Table 1)Footnote 11. Overall, this evidence is suggestive that the forces analyzed in this paper are at work in the US marriage market, although the current model is not equipped to explain the lower marriage rate of female high-school dropouts.

Figure 1. Marriage rates by income groups (CPS, 2013).

Figure 2. Divorce rates by income groups (CPS, 2013).
Table 1. Proportion never married, 35–39 years old.

Source: U.S. Census 2010.
While the evidence just discussed suggests that there may be some signs that an SATC-type equilibrium may be evolving in advanced societies – one example being the rise of single motherhood among high earning celebrities – throughout most of history many societies have imposed harsh penalties on out-of-wedlock births. At the end of Section 3, we use the model to study how such “sexual repression” affects the equilibrium. I show that a Victorian equilibrium then always exists and that the set of equilibrium bargaining shares is symmetrical and therefore not biased in favor of womenFootnote 12. Comparing such sexually repressed Victorian equilibria with the SATC equilibrium that would prevail absent sexual repression allows to compute the distribution of gains and losses from sexual repression. I show that it unambiguously benefits beta men and harms beta women; alpha men lose while alpha women are indifferent (and would gain if they valued marital fidelity per se). Thus, societies are more likely to implement sexual repression, the more they are politically dominated by beta menFootnote 13.
A consequence of the trade-off between father’s investment and good genes is that marriage does not necessarily enhance the quality of children. It increases parental investment but more children are of the less productive “beta” type. Whether marriage is beneficial for human capital accumulation depends on the productivity difference between alpha and beta types, as well as on the elasticity of a child’s human capital to parental investment. These aspects are discussed in Section 4. I first study whether a particular marriage improves the children’s human capital relative to the mother remaining single and mating with an alpha male. I show that the conditions are more stringent than for the marriage to just be viable; thus, for example, a marriage should be even more hypergamous than what is needed for its viability. These results clearly depend on the alphas being more productive rather than just more sexually attractive.
I then move to a general equilibrium dynamic analysis and study (in the Victorian case) the long-run distribution of human capital and genes in the marriage economy and compare it to the state of nature, and derive conditions for average steady state human capital to be larger under marriage than under the state of nature. Again, this need not always hold and will not if the productivity difference between the two genotypes is large enough. Section 5 summarizes and concludes.
This paper is related to the existing literature on marriage markets and on how this institution affects human capital accumulation. Overall, this literature has recognized that women are sellers in marriage markets either because of the sexual division of labor (Becker (Reference Becker1973, Reference Becker1974)) or because of the role played by women in reproduction (Aiyagari et al. (Reference Aiyagari, Greenwood and Guner2000), Edlund (Reference Edlund2006)).Footnote 14 This paper’s contribution is twofold. First, it brings back the abundance of male gametes and the existence of genetic differences in ability into the analysis, and accordingly identifies a trade-off for women as providers of legitimacy. Second, it fully analyses the consequences of that trade-off for the mating pattern and the evolution of the distribution of skills and genesFootnote 15. Also, the assortative mating property follows a different logic than in most papers in the literatureFootnote 16, as it is due to the public good aspect of children instead of complementarities between the husband’s and wife’s contributionsFootnote 17.
2. THE MODEL
2.1. Basic Setup
At each generation, people are either male or female. They consume, produce offspring and invest in the human capital of their offspring. Generations are non-overlapping and people only live one period, as far as their economically relevant activities are concerned.
Utility. People care about their consumption and their children’s human capital. I assume the same utility for men and women:
 $$\begin{equation*}
U=\ln c+\gamma \sum _{i=1}^{n_{c}}E\ln h_{i}^{\prime},
\end{equation*}$$
$$\begin{equation*}
U=\ln c+\gamma \sum _{i=1}^{n_{c}}E\ln h_{i}^{\prime},
\end{equation*}$$where c is consumption, nc the number of children, E the expectations operator, and h′i the human capital of a child. People only care about the human capital of their true genetic offspring, and cannot transmit any other asset.
Genotypes. People differ in their genetic endowment. There are two genotypes: alpha (α) and beta (β). I will assume that the alphas have better genes in that it is easier for them to accumulate human capital (model A). People then prefer alpha offsprings, all else equal. Alternatively, though, one may assume that alpha people are more sexually attractive (model B): mating with an alpha then only yields a utility gain.Footnote 18 As long as the analysis is confined to marriage markets, the two models are equivalent. But they differ in their implications for human capital accumulation.
Production and human capital accumulation. The production structure is as follows: an individual with h units of human capital can produce Ah units of output. This can be used either to consume or to invest in the children’s human capital. For an isolated individual, therefore, the budget constraint is c + nz = Ah, where z is the per-child investment in human capital.Footnote 19 The technology determining the offspring’s human capital is then given by
if the child is an alpha, and
if the child is a beta. We assume that α ⩾ β and that 0 ⩽ ψ ⩽ 1.Footnote 20
For simplicity, I will also assume that people invest in their children’s human capital before their children’s types are observed.
Mating and children. There is a perfectly competitive marriage market. Mating produces offsprings. Each intercourse produces one child. A woman can have up to n children. A man will have as many children as intercourses. I restrict the analysis to a zone where the contribution of children to utility is always positive, so that each woman will indeed have n children. I also assume that exactly n/2 of them are girls and n/2 are boys.
For simplicity, I assume that the type of a child (alpha vs. beta) only depends on the type of his or her fatherFootnote 21. I assume that alpha (resp. beta) fathers sire alpha children in proportion p α, (resp. p β ⩽ p α).
In what follows, the variables pertaining to men will be denoted by a star. Hence, for example, in a couple, h will denote the wife’s human capital and h* the husband’s human capital.
2.2. The State of Nature: No Marriage
I now study the equilibrium in the “State of Nature”, where individuals cannot contract on their mating behavior.
In the state of nature, men do not know who their offspring are. Consequently, they are not going to invest in the human capital of childrenFootnote 22. I will denote by a = p αlnα + (1 − p α)lnβ expected TFP in human capital accumulation if the father is alpha, and similarly for betas b = p βlnα + (1 − p β)lnβ. The following proposition characterizes the equilibrium in the State of NatureFootnote 23.
Proposition 1
Let
and
Assume that the initial distribution of h is such that ![]() $h\ge \underline{h}(\alpha) .$
$h\ge \underline{h}(\alpha) .$
Then, in equilibrium
(i) No β man mates.
(ii) Each woman mates n times with an α man, and invests
(2)in her offspring. $$\begin{equation}
z=\frac{\gamma \psi}{1+n\gamma \psi}Ah,
\end{equation}$$
$$\begin{equation}
z=\frac{\gamma \psi}{1+n\gamma \psi}Ah,
\end{equation}$$(iii) Each α -man mates n/p αtimes.
(iv) Utilities are given by
(3)for β men, $$\begin{equation}
\bar{U}_{\beta}^{\ast}(h^{\ast})=\ln Ah^{\ast},
\end{equation}$$(4)for α men, and
$$\begin{equation}
\bar{U}_{\beta}^{\ast}(h^{\ast})=\ln Ah^{\ast},
\end{equation}$$(4)for α men, and $$\begin{equation}
\bar{U}_{\alpha}^{\ast}(h^{\ast})=\ln Ah^{\ast}+\frac{\gamma n\psi}{ p_{\alpha}}\left( E\ln h-\ln \underline{h}(\alpha) +1\right),
\end{equation}$$(5)for women.
$$\begin{equation}
\bar{U}_{\alpha}^{\ast}(h^{\ast})=\ln Ah^{\ast}+\frac{\gamma n\psi}{ p_{\alpha}}\left( E\ln h-\ln \underline{h}(\alpha) +1\right),
\end{equation}$$(5)for women. $$\begin{equation}
\bar{U}_{\alpha}(h)=(1+\gamma n\psi) \ln Ah+\pi _{\alpha},
\end{equation}$$
$$\begin{equation}
\bar{U}_{\alpha}(h)=(1+\gamma n\psi) \ln Ah+\pi _{\alpha},
\end{equation}$$Furthermore, if
(6)then $$\begin{equation}
\ln \underline{h}(\alpha) \le \psi -p_{\alpha}\ln \frac{\alpha}{\beta}.
\end{equation}$$
$$\begin{equation}
\ln \underline{h}(\alpha) \le \psi -p_{\alpha}\ln \frac{\alpha}{\beta}.
\end{equation}$$(v) The distribution of h remains bounded from below by
 $\underline{h}(\alpha) $in all periods.
$\underline{h}(\alpha) $in all periods.
Because men do not observe who their children are, they do not invest in them and consume all their endowment. Furthermore, in this regime where children deliver positive net utility, men accept all sexual intercourse. Consequently, women can select which men they mate with. Since men do not provide resources to their children, the man’s human capital is irrelevant to the woman’s choice. Therefore, women will choose men on the basis of their genetic characteristics only, and only mate with alphas.
The State of Nature closely matches the world described by Trivers’s (Reference Trivers and Campbell1972) seminal paper: males do not invest in their offspring and there is wide dispersion in their reproductive success: alpha men potentially have many mates while beta ones are excluded from reproduction.
2.3. Marriage
I now introduce marriage into the model. Marriage is a contract by which a woman commits to have intercourse with only one man-her husbandFootnote 24. A married man knows his children are his, a property I will call legitimacy. Legitimacy makes it desirable for the man to invest in the children’s human capital. Women benefit from marriage because there is a surplus from the match, due to children’s human capital being a public good to the household. That surplus makes it possible for the man to transfer income to both his wife and his children, while remaining better-off than if he were single.
I assume commitment in marriage is perfectly enforcible. Obviously that is a simplification, given that cuckoldry and illegitimacy are not rare. Imperfect commitment could be embodied into the model by assuming that women married with beta men could cheat with alpha men, with some probability of getting caught and some penalty. To the extent that marriage still raises the probability for a man of being able to invest his own children, the qualitative properties of the model should remain, although the return to marriage should be lower than in the full enforcement case.
It is costless for a woman to marry an alpha man, since she gets the same genetic material than if she were promiscuous. By marrying, an alpha man would lose mating opportunities. But, since he would not invest in any illegitimate offspring, and since the woman knows her children are hers, imposing faithfulness to the alpha man is inefficient for the couple. Instead, it is optimal to have a double standard by which the man can be promiscuous outside of the couple, while the woman cannotFootnote 25. If one assumes a double standard, marrying is also costless for alpha men. Therefore, all marriage by alpha men are efficient, and for the sake of concision I will ignore them in the analysis: In what follows the man is beta unless otherwise specified.
If the husband is beta, the woman gets a lower genetic material than if she were single and promiscuous. She needs to be compensated for that loss by the man transferring enough resources to her. As we shall see, that is possible only if the man has enough human capital both in the absolute and relative to the woman. Thus a marriage between a woman and a beta man yields a positive net surplus only if the man’s human capital is high enough and if the woman’s human capital is low enough.Footnote 26
I will assume, as in e.g. Browning and Chiappori (1998), that the joint allocation of resources within a household is efficient. Then
Proposition 2
Let
In any household such that ![]() $h+h^{\ast} \ge \underline{h}(\beta),$
$h+h^{\ast} \ge \underline{h}(\beta),$
(i) The couple has n intercourses, producing n children
(ii) The investment level in children is
(8) $$\begin{equation}
z=\frac{\gamma \psi}{1+\gamma n\psi}A(h+h^{\ast}),
\end{equation}$$
$$\begin{equation}
z=\frac{\gamma \psi}{1+\gamma n\psi}A(h+h^{\ast}),
\end{equation}$$(iii) There exists θ ∈ [0, 1] such that the wife’s and husband’s consumption level respectively are
(9) $$\begin{eqnarray}
c =\frac{\theta}{1+\gamma n\psi}A(h+h^{\ast});
\end{eqnarray}$$(10)
$$\begin{eqnarray}
c =\frac{\theta}{1+\gamma n\psi}A(h+h^{\ast});
\end{eqnarray}$$(10) $$\begin{eqnarray}
c^{\ast} =\frac{1-\theta}{1+\gamma n\psi}A(h+h^{\ast}).
\end{eqnarray}$$
$$\begin{eqnarray}
c^{\ast} =\frac{1-\theta}{1+\gamma n\psi}A(h+h^{\ast}).
\end{eqnarray}$$(iv) The wife’s and husband’s utility are
(11) $$\begin{equation}
U_{\beta}(h,h^{\ast},\theta) =\ln \theta +(1+\gamma n\psi) ( \ln A+\ln (h+h^{\ast})) +\pi _{\beta},
\end{equation}$$(12)
$$\begin{equation}
U_{\beta}(h,h^{\ast},\theta) =\ln \theta +(1+\gamma n\psi) ( \ln A+\ln (h+h^{\ast})) +\pi _{\beta},
\end{equation}$$(12) $$\begin{equation}
U_{\beta}^{\ast}(h,h^{\ast},\theta) =\ln (1-\theta) +(1+\gamma n\psi) ( \ln A+\ln (h+h^{\ast})) +\pi _{\beta}.
\end{equation}$$
$$\begin{equation}
U_{\beta}^{\ast}(h,h^{\ast},\theta) =\ln (1-\theta) +(1+\gamma n\psi) ( \ln A+\ln (h+h^{\ast})) +\pi _{\beta}.
\end{equation}$$
Let us now analyze when marriage is beneficial relative to being single. A marriage is efficient provided there exists some sharing parameter θ such that each party can get a utility greater than their outside option. These outside options are given by the beta man’s and the woman’s utilities in the State of Nature (since one can always mate with an alpha men, as they accept all intercourse). The following result then holds:
Proposition 3
Let
Let
A marriage is efficient relative to both parties remaining single, if and only if the marriage viability condition holds:
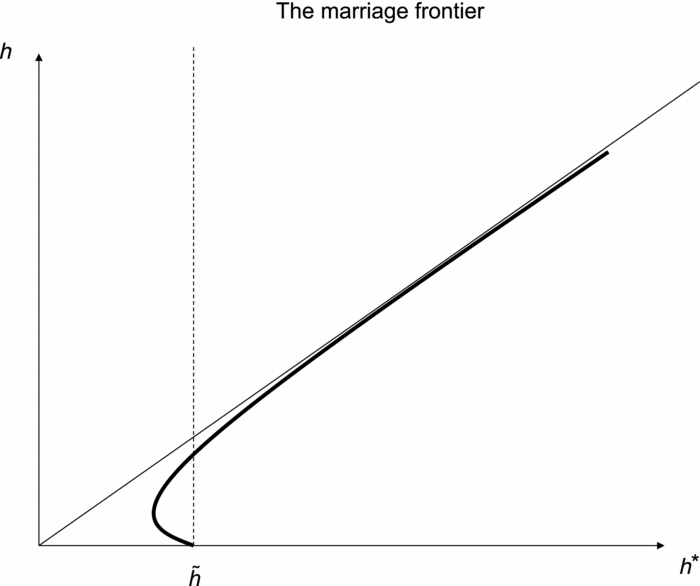
Figure 3 depicts the marriage viability set in the (h*, h) plane. Given h, there exists a minimum value of h* such that the match is viable. Furthermore, that value is increasing with h. Therefore, there is hypergamy in that the match is more viable, the more skilled the man relative to the woman.

Figure 3. The marriage frontier.
A woman with zero human capital is marriageable because an arbitrarily small consumption level is enough to compensate her for the opportunity cost of not mating with an alpha male, while her husband gets a finite benefit from legitimacy. As her human capital goes up, the consumption equivalent of foregone mating opportunities with alpha men goes up, and only men with a high enough level of human capital are willing to transfer that amount to her in exchange for legitimacy.Footnote 27
3. WHO MARRIES WHOM? MARRIAGE MARKETS
I now derive and discuss the equilibrium assignment for a given generation, and therefore a given distribution of human capital, denoted by f(). I assume that f() has as a bounded support ∈ [h min, h max]. I assume there exists a perfectly competitive marriage market. For simplicity, I also assume that alpha individuals and beta individuals cannot marry each other: there is a separate marriage market for each typeFootnote 28. However, single beta women can still mate with alpha men outside marriage. Their outside option of remaining single is therefore still given by (5). Finally, I assume that
implying, since ![]() $\underline{h}(\beta) \ge \underline{h}(\alpha),$ that all women will mate n times, including single ones.
$\underline{h}(\beta) \ge \underline{h}(\alpha),$ that all women will mate n times, including single ones.
3.1. Defining an Equilibrium
The following definition clarifies the candidate equilibria. Note that it rules out equilibria where some individuals get married and other identical ones do not, except over a set of measure zero.
Definition 1Let f() be the distribution of human capital among the beta individuals, which is assumed to be the same between men and women. Let [h min, h max] be the support of f(). An assignment is
(i) A pair of sets S, S*⊆[h min, h max].
(ii) A mapping Footnote 29h*() from S to S*
such that for any measurable set Σ⊆S
(14) $$\begin{equation}
\int _{\Sigma}f(h)dh=\int _{h^{\ast}(\Sigma) }f(h^{\ast})dh^{\ast}.
\end{equation}$$
$$\begin{equation}
\int _{\Sigma}f(h)dh=\int _{h^{\ast}(\Sigma) }f(h^{\ast})dh^{\ast}.
\end{equation}$$
The sets S, S* tell us the set of women and men, respectively, who are married. The mapping h*() tells us who marries whom. Condition (14) ensures that each woman marries exactly one man, so that for any set of women Σ the measure of the set of their husbands is equal to the measure of Σ.
Definition 2An equilibrium is a quadruple (S, S*, h*(), θ()) such that (S, S*, h*()) is an assignment and θ is a function: S → [0, 1] such that:
(i) ∀h ∈ S,
(15) $$\begin{equation}
U_{\beta}(h,h^{\ast}(h),\theta (h))\ge \bar{U}_{\alpha}(h).
\end{equation}$$
$$\begin{equation}
U_{\beta}(h,h^{\ast}(h),\theta (h))\ge \bar{U}_{\alpha}(h).
\end{equation}$$(ii) ∀h ∈ S,
(16) $$\begin{equation}
U_{\beta}^{\ast}(h,h^{\ast}(h),\theta (h))\ge \bar{U}_{\beta}^{\ast}(h^{\ast}(h)).\end{equation}$$
$$\begin{equation}
U_{\beta}^{\ast}(h,h^{\ast}(h),\theta (h))\ge \bar{U}_{\beta}^{\ast}(h^{\ast}(h)).\end{equation}$$(iii) Let
 $V^{\ast}(h^{\ast})=\bar{U}_{\beta}^{\ast}(h^{\ast})$if h*∉S* and V*(h*) = U*β(h*−1(h*), h*, θ(h*−1(h*))) if h* ∈ S*. Let
$V^{\ast}(h^{\ast})=\bar{U}_{\beta}^{\ast}(h^{\ast})$if h*∉S* and V*(h*) = U*β(h*−1(h*), h*, θ(h*−1(h*))) if h* ∈ S*. Let  $V(h)= \bar{U}_{\alpha}(h)$if h∉S and V(h) = U β(h, h*(h), θ(h)) if h ∈ S.
$V(h)= \bar{U}_{\alpha}(h)$if h∉S and V(h) = U β(h, h*(h), θ(h)) if h ∈ S.For any h, h* ∈ [h min, h max], let
 $\hat{\theta}(h,h^{\ast})$be such that
$\hat{\theta}(h,h^{\ast})$be such that  $U_{\beta}(h,h^{\ast}, \hat{\theta}^{\ast}(h,h^{\ast}))=V(h).$Then the following must be true: (17)
$U_{\beta}(h,h^{\ast}, \hat{\theta}^{\ast}(h,h^{\ast}))=V(h).$Then the following must be true: (17) $$\begin{equation}
\forall h,h^{\ast}\in [h_{\min},h_{\max}],U_{\beta}^{\ast}(h,h^{\ast},\hat{\theta}(h,h^{\ast}))\le V^{\ast}(h^{\ast}).
\end{equation}$$
$$\begin{equation}
\forall h,h^{\ast}\in [h_{\min},h_{\max}],U_{\beta}^{\ast}(h,h^{\ast},\hat{\theta}(h,h^{\ast}))\le V^{\ast}(h^{\ast}).
\end{equation}$$
This definition spells out the three conditions for the equilibrium assignment to be better than any deviation. Condition (i) states that married women get a higher utility than if they were single. Condition (ii) states that married men get a higher utility than if they were single. Condition (iii) that no new couple can be formed so that one party gets at least his/her reservation utility and the other gets strictly more than his/her reservation utility. The function θ() defines the equilibrium consumption share of women. This set of prices clears the market in the sense that no married individual can be better-off by being single instead (conditions (i) and (ii)) or making an acceptable offer to another mate (condition (iii)).Footnote 30, Footnote 31
3.2. Properties of an Equilibrium
We now turn to analyzing the properties of the equilibrium assignment. A natural question to be asked is: will there be sorting? Intuitively, individuals with more human capital may be willing to pay more to get a higher quality mate. This is actually true here:
Proposition 4Any equilibrium assignment function h*(h) must be nondecreasing.
This result, which in some way is a corollary of Lam (Reference Lam1988), comes from the public good aspect of children’s human capital in the household. Since marriage provides benefits in the form of the children’s human capital, it is an increasing returns technology: when the average human capital of a couple doubles, its output, in consumption-equivalent terms, more than doubles; not only can the consumption of each member double, but the quality of the children also goes up. For this reason, people with high human capital are willing to pay more to increase their spouse’s human capital than people with low human capital; the usual sorting conditions hold.
In what follows, we will be able to elicit two types of equilibria, which we now define precisely.
Definition 3An assignment is “Victorian” if S = S* = [h min, h max].
Definition 4An assignment has the “Sex and the City” (SATC) property if there exists ![]() $\bar{h} \le h_{\max}$and
$\bar{h} \le h_{\max}$and ![]() $\underline{h} ^{\ast} \ge h_{\min}$such that
$\underline{h} ^{\ast} \ge h_{\min}$such that ![]() $S=[h_{\min},\bar{h}]$and
$S=[h_{\min},\bar{h}]$and ![]() $S^{\ast}=[\underline{h}^{\ast},h_{\max}].$
$S^{\ast}=[\underline{h}^{\ast},h_{\max}].$
A Victorian assignment is an assignment where everybody marries. Because h() is nondecreasing, women must then marry men with the same rank in the distribution of income. Given that men and women have the same initial distribution of human capital, a Victorian assignment is homogamous, i.e. h*(h) = h.Footnote 32
An “SATC” assignment is such that any single woman has more human capital than any married woman, while the reverse holds for men. Because h() is monotonous, an SATC assignment is hypergamous. Women must marry men who have a higher rank than them in the distribution of income: h*(h) ⩾ h.Footnote 33
The following proposition shows that Victorian and SATC are the only two possible equilibrium types, provided F() has full support.
Proposition 5Assume F() has full support. Then the equilibrium assignment must be either Victorian or SATC.
Proposition 5 implies that in any equilibrium, the singles must be found at the top of the skilled distribution for women and at the bottom for the men. If a man is better-off married than single, then all men with greater skills could also be better-off than single by marrying his wife and give her her reservation utility, which is associated with a smaller share of the now larger surplus than what she got with her original husband. This implies that ![]() $S^{\ast}=[\underline{h}^{\ast},h_{\max}]$ for some
$S^{\ast}=[\underline{h}^{\ast},h_{\max}]$ for some ![]() $\underline{h} ^{\ast}.$ Furthermore, one can show that S must be an interval: single women who are richer than some married women and poorer than some other married ones can successfully underbid one of these two. Finally, the poorest married woman (
$\underline{h} ^{\ast}.$ Furthermore, one can show that S must be an interval: single women who are richer than some married women and poorer than some other married ones can successfully underbid one of these two. Finally, the poorest married woman (![]() $\underline{h})$ must marry the poorest married man (
$\underline{h})$ must marry the poorest married man (![]() $ \underline{h}^{\ast})$. But if both of them are richer than h min, competition from poorer single men and women must drive the surplus of their match to zero–leaving them just as well off as if they were single. But this is not sufficient since, by the hypergamy property, a marriage between
$ \underline{h}^{\ast})$. But if both of them are richer than h min, competition from poorer single men and women must drive the surplus of their match to zero–leaving them just as well off as if they were single. But this is not sufficient since, by the hypergamy property, a marriage between ![]() $ \underline{h}^{\ast}$ and
$ \underline{h}^{\ast}$ and ![]() $h \le \underline{h}$ would then generate a strictly positive surplus and therefore successfully break the original marriage. Therefore, there cannot be any single woman poorer than the poorest married one.
$h \le \underline{h}$ would then generate a strictly positive surplus and therefore successfully break the original marriage. Therefore, there cannot be any single woman poorer than the poorest married one.
3.3. Existence of Victorian Equilibria
Having established results regarding how any equilibrium looks like, we are now able to construct equilibria. In this section, I characterize the existence conditions for Victorian equilibria.
Proposition 6
A. A Victorian equilibrium exists if and only if
(18) $$\begin{equation}
(e^{k}-2^{\gamma n\psi})h_{\max}^{1+\gamma n\psi}\le 2^{\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min},
\end{equation}$$
$$\begin{equation}
(e^{k}-2^{\gamma n\psi})h_{\max}^{1+\gamma n\psi}\le 2^{\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min},
\end{equation}$$B. The corresponding assignment such that
(i) S = S* = [h min, h max].
(ii) h*(h) = h.
(iii)
(19)where λ is any number such that $$\begin{equation}
\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),
\end{equation}$$(20)
$$\begin{equation}
\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),
\end{equation}$$(20) $$\begin{eqnarray}
&&\max \left(( 2^{-\gamma n\psi}e^{k}-1) h_{\max}^{1+\gamma n\psi}, ( 2^{-\gamma n\psi}e^{k}-1) h_{\min}^{1+\gamma n\psi}\right) \nonumber\\
&&\quad\le \lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.
\end{eqnarray}$$
$$\begin{eqnarray}
&&\max \left(( 2^{-\gamma n\psi}e^{k}-1) h_{\max}^{1+\gamma n\psi}, ( 2^{-\gamma n\psi}e^{k}-1) h_{\min}^{1+\gamma n\psi}\right) \nonumber\\
&&\quad\le \lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.
\end{eqnarray}$$
Because there are no singles, the sharing rule within the household is undetermined: the location of the θ(h) profile depends on an undetermined parameter λ. Sharing rules with a higher value of λ are more favorable for women. Also, it can be shown that the interval of acceptable values of λ favors women since it is centered around a strictly positive valueFootnote 34.
3.4. Existence of a “Sex and the City” Equilibrium
If (18) is violated, can we construct an SATC equilibrium? While I cannot prove existence of a marriage market equilibrium for any set of parameters (and I conjecture that for some parameters existence will fail), one can construct an SATC equilibrium if (18) is not violated by too much. This is what the next proposition says:
Proposition 7Assume there exists B ⩾ 0 such that if
then if B is small enough,
(i) An SATC equilibrium exists such that
 $S=[h_{\min},\bar{h}]$and S* = [
$S=[h_{\min},\bar{h}]$and S* = [ $\underline h$*, h max].
$\underline h$*, h max].(ii) In this equilibrium, the assignment function is
(22)implying h*(h min) = $$\begin{equation}
h^{\ast}(h)=F^{-1}(F(h)+F(\underline{h}^{\ast})),
\end{equation}$$
$$\begin{equation}
h^{\ast}(h)=F^{-1}(F(h)+F(\underline{h}^{\ast})),
\end{equation}$$ $\underline h$*. Furthermore,
$\underline h$*. Furthermore,  $\bar{h}=F^{-1}(1-F($
$\bar{h}=F^{-1}(1-F($ $\underline h$*)), implying
$\underline h$*)), implying  $h^{\ast}(\bar{h})=h_{\max}.$
$h^{\ast}(\bar{h})=h_{\max}.$(iii) The married woman’s share in bargaining satisfies
(23)where μ is a constant. $$\begin{equation}
\theta (h)=\left( h+h^{\ast}(h)\right) ^{-(1+\gamma n\psi) }\left[ (1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \right],
\end{equation}$$
$$\begin{equation}
\theta (h)=\left( h+h^{\ast}(h)\right) ^{-(1+\gamma n\psi) }\left[ (1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \right],
\end{equation}$$(iv) The equilibrium is locally unique.
Proof – See Appendix
Thus, singleness arises at the top of the skill distribution for women, and at the bottom for men, as an outcome of competition in marriage markets. This, despite that the sex ratio is 1:1 and that all homogamous marriages might be preferred to being single.Footnote 35
To illustrate these results, consider the following simple numerical example: I assume a = 2ψ, b = ψ, n = 1, γ = 1/ψ, A = 2, implying ![]() $ k=1,\tilde{h}=2/e,\pi _{\beta}=1-2\ln 2,$ and
$ k=1,\tilde{h}=2/e,\pi _{\beta}=1-2\ln 2,$ and ![]() $\underline{h}(\beta) =1.$ I assume that h is uniformly distributed over [h min = 1, h max].
$\underline{h}(\beta) =1.$ I assume that h is uniformly distributed over [h min = 1, h max].
It can be checked that the condition for the Victorian equilibrium to prevail is ![]() $h_{\max} \le \sqrt{\frac{2(1-1/e)}{e-2}}\approx 1.326.$ The minimum value of λ is then (e/2 − 1)h 2max, which varies between 0.359 and 1 − 1/e = 0.632 as h max varies from 1 to 1.326. The maximum value of λ is equal to 0.632 regardless of h max. If λ is equal to its minimum, then the consumption share of the least skilled women is equal to
$h_{\max} \le \sqrt{\frac{2(1-1/e)}{e-2}}\approx 1.326.$ The minimum value of λ is then (e/2 − 1)h 2max, which varies between 0.359 and 1 − 1/e = 0.632 as h max varies from 1 to 1.326. The maximum value of λ is equal to 0.632 regardless of h max. If λ is equal to its minimum, then the consumption share of the least skilled women is equal to ![]() $\frac{1}{2}(1+(e/2-1)h_{\max}^{2}),$ which varies between 0.68 and 0.816 as h max varies from 1 to 1.326. The consumption share of the most skilled women is equal to e/4 = 0.68, independently of h max. If λ is equal to its maximum, the consumption share of the least skilled women is equal to 0.816, independently of h max. The consumption share of the most skilled women is equal to
$\frac{1}{2}(1+(e/2-1)h_{\max}^{2}),$ which varies between 0.68 and 0.816 as h max varies from 1 to 1.326. The consumption share of the most skilled women is equal to e/4 = 0.68, independently of h max. If λ is equal to its maximum, the consumption share of the least skilled women is equal to 0.816, independently of h max. The consumption share of the most skilled women is equal to ![]() $\frac{1}{2}(1+(1-1/e)h_{\max}^{-2}),$ which varies from 0.816 to 0.68 as h max varies from 1 to 1.326.
$\frac{1}{2}(1+(1-1/e)h_{\max}^{-2}),$ which varies from 0.816 to 0.68 as h max varies from 1 to 1.326.
For h max ⩾ 1.326, we can construct SATC equilibria – checking that all the sufficient conditions spelled out in the proof of Proposition 7 hold – as long as h max remains smaller than 3.89. Thus, the range where the SATC equilibrium exists is wide; h max can be well above the maximum value for which a Victorian equilibrium prevails. Table 2 reports the bounds of the set of married men and women, the constant μ, the consumption share of the most and least skilled married women, and the fraction of unmarried people, for increasing values of h max, starting from the critical level of 1.326.
Table 2. Characteristics of the SATC equilibrium, for various values of h max

3.5. Discussion
3.5.1
How is output being shared? In both the SATC and the Victorian equilibrium, θ() satisfies the following differential equation:
This equation tells us that locally, women with an arbitrarily close level of human capital cannot profitably underbid a married woman for her husband (a similar condition for men leads to the same mathematical expression). The second term in parentheses, h*′(h), tells us that the larger the husband’s human capital relative to the husbands of marginally less skilled women, the lower the share of output that this woman can get, due to competition from these women. The first term tells us that the larger a woman’s output share, the smaller (more negative) θ′(h). This is because the greater her output share, the greater the incentives for marginally less skilled women to underbid her; for them to be deterred from doing that, their own output share must be higher, hence θ′(h) must be lower.
3.5.2. The role of inequality
A key property of (18) is that it is more likely to be satisfied, the greater h min and the lower h max. Therefore, greater inequality, as defined by a larger h max and/or a lower h min makes it more likely that the equilibrium, if any, be of the SATC type. In other words, inequality destroys the Victorian equilibrium and therefore has an adverse effect on the number of marriages.
Let us try to provide some intuition for this result. The mechanism at work is an unraveling of marriage market competition throughout the distribution of income. If ek ⩽ 2γnψ, (18) always holds and the Victorian equilibrium always exists, regardless of h min and h max. If ek ⩾ 2γnψ, then Proposition 6 implies that λ ⩾ 0, so that (i) women get more than 50% of the marriage’s total consumption, and (ii) this share is lower, the greater the woman’s human capital. Women get a large share of the surplus because k is large, implying that the value of the lost genetic material from mating with a beta man instead of an alpha man is large. But, as seen in Section 3.5.1, this large share of the surplus has an effect on competition between married beta people: as implied by (24), the woman’s output share must be more steeply decreasing with h. Hence, if ek ⩾ 2γnψ, competition tends to reduce the share of high-skill women and to increase that of low-skill women. But, if there is too much inequality, this process will be defeated by the exit options of low-skill men who will be better-off single than transferring a large share of the surplus to their wives. And similarly, high-skill women will get too low a share of the surplus for them to get appropriate compensation for mating with a beta man. This destroys the Victorian equilibrium and triggers a transition to an SATC equilibrium.
3.5.3. The returns to human capital
While the model has no role for the returns to human capital, since people cannot change the level of h inherited from their parents, it is instructive to compute them among alternative arrangements. To do so, I compare the marginal utility of human capital for men and women at a given level of human capital for different institutions. In addition to any difference in monetary returns, there are differences between men and women in the returns to human capital expressed in terms of utility, to the extent that if men are single they do not expect to have to invest in their offsprings’ human capital, while this is not true for single women. This may help explain the recent educational gap in favor of women despite that the wage gap continues to favor men, in light of the increased prevalence of divorce rates and marital instability.Footnote 36
State of Nature. In the state of nature, women have a greater return to human capital than men:
 $$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}^{\ast}(h^{\ast})}{dh^{\ast}} &=&\frac{d\bar{U} _{\alpha}^{\ast}(h^{\ast})}{dh^{\ast}}=\frac{1}{h^{\ast}};\\
\frac{d\bar{U}(h)}{dh} &=&\frac{1+\gamma n\psi}{h}.
\end{eqnarray*}$$
$$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}^{\ast}(h^{\ast})}{dh^{\ast}} &=&\frac{d\bar{U} _{\alpha}^{\ast}(h^{\ast})}{dh^{\ast}}=\frac{1}{h^{\ast}};\\
\frac{d\bar{U}(h)}{dh} &=&\frac{1+\gamma n\psi}{h}.
\end{eqnarray*}$$This is because women invest their resources in both consumption and children, while men spend all on consumption. This suggest that if there were scope for accumulating human capital beyond what is inherited from parents, then in the state of nature women would acquire more human capital than men.
Victorian equilibrium. Let us now compute the rate of return to h in the Victorian equilibrium. For beta women, it is equal to
 $$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}}{dh} &=&\frac{\theta ^{\prime}(h)}{\theta (h)}+ \frac{1+\gamma n\psi}{h} \\
&=&\frac{(1+\gamma n\psi) }{h+\lambda h^{-\gamma n\psi}}.
\end{eqnarray*}$$
$$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}}{dh} &=&\frac{\theta ^{\prime}(h)}{\theta (h)}+ \frac{1+\gamma n\psi}{h} \\
&=&\frac{(1+\gamma n\psi) }{h+\lambda h^{-\gamma n\psi}}.
\end{eqnarray*}$$Similarly, for men we get
 $$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}^{\ast}}{dh^{\ast}} &=&\frac{-\theta ^{\prime}(h)}{ 1-\theta (h)}+\frac{1+\gamma n\psi}{h} \\
&=&\frac{(1+\gamma n\psi) }{h-\lambda h^{-\gamma n\psi}}.
\end{eqnarray*}$$
$$\begin{eqnarray*}
\frac{d\bar{U}_{\beta}^{\ast}}{dh^{\ast}} &=&\frac{-\theta ^{\prime}(h)}{ 1-\theta (h)}+\frac{1+\gamma n\psi}{h} \\
&=&\frac{(1+\gamma n\psi) }{h-\lambda h^{-\gamma n\psi}}.
\end{eqnarray*}$$If λ ⩾ 0, i.e. θ(h) ⩾ 1/2, then men have a greater return to human capital than women. This is because by acquiring more human capital they end up marrying a woman with a smaller equilibrium share of output. The converse occurs for women. If λ ⩽ 0, i.e. if k is not too large, then the reverse holds: women have a greater return to human capital because in equilibrium their husband’s output share falls as they climb the social ladder.
Thus in a Victorian equilibrium the only factor that may introduce a gap between men and women in terms of their incentives to acquire human capital is the indeterminate parameter λ which determines the sharing rule. If the economy coordinates on an equilibrium such that λ ⩾ 0, then women have a greater share of the surplus than men but this fuels competition for richer men, thus reducing the woman’s share when her human capital goes up. This in turn reduces the returns to human capital for women. The converse occurs for λ ⩽ 0.
SATC equilibrium. The analysis is richer in the case of an SATC equilibrium. For married women, the returns to human capital come from three components:
• The effect of their own human capital on the quality of their mate, which is equal to
 $$\begin{equation*}
\frac{\partial U_{\beta}}{\partial h^{\ast}}h^{\ast \prime}(h)=\frac{ (1+\gamma n\psi) h^{\ast \prime}(h)}{h+h^{\ast}(h)}.
\end{equation*}$$
$$\begin{equation*}
\frac{\partial U_{\beta}}{\partial h^{\ast}}h^{\ast \prime}(h)=\frac{ (1+\gamma n\psi) h^{\ast \prime}(h)}{h+h^{\ast}(h)}.
\end{equation*}$$The larger h*′(h), the greater the increase in the husband’s human capital when the wife’s human capital goes up by one unit. Since
 $h^{\ast \prime}(h)=\frac{f(h)}{f(h^{\ast})}$ by virtue of (22), this effect is stronger, the scarcer men are relative to women locally.
$h^{\ast \prime}(h)=\frac{f(h)}{f(h^{\ast})}$ by virtue of (22), this effect is stronger, the scarcer men are relative to women locally.• The effect of their human capital on their output share, given by
 $$\begin{equation*}
\frac{\partial U_{\beta}}{\partial \theta}\theta ^{\prime}(h)=\frac{ \theta ^{\prime}(h)}{\theta (h)}.
\end{equation*}$$
$$\begin{equation*}
\frac{\partial U_{\beta}}{\partial \theta}\theta ^{\prime}(h)=\frac{ \theta ^{\prime}(h)}{\theta (h)}.
\end{equation*}$$The return to human capital is greater, the greater the increment in the woman’s output share when she climbs the social ladder. As implied by (24), this effect can be further decomposed into the effect of husband’s incremental human capital and the effect of marriage competition. Straightforward computations show that the former exactly cancels the
 $\frac{ \partial U_{\beta}}{\partial h^{\ast}}h^{\ast \prime}(h)$ term, so that h*′(h) disappears from the final formula.
$\frac{ \partial U_{\beta}}{\partial h^{\ast}}h^{\ast \prime}(h)$ term, so that h*′(h) disappears from the final formula.• Finally, there is a direct effect due to the decreasing marginal utility of human capital; this effect is equal to
 $$\begin{equation*}
\frac{\partial U_{\beta}}{\partial h}=\frac{1+n\gamma \psi}{h+h^{\ast}(h)}.
\end{equation*}$$
$$\begin{equation*}
\frac{\partial U_{\beta}}{\partial h}=\frac{1+n\gamma \psi}{h+h^{\ast}(h)}.
\end{equation*}$$
Similar effects hold for men. Putting all these effects together, we get the net return to human capital for women:
For men, the corresponding formula is
One effect tends to generate greater return to human capital for men than for women with the same skills: the former’s mate has less skills than the latter’s; because of hypergamy, total household human capital is smaller for men than for women with the same skills, hence the greater returns to skills for the former. This effect was not present in the homogamous Victorian equilibrium. The other effect is that of θ, which is the same as in the Victorian equilibrium. If θ() ⩾ 1/2 then women will have a lower return to human capital because of the net effect of marriage competition. While we do not know in general whether this inequality holds, it does for the equilibria constructed in Proposition 6, because they are close to the limit Victorian equilibrium such that (18) holds exactly, and we know from Proposition 6 that λ ⩾ 0, i.e. θ() ⩾ 1/2, for these equilibria.
3.6. Comparative Statics with Respect to the Assignment
In this section, I provide further results on the comparative statics of the SATC assignment constructed in Proposition 7. The following result can be proved:
Proposition 8Under the conditions of Proposition 7, the following comparative statics result hold:
(i)
 $\displaystyle\frac{d\underline{h}^{\ast}}{dk} \ge 0;\frac{d\mu}{dk} \ge 0$
$\displaystyle\frac{d\underline{h}^{\ast}}{dk} \ge 0;\frac{d\mu}{dk} \ge 0$(ii)
 $\displaystyle\frac{d\underline{h}^{\ast}}{d\tilde{h}} \ge 0;\frac{d\mu}{d \tilde{h}} \le 0$
$\displaystyle\frac{d\underline{h}^{\ast}}{d\tilde{h}} \ge 0;\frac{d\mu}{d \tilde{h}} \le 0$(iii) For a uniform distribution F(),
 $\displaystyle\frac{d \underline{h}^{\ast}}{dh_{\max}} \ge 0,\displaystyle\frac{d\mu}{dh_{\max}} \ge 0$and the proportion of married people falls with h max.
$\displaystyle\frac{d \underline{h}^{\ast}}{dh_{\max}} \ge 0,\displaystyle\frac{d\mu}{dh_{\max}} \ge 0$and the proportion of married people falls with h max.
This result tells us that the proportion of married people will fall, and the equilibrium gap in human capital between husbands and wives rise,
– if k goes up, that is the opportunity cost of mating with a beta instead of an alpha goes up. This is natural, as an increase in k raises the outside option of celibacy for the most skilled women. For the same reason, women get a higher share of output, i.e. μ goes up.
– if
 $\tilde{h}$ goes up, holding other parameters constant. The parameter
$\tilde{h}$ goes up, holding other parameters constant. The parameter  $\tilde{h}$ is inversely related to the hedonic value of having children with a beta father, relative to not having children. The greater
$\tilde{h}$ is inversely related to the hedonic value of having children with a beta father, relative to not having children. The greater  $\tilde{h},$ the lower the value of children. When
$\tilde{h},$ the lower the value of children. When  $\tilde{h}$ goes up, the least skilled married men find themselves better-off being single, as their willingness to pay for children is lower. As a result, too, the women’s share falls, but they get assigned to richer husbands.
$\tilde{h}$ goes up, the least skilled married men find themselves better-off being single, as their willingness to pay for children is lower. As a result, too, the women’s share falls, but they get assigned to richer husbands.– if there is an increase in inequality due to a higher maximum level of human capital, in the case of a uniform distribution. This reduces the proportion of women below
 $\bar{h},$ but increases the proportion of men above
$\bar{h},$ but increases the proportion of men above  $\underline{h}^{\ast}.$ This creates an imbalance in the marriage market, which leads to an increase in the women’s share of output as well as an increase in
$\underline{h}^{\ast}.$ This creates an imbalance in the marriage market, which leads to an increase in the women’s share of output as well as an increase in  $\underline{h}^{\ast}.$ The total effect on the number of marriages is negative.
$\underline{h}^{\ast}.$ The total effect on the number of marriages is negative.
3.7. Sexual Repression
We have studied the properties of the equilibrium assignment when women can exercise their choice between committing to a monogamous marriage and having children on their own. Traditionally, though, many societies put severe penalities on out-of-wedlock birth, which I will call “sexual repression”. While such penalties are nonbinding if the equilibrium is Victorian, they would prevent an SATC equilibrium from arising.
In this section, I compute the equilibrium when there is sexual repression. I then compare it to an equilibrium without sexual repression: When the latter is of the SATC type, sexual repression reduces the welfare of beta women while increasing that of beta men. Alpha men lose to the extent that their mating opportunities outside marriage disappear, while alpha women are indifferent.
The analysis thus sheds light on the political economy of sexual repression. In a patriarchal society where men have more political power than women and where beta men are more numerous than alpha men, sexual repression is likely to arise. It allows the low-skilled men to marry and procreate, and men with greater skills get higher quality mates. Furthermore, they also get an improvement in their bargaining share as the outside option of single motherhood has disappeared for women. Hence, beta married women lose, and so do single ones.
Proposition 9Assume that no single woman can legally bear children. Then, there exists a Victorian equilibrium assignment such that
(i) S = S* = [h min, h max].
(ii) h*(h) = h.
(iii)
 $\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),$where λ is any number such that (25)
$\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),$where λ is any number such that (25) $$\begin{equation}
-\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}\le \lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.
\end{equation}$$
$$\begin{equation}
-\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}\le \lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.
\end{equation}$$
Proof – See Appendix.
This proposition tells us that under sexual repression a Victorian equilibrium always exists. Furthermore, a comparison of (25) with (20) shows that the upper bound of the admissible range for λ (which is determined by men’s outside option) is the same as for a Victorian equilibrium absent sexual repression. On the other hand, the lower bound here is always negative and lower than absent sexual repression. And it is the opposite of the upper bound. Thus the bias in favor of women is eliminated: the interval for the equilibrium values of λ is now centered around zero. Sexual repression widens the range of equilibrium sharing rules and the additional values of λ are all more favorable to beta men than the ones that could prevail absent sexual repression.
Proposition 10Assume that in the absence of sexual repression there exists a Sex and the City equilibrium. Let VS(h) (resp. V*S(h*)) be the utility of a beta woman (resp. beta man) with human capital h (resp. h*) in that SATC equilibrium. Let VV(h; λ) (resp. V*V(h*; λ)) be the utility of a beta woman (resp. beta man) in a Victorian equilibrium with sexual repression and sharing parameter λ. Then
 $$\begin{eqnarray*}
\forall \lambda &\in &[-\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}], \\
\forall h &\in &[h_{\min},h_{\max}],V_{V}(h;\lambda) \le V_{S}(h),\textit{and} \\
\forall h^{\ast} &\in &[h_{\min},h_{\max}],V_{V}^{\ast}(h^{\ast};\lambda) \ge V_{S}^{\ast}(h^{\ast}).
\end{eqnarray*}$$
$$\begin{eqnarray*}
\forall \lambda &\in &[-\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}], \\
\forall h &\in &[h_{\min},h_{\max}],V_{V}(h;\lambda) \le V_{S}(h),\textit{and} \\
\forall h^{\ast} &\in &[h_{\min},h_{\max}],V_{V}^{\ast}(h^{\ast};\lambda) \ge V_{S}^{\ast}(h^{\ast}).
\end{eqnarray*}$$Proof – See Appendix.
This proposition tells us that even if we compare the sexually repressed Victorian equilibrium which is most favorable to women to the SATC equilibrium, the latter leaves them better-off, while men are better-off in the former.
Intuitively, if one were to start from an SATC equilibrium and introduce sexual repression, highly skilled single women would not be able to have children and it would be profitable for them to underbid the most skilled married women. This process would trickle down throughout the distribution of skills, thus reducing the bargaining share of all women, while at the same time reassigning a husband with lower skills to each married woman. For these reasons all beta women lose, while all beta men gain for symmetrical reasons.
This result sheds light on the observation that patriarchal societies where men have disproportional political power compared to women tend to be associated with sexual repression. Such provisions both ensure that each beta man can get a wife while at the same time eliminating the source of the asymmetry between men and women in bargaining, namely the women’s natural possibility to get their own illegitimate children from a mate they pick. Conversely, the introduction of women’s suffrage in the West during the twentieth century has been followed by a relaxation of regulations and social norms that penalized single parenthood.Footnote 37
4. THE DYNAMICS OF HUMAN CAPITAL ACCUMULATION
We now provide some results about the effect of marriage on the dynamics of human capital accumulation. The results clearly depend on whether mating with an alpha man enhances the children’s human capital as assumed above (model A) or whether the gratification is purely hedonic (model B).
In model A, I show that marriage improves human capital accumulation provided the productivity difference between the two types is not too large. Otherwise, marriage will reduce the level of human capital in the long run despite that all marriages are voluntary and viable. I also show that imposing marriage as a social norm, for example in the form of sexual repression, further reduces the level of human capital accumulation.
In model B, however, marriage has unambiguous positive effects on human capital accumulation, since the mate’s type has no impact on the innate ability of the children, who always get higher parental investment under marriage.
I first analyze the effects of marriage on the children’s human capital for a given couple in partial equilibrium, and then provide some results on aggregate dynamics in general equilibrium.
4.1. Partial Equilibrium Analysis
One key aspect of marriage is that two parents, rather than one, now invest in the children’s human capital. In the state of nature, parental investment is equal to ![]() $z=\frac{\gamma \psi}{1+\gamma n\psi}Ah;$ in the matrimonial society, it is equal to
$z=\frac{\gamma \psi}{1+\gamma n\psi}Ah;$ in the matrimonial society, it is equal to ![]() $z=\frac{\gamma \psi}{1+\gamma n\psi}A(h+h^{\ast}).$ Because the child’s human capital is a public good to his/her parents, establishing a link between fathers and their children works like a free lunch and may make all parties better-off. The woman, however, bears the opportunity cost of not mating with an alpha male. If she did instead of marrying, the resulting expected human capital of children would be equal to
$z=\frac{\gamma \psi}{1+\gamma n\psi}A(h+h^{\ast}).$ Because the child’s human capital is a public good to his/her parents, establishing a link between fathers and their children works like a free lunch and may make all parties better-off. The woman, however, bears the opportunity cost of not mating with an alpha male. If she did instead of marrying, the resulting expected human capital of children would be equal to
This is to be compared with its counterpart if the woman marries a beta man:
We get that marriage improves the children’s human capital, i.e. Elnh′Mβ ⩾ ElnhS′, if and only if
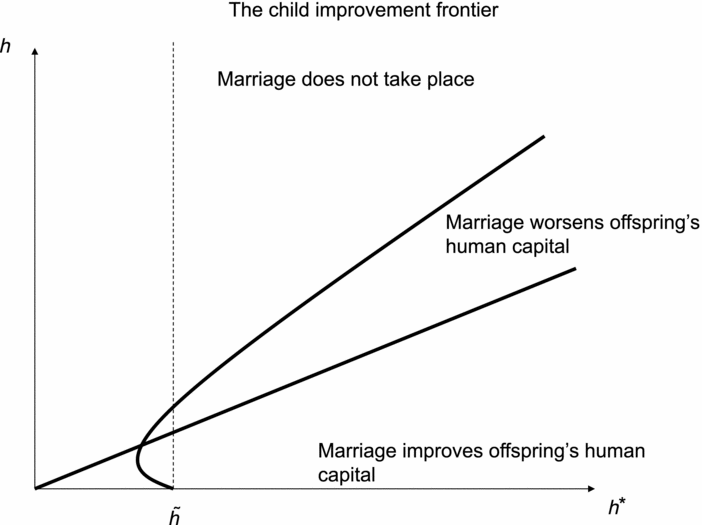
It is easy to see that if ![]() $h \ge \tilde{h},$ this condition is stronger than (13) – See Figure 4. There are marriages that are preferable to being single but yield a lower human capital to the children than if the woman mated with an alpha man instead. The additional investment in children is not enough to compensate for the poorer genetic material. This is possible because the mother gets a higher consumption level due to the “free lunch” aspect of legitimacy discussed above (while the father still gets the direct benefit of legitimacy). Marriage not only boosts investment in children but has consumption benefits as well.
$h \ge \tilde{h},$ this condition is stronger than (13) – See Figure 4. There are marriages that are preferable to being single but yield a lower human capital to the children than if the woman mated with an alpha man instead. The additional investment in children is not enough to compensate for the poorer genetic material. This is possible because the mother gets a higher consumption level due to the “free lunch” aspect of legitimacy discussed above (while the father still gets the direct benefit of legitimacy). Marriage not only boosts investment in children but has consumption benefits as well.

Figure 4. The child improvement frontier.
To summarize: While marriage always boosts investment in children, it does not necessarily boost their human capital in model A, because of the implied reduction in the father’s genetic quality. Human capital goes up if the marriage is sufficiently hypergamous or if the father is alpha.
4.2. The Effect of Marriage on Aggregate Human Capital Accumulation in the Long-Run
I now study the effect of marriage on human capital accumulation in the economy as a whole, comparing human capital accumulation in the State of Nature with a marital economy in a Victorian equilibrium.Footnote 38
4.2.1. Aggregate human capital accumulation in the State of Nature
A first step is to characterize aggregate human capital accumulation in the State of Nature. This is easy, provided average human capital is defined in logarithms. Assume the conditions of Proposition 1 hold. We get from (2):
 $$\begin{eqnarray}
E\ln h^{\prime} &=&a+\psi E\ln z \nonumber \\
&=&\psi (E\ln h-\ln \underline{h}(\alpha) +1).
\end{eqnarray}$$
$$\begin{eqnarray}
E\ln h^{\prime} &=&a+\psi E\ln z \nonumber \\
&=&\psi (E\ln h-\ln \underline{h}(\alpha) +1).
\end{eqnarray}$$ Thus, average log human capital converges to ![]() $\psi (1-\ln \underline{h} (\alpha) )/(1-\psi) .$
$\psi (1-\ln \underline{h} (\alpha) )/(1-\psi) .$
4.2.2. Aggregate human capital accumulation in an economy with marriage
We now turn to the marital economy. An important technical step is to ensure that a marriage market equilibrium exists at all dates. To do so, we construct a Victorian equilibrium by checking that the inherited distribution of skills at each date satisfies the conditions of Proposition 6. As long as that is granted, it is straightforward to characterize the evolution of the economy’s average human capital, as well as its genetic composition, and compare it to the state of nature.
Proposition 11Let
 $$\begin{eqnarray*}
h_{\max,\alpha}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\alpha) )+(1-p_{\alpha})\ln \frac{\alpha}{\beta}}{1-\psi}\right]; \\
h_{\max,\beta}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\beta) )+(\psi -p_{\beta})\ln \frac{\alpha}{\beta}}{1-\psi}\right] \\
h_{\min,\beta}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\beta) )-p_{\beta}\ln \frac{\alpha}{\beta}}{1-\psi}\right].
\end{eqnarray*}$$
$$\begin{eqnarray*}
h_{\max,\alpha}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\alpha) )+(1-p_{\alpha})\ln \frac{\alpha}{\beta}}{1-\psi}\right]; \\
h_{\max,\beta}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\beta) )+(\psi -p_{\beta})\ln \frac{\alpha}{\beta}}{1-\psi}\right] \\
h_{\min,\beta}^{LR} &=&\exp \left[ \frac{\psi (1+\ln 2-\ln \underline{h} (\beta) )-p_{\beta}\ln \frac{\alpha}{\beta}}{1-\psi}\right].
\end{eqnarray*}$$Assume that
Assume that the support of the initial distribution of human capital for alpha (resp. beta) people is contained in [hLR min, β, h max, αLR] (resp. [hLR min, β, h max, βLR]). Assume (18) holds at h max = hLR max, βand h min = hLR min, β. Let ρtbe the proportion of alpha individuals. Then
(i) There exists a path for the economy where the marriage market equilibrium is Victorian for both the alphas and the betas at each date.
(ii) Along this path ρtevolves according to
(29) $$\begin{equation}
\rho _{t+1}=p_{\beta}(1-\rho _{t})+p_{\alpha}\rho _{t}.
\end{equation}$$
$$\begin{equation}
\rho _{t+1}=p_{\beta}(1-\rho _{t})+p_{\alpha}\rho _{t}.
\end{equation}$$(iii) The average log human capital of this economy, defined as Elnht, evolves according to
(30) $$\begin{equation}
E\ln h_{t+1}=\psi [E\ln h_{t}+1+\ln 2-\rho _{t}\ln \underline{h} (\alpha) -(1-\rho _{t})\ln \underline{h}(\beta)].
\end{equation}$$
$$\begin{equation}
E\ln h_{t+1}=\psi [E\ln h_{t}+1+\ln 2-\rho _{t}\ln \underline{h} (\alpha) -(1-\rho _{t})\ln \underline{h}(\beta)].
\end{equation}$$
The following proposition establishes conditions for such a Victorian equilibrium to deliver a higher average level of human capital.
Proposition 12Assume the conditions of Proposition 11 hold. Then
(i) the Victorian equilibrium has a greater offspring expected log human capital than the State of Nature at date t if and only if
 $$\begin{equation*}
\psi \ln 2-(1-\rho _{t})\left[ p_{\alpha}-p_{\beta}\right] \ln \frac{ \alpha}{\beta} \ge 0.
\end{equation*}$$
$$\begin{equation*}
\psi \ln 2-(1-\rho _{t})\left[ p_{\alpha}-p_{\beta}\right] \ln \frac{ \alpha}{\beta} \ge 0.
\end{equation*}$$(ii) The Victorian equilibrium steady state has a greater expected log human capital than the State of Nature steady state if and only if
 $$\begin{equation*}
\psi \ln 2 \ge (p_{\alpha}-p_{\beta})\frac{1-p_{\alpha}}{1-p_{\alpha}+p_{\beta}}\ln \frac{\alpha}{\beta}.
\end{equation*}$$
$$\begin{equation*}
\psi \ln 2 \ge (p_{\alpha}-p_{\beta})\frac{1-p_{\alpha}}{1-p_{\alpha}+p_{\beta}}\ln \frac{\alpha}{\beta}.
\end{equation*}$$(iii) At any given date, the Victorian equilibrium has a greater expected log human capital for the betas than the State of Nature if and only if
(31) $$\begin{equation}
\psi \ln 2 \ge \left[ p_{\alpha}-p_{\beta}\right] \ln \frac{\alpha}{\beta}.
\end{equation}$$
$$\begin{equation}
\psi \ln 2 \ge \left[ p_{\alpha}-p_{\beta}\right] \ln \frac{\alpha}{\beta}.
\end{equation}$$
This is equivalent to (26) for h* = h.
Proposition 12 suggests that marriage boosts society’s aggregate human capital if
(i) The alphas are not too different from the betas in terms of the likelihood of getting an alpha offspring, or
(ii) The alpha’s productivity in accumulating human capital is not too different from the betas’, or
(iii) The proportion of alphas is sufficiently large.Footnote 39
The condition for marriage to increase the human capital of the betas has the same qualitative properties but is more stringent, since one now ignore the alpha marriages that always enhance the offspring’s human capital, since they involve no loss of genetic material.
4.3. Can Sexual Repression Enhance Human Capital?
The penalization of out-of-wedlock birth has been prevalent in most civilizations throughout history. The political economy analysis offered in Section 3.7 may help to explain why that is the case. Another issue is whether sexual repression benefits future generations by improving their average human capital. If so, we might expect societies where it prevails to grow faster and potentially eliminate societies where it does not prevail.
Remember that sexual repression works by forcing a Victorian equilibrium upon a society that would otherwise be in an SATC equilibrium. This has two conflicting effects on the offsprings’ human capital:
– Married women have children with greater parental investment, and therefore more human capital, in the SATC equilibrium. This is because they marry a man with better skills than in the Victorian assignment.
– Unmarried women have lower parental investment but their children are better endowed genetically.
Despite that, we can establish a non-ambiguous result:
Proposition 13Assume an SATC equilibrium exists absent sexual repression. For any woman with human capital h, let ESATClnh′(h) (resp. EVlnh′(h)) be her offspring’s average log human capital in the SATC equilibrium (resp. in the sexually repressed Victorian assignment). Then
Again, this result would be overturned if the value of alpha men were purely hedonic (model B), in which case sexual repression would unambiguously enhance human capital accumulation.
5. SUMMARY AND CONCLUSION
By bringing fathers into the family, marriage allows to increase parental investment in children. But, for this to be credibly operational, monogamy must be enforced. As a result, women lose the opportunity of choosing more attractive mates.
Most of the results derive from this trade-off. Hypergamy arises from the fact that women must be compensated for the utility loss associated with the foregone mating opportunities. Assortative mating arises even though there are no complementarities between the skills of the two members of the couple, due to the public good aspect of children’s human capital, which generates increasing returns to skills in the household.
The institution of marriage reduces the genetic quality of offspring, with that reduction being compensated by greater parental investment. As a result, a marital society does not necessarily imply greater human capital than the State of nature. As in Saint-Paul (Reference Saint-Paul2007), this is an example of institutions increasing the frequency of less fit genes as they provide alternative means of achieving fitness. But this result would be overturned if one holds the view that the mates with the best genes are not more productive but only more sexually attractive, in which case the marital society unambiguously achieves greater human capital.
Another key result is that inequality in skills in some sense intensifies competition in marriage markets and leads to “SATC” equilibria where a pool of single women arises at the top, while a corresponding pool of single men emerges at the bottom of the distribution.
Acknowledgments
I am grateful to Daron Acemoglu, Alison Booth, Maristella Bottcini, Lena Edlund, John Moore, Alwyn Young, the editor, an anonymous referee, and seminar participants at Birkbeck College, Collegio Carlo Alberto, the London School of Economics, Edinburgh University, The NBER Summer Institute, The Italian Labour Economics Association congress (Trieste), University College, CREST, and the Yrjö Jahnsson Award lecture, Helsinki, for helpful comments and suggestions.
APPENDIX A
A.1. Proof of Proposition 1
Conditional on mating, a woman will always prefer an α, since she gets a higher utility for any parental investment. This proves (i). As a result, the maximization problem of women is
 $$\begin{eqnarray*}
&&\max _{c,\upsilon,z}\ln c+\gamma \nu(p_{\alpha}\ln (\alpha z^{\psi})+(1-p_{\alpha})\ln (\beta z^{\psi})), \\
&&\quad s.t.\text{}c+\upsilon z \le Ah, \\
&&\quad 0 \le \upsilon \le n.
\end{eqnarray*}$$
$$\begin{eqnarray*}
&&\max _{c,\upsilon,z}\ln c+\gamma \nu(p_{\alpha}\ln (\alpha z^{\psi})+(1-p_{\alpha})\ln (\beta z^{\psi})), \\
&&\quad s.t.\text{}c+\upsilon z \le Ah, \\
&&\quad 0 \le \upsilon \le n.
\end{eqnarray*}$$Writing the Lagrangian as
it is easy to see that the full fertility regime (μ ⩾ 0) prevails iff
In this case, the solution is given by
Consequently, (32) holds iff
Clearly, from this, the minimum level of human capital for a woman to be in this regime is ![]() $\underline{h},$ as defined in (1). Therefore, all women are willing to mate n times with an α man as long as they all have more human capital than
$\underline{h},$ as defined in (1). Therefore, all women are willing to mate n times with an α man as long as they all have more human capital than ![]() $\underline{h}.$
$\underline{h}.$
Next, a beta offspring with a mother of human capital h has a human capital given by βz ψ. This is larger than ![]() $\underline{h}$ iff
$\underline{h}$ iff
If the distribution of human capital is above ![]() $\underline{h},$ a sufficient condition for the distribution of human capital among offsprings to have that same property is that (37) holds at
$\underline{h},$ a sufficient condition for the distribution of human capital among offsprings to have that same property is that (37) holds at ![]() $h=\underline{h}.$ Rearranging using (1), we see that this is equivalent to (6) . This proves that (v) holds if the two assumptions in the Proposition hold. To check that (i) and (ii) hold, we need to check that it is rational for alpha men to mate. If they do so, their utility is clearly given by
$h=\underline{h}.$ Rearranging using (1), we see that this is equivalent to (6) . This proves that (v) holds if the two assumptions in the Proposition hold. To check that (i) and (ii) hold, we need to check that it is rational for alpha men to mate. If they do so, their utility is clearly given by
This is because the proportion of alpha men in the total population of men is p α, therefore each alpha man mates n/p α times. For this to be larger than utility without mating, it must be that Elnh ⩾ 0. Clearly, the expected human capital of an offspring condition on the mother’s human capital h is given by
 $$\begin{eqnarray*}
E(\ln h^{\prime} &\mid &h)=a+\psi \ln z \\
&=&a+\psi \left( \ln A+\ln h\right) +\psi \ln (\gamma \psi) -\psi \ln (1+\gamma n\psi) \\
&=&\psi (\ln h-\ln \underline{h}+1) \ge 0.
\end{eqnarray*}$$
$$\begin{eqnarray*}
E(\ln h^{\prime} &\mid &h)=a+\psi \ln z \\
&=&a+\psi \left( \ln A+\ln h\right) +\psi \ln (\gamma \psi) -\psi \ln (1+\gamma n\psi) \\
&=&\psi (\ln h-\ln \underline{h}+1) \ge 0.
\end{eqnarray*}$$This proves that Elnh ⩾ 0 and therefore that it is also in the interest of alpha men to mate. This proves claims (ii) and (iii). From the preceding formula we get that ![]() $E\ln h^{\prime}=\psi (E\ln h-\ln \underline{h}+1),$ which, once substituted into (38), proves (4). Equation (3) holds straightforwardly from (i). Equation (5) holds from substituting the optimal policies (33)–(35) into the woman’s utility function. This proves (iv).
$E\ln h^{\prime}=\psi (E\ln h-\ln \underline{h}+1),$ which, once substituted into (38), proves (4). Equation (3) holds straightforwardly from (i). Equation (5) holds from substituting the optimal policies (33)–(35) into the woman’s utility function. This proves (iv).
A.2. Proof of Proposition 2
The household maximizes
for some θ ∈ [0, 1]. The budget constraint for the couple is now
Furthermore
The Lagrangian is
 $$\begin{eqnarray*}
L &=&\theta (\ln c+\gamma \upsilon E\ln h^{\prime})+(1-\theta) (\ln c^{\ast}+\gamma \upsilon E\ln h^{\prime}) \\
&&+\;\lambda \left( A(h+h^{\ast})-\left( c+c^{\ast}+\nu z\right) \right) +\mu (n-\upsilon) +\varepsilon \upsilon .
\end{eqnarray*}$$
$$\begin{eqnarray*}
L &=&\theta (\ln c+\gamma \upsilon E\ln h^{\prime})+(1-\theta) (\ln c^{\ast}+\gamma \upsilon E\ln h^{\prime}) \\
&&+\;\lambda \left( A(h+h^{\ast})-\left( c+c^{\ast}+\nu z\right) \right) +\mu (n-\upsilon) +\varepsilon \upsilon .
\end{eqnarray*}$$ Looking at the FOCs, a regime such that ![]() $\upsilon =n$ arises iff (8)–(35) holds and if μ ⩾ 0, i.e.
$\upsilon =n$ arises iff (8)–(35) holds and if μ ⩾ 0, i.e. ![]() $\partial L/\partial \upsilon \ge 0,$ or equivalently
$\partial L/\partial \upsilon \ge 0,$ or equivalently
From λ = θ/c and (8)–(35), one can check that this inequality is equivalent to ![]() $h+h^{\ast} \ge \underline{h}(\beta) .$ One can then prove (11)–(12) by simple substitution of the optimal policies into the utility function.
$h+h^{\ast} \ge \underline{h}(\beta) .$ One can then prove (11)–(12) by simple substitution of the optimal policies into the utility function.
A.3. Proof of Proposition 3
Confronting (11) with (5), we see that for the woman to gain from marriage she must at least get a share θmin of consumption, where
 $$\begin{equation*}
\theta _{\min}=e^{k}\left( 1+\frac{h^{\ast}}{h}\right) ^{-(1+\gamma n\psi) }.
\end{equation*}$$
$$\begin{equation*}
\theta _{\min}=e^{k}\left( 1+\frac{h^{\ast}}{h}\right) ^{-(1+\gamma n\psi) }.
\end{equation*}$$The match is efficient if the man’s utility U*β(h, h*, θ), computed at θ = θmin, is greater than the man’s outside option, which is given by (3). That defines the condition under which the marriage takes place:
Substituting the value of θmin, we get Equation (13).
A.4. Proof of Proposition 4
Suppose it’s not. Then we can find two married couples, (h 0, h*1) and (h 1, h*0), such that h 0 ⩽ h 1 and h*0 = h*(h 1) ⩽ h*1 = h*(h 0). Let θ0 = θ(h 0) and θ1 = θ(h 1). For this assignment to be an equilibrium, condition (iii) in Definition 2 must hold. Let us apply it for h* = h*1 and h = h 1. Using (11), we see that
Using (12) and (17), we see that we must have
Substituting (39), we get that the following inequality must hold:
If we now apply condition (iii) to h* = h*0 and h = h 0, we get a similar condition
Adding these two inequalities, we get that the following must hold
However, the strict convexity of the function ψ(x) = x 1 + γnψ precludes it. Let ![]() $\mu =\frac{h_{1}-h_{0}}{h_{1}-h_{0}+h_{1}^{\ast}-h_{0}^{\ast}}\in [0,1].$ One has h 1 + h*0 = μ(h 1 + h*1) + (1 − μ)(h 0 + h*0), and h*1 + h 0 = (1 − μ)(h 1 + h*1) + μ(h 0 + h*0). Therefore, ψ(h*1 + h 0) ⩽ (1 − μ)ψ(h 1 + h*1) + μψ(h 0 + h*0) and ψ(h 1 + h*0) ⩽ μψ(h 1 + h*1) + (1 − μ)ψ(h 0 + h*0). Adding these two inequalities, we clearly contradict (40).
$\mu =\frac{h_{1}-h_{0}}{h_{1}-h_{0}+h_{1}^{\ast}-h_{0}^{\ast}}\in [0,1].$ One has h 1 + h*0 = μ(h 1 + h*1) + (1 − μ)(h 0 + h*0), and h*1 + h 0 = (1 − μ)(h 1 + h*1) + μ(h 0 + h*0). Therefore, ψ(h*1 + h 0) ⩽ (1 − μ)ψ(h 1 + h*1) + μψ(h 0 + h*0) and ψ(h 1 + h*0) ⩽ μψ(h 1 + h*1) + (1 − μ)ψ(h 0 + h*0). Adding these two inequalities, we clearly contradict (40).
A.5. Proof of Proposition 5
The first step is to show that if a man is married, all men with greater human capital must also be married. To see this, consider a man with human capital h*0, married with a woman with human capital h 0. Let θ0 = θ(h 0) be her corresponding equilibrium output share. A man with human capital h*1 ⩾ h 0* can marry her if he gives her a share ![]() $\hat{\theta}(h_{0},h_{1}^{\ast})$ such that
$\hat{\theta}(h_{0},h_{1}^{\ast})$ such that ![]() $U_{\beta}(h_{0},h_{1}^{\ast},\hat{\theta}(h_{0},h_{1}^{\ast}))=V(h_{0})=U_{\beta}(h_{0},h_{0}^{\ast},\theta _{0}),$ or equivalently, using (11),
$U_{\beta}(h_{0},h_{1}^{\ast},\hat{\theta}(h_{0},h_{1}^{\ast}))=V(h_{0})=U_{\beta}(h_{0},h_{0}^{\ast},\theta _{0}),$ or equivalently, using (11),
If h*1 is single, then he must not be better-off by marrying h 0 and offering her an output share equal to ![]() $\hat{\theta} (h_{0},h_{1}^{\ast});$ otherwise, (17) would be violated. Therefore, we must have
$\hat{\theta} (h_{0},h_{1}^{\ast});$ otherwise, (17) would be violated. Therefore, we must have ![]() $U_{\beta}^{\ast}(h_{0},h_{1}^{\ast},\hat{\theta} (h_{0},h_{1}^{\ast}))\le V(h_{1}^{\ast})=\bar{U}_{\beta}^{\ast}(h_{1}^{\ast}),$ or equivalently, using (12) and (3),
$U_{\beta}^{\ast}(h_{0},h_{1}^{\ast},\hat{\theta} (h_{0},h_{1}^{\ast}))\le V(h_{1}^{\ast})=\bar{U}_{\beta}^{\ast}(h_{1}^{\ast}),$ or equivalently, using (12) and (3),
Substituting (41) into (42), we see that the following inequality must hold:
At the same time, h*0 must be better-off married with h 0 than single, otherwise (16) would be violated. Using (12) and (3), this is equivalent to
Putting together (44) and (43), we see that the following inequality must hold:
Observe that ![]() $\underline{h}(\beta) =\tilde{h}\exp (1-\ln (1+\gamma n\psi) /\gamma n\psi) \ge \tilde{h},$ and that the expression
$\underline{h}(\beta) =\tilde{h}\exp (1-\ln (1+\gamma n\psi) /\gamma n\psi) \ge \tilde{h},$ and that the expression ![]() $(h_{0}+x)^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}x$ is strictly increasing in x over the relevant range provided
$(h_{0}+x)^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}x$ is strictly increasing in x over the relevant range provided ![]() $h_{\min} \ge \frac{1}{2}(1+$
$h_{\min} \ge \frac{1}{2}(1+$![]() $\gamma n\psi) ^{-\gamma n\psi}\tilde{h},$ which is clearly implied by our assumption that
$\gamma n\psi) ^{-\gamma n\psi}\tilde{h},$ which is clearly implied by our assumption that ![]() $h_{\min} \ge \underline{h}(\beta) .$ Therefore, (45) cannot hold for h*1 ⩾ h 0*. Therefore, h*1 must be married too. Consequently, it must be that
$h_{\min} \ge \underline{h}(\beta) .$ Therefore, (45) cannot hold for h*1 ⩾ h 0*. Therefore, h*1 must be married too. Consequently, it must be that ![]() $S^{\ast}=[\underline{h}^{\ast},h_{\max}].$
$S^{\ast}=[\underline{h}^{\ast},h_{\max}].$
Next, we show that the inverse assignment function h*−1() must be continuous over S*. Suppose it is not the case. Since it is monotonic, the set of its discontinuity points is at most countable. Then there exists some ![]() $h_{0}^{\ast}\in \mathring{S}$ such that h 1 = limh* → h*+0h*−1() ⩾ h*−1(h*0) = h 0.Footnote 40 Then, all women in (h 0, h 1) must be single. Furthermore, a man with human capital h*0 must be indifferent between marrying a woman with human capital h 0 or a woman with human capital (arbitrarily close to) h 1, since he prefers the former but a man with an arbitrarily close human capital to his prefers the latter. Denoting θ0 = θ(h 0) and θ1 = limh → h +1θ(h), this can be written as
$h_{0}^{\ast}\in \mathring{S}$ such that h 1 = limh* → h*+0h*−1() ⩾ h*−1(h*0) = h 0.Footnote 40 Then, all women in (h 0, h 1) must be single. Furthermore, a man with human capital h*0 must be indifferent between marrying a woman with human capital h 0 or a woman with human capital (arbitrarily close to) h 1, since he prefers the former but a man with an arbitrarily close human capital to his prefers the latter. Denoting θ0 = θ(h 0) and θ1 = limh → h +1θ(h), this can be written as
Another equilibrium condition is that all women such that h ∈ (h 0, h 1) could not be better-off if they married h*0. The woman’s output share that would leave him indifferent between marrying h 0 or h 1 and marrying h is ![]() $\hat{\theta}^{\ast}(h,h_{0}^{\ast})$ such that
$\hat{\theta}^{\ast}(h,h_{0}^{\ast})$ such that
That a woman with h ∈ (h 0, h 1) prefers to be single than marrying h*0 under these terms can be written as
Note that ![]() $\hat{\theta}^{\ast}(h_{0},h_{0}^{\ast})=\theta _{0}$ and
$\hat{\theta}^{\ast}(h_{0},h_{0}^{\ast})=\theta _{0}$ and ![]() $\hat{ \theta}^{\ast}(h_{1},h_{0}^{\ast})=\theta _{1}.$ Taking limits in (48) for h → h 0 and h → h 1 and noting that a woman with h = h 0 or h arbitrarily close to h 1 is married in equilibrium and thus not worse-off than single, we see that (48) must hold with equality at the bounds of (h 0, h 1), i.e.
$\hat{ \theta}^{\ast}(h_{1},h_{0}^{\ast})=\theta _{1}.$ Taking limits in (48) for h → h 0 and h → h 1 and noting that a woman with h = h 0 or h arbitrarily close to h 1 is married in equilibrium and thus not worse-off than single, we see that (48) must hold with equality at the bounds of (h 0, h 1), i.e.
Using (49) and (50) to eliminate θ0 and θ1 in (46), we get that
Let ϕ(h) be the function defined by ϕ(h) = (h*0 + h)1 + γnψ − ekh 1 + γnψ. It is easy to see that ϕ′(h) is positive and then negative as h goes from zero to infinity. Since ϕ(h 0) = ϕ(h 1), ϕ() must be hump-shaped between h 0 and h 1, implying that
But, substituting (47) into (48), we see that we must also have
Substituting again the value of θ0 from (49), we see that this is equivalent to
which is clearly a contradiction. Therefore, the inverse assignment function must be continuous, implying that S is an interval.
Let ![]() $\underline{h}$ be the lower bound of S. It must be that
$\underline{h}$ be the lower bound of S. It must be that ![]() $h^{\ast}( \underline{h})=\underline{h}^{\ast}.$ If
$h^{\ast}( \underline{h})=\underline{h}^{\ast}.$ If ![]() $\underline{h}^{\ast}=h_{\min},$ then all men are married, so must all women, and one must have S = [h min, h max]. The equilibrium is then Victorian. Assume then that
$\underline{h}^{\ast}=h_{\min},$ then all men are married, so must all women, and one must have S = [h min, h max]. The equilibrium is then Victorian. Assume then that ![]() $ \underline{h}^{\ast} > h_{\min}.$ Assume
$ \underline{h}^{\ast} > h_{\min}.$ Assume ![]() $\underline{h} > h_{\min}.$ Then, all women such that
$\underline{h} > h_{\min}.$ Then, all women such that ![]() $h \le \underline{h}$ are single. We can use similar steps as the ones used to derive (47)–(50) to show that
$h \le \underline{h}$ are single. We can use similar steps as the ones used to derive (47)–(50) to show that ![]() $\underline{h}$ is just indifferent between being married and single, i.e.
$\underline{h}$ is just indifferent between being married and single, i.e.
By the same token, ![]() $\underline{h}^{\ast}$ is also indifferent between being married and single, that is
$\underline{h}^{\ast}$ is also indifferent between being married and single, that is
Putting these two conditions together, we see that the marriage viability condition (13) must be satisfied with equality at ![]() $h=\underline{h}$ and
$h=\underline{h}$ and ![]() $h^{\ast}=\underline{h}^{\ast}.$ But this implies that it is satisfied strictly for any
$h^{\ast}=\underline{h}^{\ast}.$ But this implies that it is satisfied strictly for any ![]() $h < \underline{h}$ and
$h < \underline{h}$ and ![]() $h^{\ast}=\underline{h}^{\ast}.$ Therefore, a woman with human capital
$h^{\ast}=\underline{h}^{\ast}.$ Therefore, a woman with human capital ![]() $h < \underline{h}$ can underbid
$h < \underline{h}$ can underbid ![]() $ \underline{h}$ to marry
$ \underline{h}$ to marry ![]() $\underline{h}^{\ast}$ and give him a positive surplus, meaning that condition (iii) in Definition 2 must be violated. Hence, it cannot be that
$\underline{h}^{\ast}$ and give him a positive surplus, meaning that condition (iii) in Definition 2 must be violated. Hence, it cannot be that ![]() $\underline{h} > h_{\min},$ implying that if
$\underline{h} > h_{\min},$ implying that if ![]() $ \underline{h}^{\ast} > h_{\min}$ the equilibrium must be SATC.
$ \underline{h}^{\ast} > h_{\min}$ the equilibrium must be SATC.
A.6. Proof of Proposition 6
Assume (18) holds. We show that a Victorian assignment along with a sharing rule defined by (19) matches all equilibrium conditions in Definition 2. Let us start with condition (iii). Since all women are married, their reservation utility is given by
By marrying another man with human capital h* and get a fraction θ of consumption, their utility would be given by (11). Therefore, the consumption share that would make them indifferent between their marriage and this alternative marriage is given by
The new husband utility is now
It must not exceed the utility he had in his assigned marriage
Using (52) to eliminate ![]() $\hat{\theta}(h,h^{\ast}),$ and rearranging, we see that the condition
$\hat{\theta}(h,h^{\ast}),$ and rearranging, we see that the condition ![]() $U_{\beta}^{\ast}(h,h^{\ast},\hat{\theta} (h,h^{\ast}))\le V^{\ast}(h^{\ast})$ is equivalent to
$U_{\beta}^{\ast}(h,h^{\ast},\hat{\theta} (h,h^{\ast}))\le V^{\ast}(h^{\ast})$ is equivalent to
and, since the actual equilibrium assignment defines V*(h*), this will hold with equality for h* = h*(h).
In our candidate equilibrium, we have h*(h) = h. The preceding formula can simply be rewritten as
Suppose now that we have ![]() $\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),$ this boils down to
$\theta (h)=\frac{1}{2}\left( 1+\lambda h^{-(1+\gamma n\psi) }\right),$ this boils down to
 $$\begin{equation*}
\left(\frac{h+h^{\ast}}{2}\right)^{1+\gamma n\psi}\le \frac{h^{1+\gamma n\psi}+h^{\ast}{}^{1+\gamma n\psi}}{2},
\end{equation*}$$
$$\begin{equation*}
\left(\frac{h+h^{\ast}}{2}\right)^{1+\gamma n\psi}\le \frac{h^{1+\gamma n\psi}+h^{\ast}{}^{1+\gamma n\psi}}{2},
\end{equation*}$$which is true by convexity. This proves that our candidate equilibrium satisfies (iii) in Definition 2.
Let us now check condition (i), i.e. that women are better-off married than single. Comparing (51) and (5), we see that the condition ![]() $ V(h)\ge \bar{U}_{\alpha}(h)$ is equivalent to
$ V(h)\ge \bar{U}_{\alpha}(h)$ is equivalent to
Given (19), this is equivalent to
This defines the set of values of λ for which (i) in Definition 2 holds.
Turning now to condition (ii), using (53) and (3), and the fact that h*−1(h*) = h*, the condition ![]() $V^{\ast}(h^{\ast})\ge \bar{U}_{\beta}^{\ast}(h^{\ast})$ is equivalent to
$V^{\ast}(h^{\ast})\ge \bar{U}_{\beta}^{\ast}(h^{\ast})$ is equivalent to
If λ ⩾ 0 then the LHS goes up with h*; since the RHS falls with h*, then for this to hold for all h* it must hold for h* = h min. We get the condition that
Note that the RHS to this inequality is always positive.
If λ ⩽ 0 then the LHS of (55) is always greater than 0.5, and therefore always exceeds the RHS since ![]() $h^{\ast} \ge \underline{h}(\beta) \ge \tilde{h}.$
$h^{\ast} \ge \underline{h}(\beta) \ge \tilde{h}.$
Summarizing all these findings, we see that there exist values of λ which satisfy (i) and (ii) if and only if (18) holds – which is always the case if ek ⩽ 2γnψ. The relevant interval of values of λ is then clearly defined by (20).
The preceding steps imply that if (18) holds, then a Victorian equilibrium exists. Furthermore, the claims of part B hold by construction. We now prove that those conditions are necessary.
We have seen that for the equilibrium condition (87) to hold (see footnote 30), it must be that (54) holds. We also know that (54) holds with equality at h* = h*(h). Thus, h*(h) must be a local extremum of the RHS of (54) minus its LHS, as a function of h*. Locally, this means that:
 $$\begin{eqnarray}
&&(1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi} \nonumber\\
&&\quad=(1-\theta (h))(1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi}\times \left( 1+h^{\ast \prime}(h)^{-1}\right) \nonumber\\
&&\qquad-\;(h+h^{\ast}(h))^{1+\gamma n\psi}\frac{\theta ^{\prime}(h)}{h^{\ast \prime}(h)}.
\end{eqnarray}$$
$$\begin{eqnarray}
&&(1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi} \nonumber\\
&&\quad=(1-\theta (h))(1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi}\times \left( 1+h^{\ast \prime}(h)^{-1}\right) \nonumber\\
&&\qquad-\;(h+h^{\ast}(h))^{1+\gamma n\psi}\frac{\theta ^{\prime}(h)}{h^{\ast \prime}(h)}.
\end{eqnarray}$$In a Victorian equilibrium h*(h) = h, and this simplifies to
The solution to this differential equation is given by (19), which is therefore a necessary condition for a Victorian equilibrium. We then have already seen that (20) is necessary for (i) and (ii) in Definition 2 to be satisfied, and that this interval of values of λ is nonempty if and only if (18) holds. This proves that (18) is necessary and that the conditions in B necessarily hold.
A.7. Proof of Proposition 7
A. Constructing the assignment for the SATC equilibrium
We now show that an SATC equilibrium can be constructed. For this, we look for a pair (![]() $\underline h$
$\underline h$![]() $^{\ast},\bar{h})$ such that
$^{\ast},\bar{h})$ such that ![]() $\underline h$* ⩾ h min,
$\underline h$* ⩾ h min, ![]() $\bar{h} \le h_{\max},$ and the assignment given by
$\bar{h} \le h_{\max},$ and the assignment given by
is an equilibrium one. Clearly, given ![]() $\bar{h},$ if we choose
$\bar{h},$ if we choose
the candidate assignment will map ![]() $S=[h_{\min},\bar{h}]$ to S* = [
$S=[h_{\min},\bar{h}]$ to S* = [![]() $\underline h$*, h max] and satisfy (14). Therefore, it is indeed an assignment:
$\underline h$*, h max] and satisfy (14). Therefore, it is indeed an assignment:
• We have proved that given any
 $\bar{h},$ the value of
$\bar{h},$ the value of  $\underline{h} ^{\ast}$ given by (59) and the h*() function defined by (58) are an assignment.
$\underline{h} ^{\ast}$ given by (59) and the h*() function defined by (58) are an assignment.
B. Checking that married people cannot underbid one another
Next, let us assume that the sharing function θ(h) satisfies (23). We show that (iii) in Definition 2 holds for h ∈ S and h* ∈ S*. As shown in the proof of Proposition 6, this is equivalent to (54). Substituting (23), we get that this is equivalent to
 $$\begin{equation}
(h+h^{\ast})^{1+\gamma n\psi}\le (h^{\ast -1}(h^{\ast})+h^{\ast})^{1+\gamma n\psi}+(1+\gamma n\psi) \int _{h^{\ast -1}(h^{\ast})}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz,\end{equation}$$
$$\begin{equation}
(h+h^{\ast})^{1+\gamma n\psi}\le (h^{\ast -1}(h^{\ast})+h^{\ast})^{1+\gamma n\psi}+(1+\gamma n\psi) \int _{h^{\ast -1}(h^{\ast})}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz,\end{equation}$$for all h, h* ∈ S × S*. Clearly, equality holds for h* = h*(h). Furthermore, the derivative of the RHS of (60) with respect to h* is (1 + γnψ)(h*−1(h*) + h*)γnψ, while the derivative of the LHS is (1 + γnψ)(h + h*)γnψ. Given that h*(h) is increasing, the former is clearly larger than the latter for h* ⩾ h*(h), and smaller for h* ⩽ h*(h). Consequently, the difference between the RHS and the LHS reaches it minimum at h* = h*(h); hence (60) holds.
• We have proved that if θ(h) satisfies (23), then condition (iii) holds for (h, h*) ∈ S × S*.
C. Deriving the value-matching conditions at the frontier of S and S*
Next, we show that there exist values for ![]() $\mu,\bar{h}$ and
$\mu,\bar{h}$ and ![]() $\underline h$* such that, in addition to (59), the two following conditions hold:
$\underline h$* such that, in addition to (59), the two following conditions hold:
These two conditions mean that the reservation utilities V() and V*() do not jump as one crosses the boundaries of S and S*. Otherwise, the equilibrium conditions would be violated. Suppose, for example, that a woman such that h is marginally higher than ![]() $\bar{h}$ has a utility higher than
$\bar{h}$ has a utility higher than ![]() $U_{\beta}(\bar{h},h_{\max},\theta (\bar{h}))$ by a discrete amount. Then, since the θ() function is continuous over S, women with h below
$U_{\beta}(\bar{h},h_{\max},\theta (\bar{h}))$ by a discrete amount. Then, since the θ() function is continuous over S, women with h below ![]() $\bar{h}$ but arbitrarily close to it would be better-off being single, and condition (i) in Definition 2 would be violated. Suppose now that a woman with h marginally higher than
$\bar{h}$ but arbitrarily close to it would be better-off being single, and condition (i) in Definition 2 would be violated. Suppose now that a woman with h marginally higher than ![]() $\bar{h}$ has a utility lower than
$\bar{h}$ has a utility lower than ![]() $U_{\beta}(\bar{h},h_{\max},\theta (\bar{h}))$ by a discrete amount. Then
$U_{\beta}(\bar{h},h_{\max},\theta (\bar{h}))$ by a discrete amount. Then ![]() $\hat{\theta}(h,h_{\max}) \le \theta (\bar{h}):$ since these women are arbitrarily close to
$\hat{\theta}(h,h_{\max}) \le \theta (\bar{h}):$ since these women are arbitrarily close to ![]() $\bar{h},$ but have a discretely lower utility than the married women with
$\bar{h},$ but have a discretely lower utility than the married women with ![]() $\bar{h},$ they can reach that same utility by marrying a man with h max and get a lower fraction of the surplus. But the h max man would then be better-off and this would violate condition (iii). Therefore, (61) must hold. A similar reasoning applies to (62). Using (5) and (11), we see that (61) is equivalent to
$\bar{h},$ they can reach that same utility by marrying a man with h max and get a lower fraction of the surplus. But the h max man would then be better-off and this would violate condition (iii). Therefore, (61) must hold. A similar reasoning applies to (62). Using (5) and (11), we see that (61) is equivalent to
Substituting (23), we see that this is equivalent to
 $$\begin{equation}
\mu =e^{k}\bar{h}^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz=\mu _{H}(\bar{h}) .
\end{equation}$$
$$\begin{equation}
\mu =e^{k}\bar{h}^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz=\mu _{H}(\bar{h}) .
\end{equation}$$Similarly, we can substitute (3) and (12) into (62) and get
or equivalently, given (23),
Equations (65) and (64) define a 2 × 2 system in ![]() $\bar{h}$ and μ, where
$\bar{h}$ and μ, where ![]() $\underline{h}^{\ast}$ is implicitly treated as a function of
$\underline{h}^{\ast}$ is implicitly treated as a function of ![]() $ \bar{h}$ defined by (59).
$ \bar{h}$ defined by (59).
D. Showing that there is a solution, for ![]() $\mu,\bar{h},\underline{h} ^{\ast}$which satisfies the value-matching conditions as well as condition (iii) in Definition 2 for pairs of singles
$\mu,\bar{h},\underline{h} ^{\ast}$which satisfies the value-matching conditions as well as condition (iii) in Definition 2 for pairs of singles
To prove that it has a solution, we use the intermediate value theorem. First, we show that μH(h max) ⩾ μL(h max). If ![]() $\bar{h} =h_{\max}$, then h*(h) = h. Therefore, μH(h max) = ekh 1 + γnψmax − 2γnψ(h 1 + γnψmax − h 1 + γnψmin), and μL(h max) =
$\bar{h} =h_{\max}$, then h*(h) = h. Therefore, μH(h max) = ekh 1 + γnψmax − 2γnψ(h 1 + γnψmax − h 1 + γnψmin), and μL(h max) = ![]() $2^{1+\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h} ^{\gamma n\psi}h_{\min}.$ Clearly, the condition
$2^{1+\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h} ^{\gamma n\psi}h_{\min}.$ Clearly, the condition ![]() $e^{k}h_{\max}^{1+\gamma n\psi}-2^{\gamma n\psi}\left( h_{\max}^{1+\gamma n\psi}-h_{\min}^{1+\gamma n\psi}\right) \ge 2^{1+\gamma n\psi}h_{\min}^{1+\gamma n\psi}- \tilde{h}^{\gamma n\psi}h_{\min}$ is equivalent to (18) being violated, which is true by assumption.
$e^{k}h_{\max}^{1+\gamma n\psi}-2^{\gamma n\psi}\left( h_{\max}^{1+\gamma n\psi}-h_{\min}^{1+\gamma n\psi}\right) \ge 2^{1+\gamma n\psi}h_{\min}^{1+\gamma n\psi}- \tilde{h}^{\gamma n\psi}h_{\min}$ is equivalent to (18) being violated, which is true by assumption.
Next, let ![]() $\hat{h}$ be the minimum possible value of
$\hat{h}$ be the minimum possible value of ![]() $\bar{h}$ such that condition (iii) in Definition 2 holds for
$\bar{h}$ such that condition (iii) in Definition 2 holds for ![]() $h \ge \bar{h}$ and
$h \ge \bar{h}$ and ![]() $h^{\ast} \le \underline{h}^{\ast}$. The threshold
$h^{\ast} \le \underline{h}^{\ast}$. The threshold ![]() $\hat{h}$ is such that a marriage between the least skilled single woman and the most skilled single man is barely viable, i.e.
$\hat{h}$ is such that a marriage between the least skilled single woman and the most skilled single man is barely viable, i.e.
Observe that (59) implies that ![]() $\underline{h}^{\ast \prime}() \le 0,$ while (65) states that
$\underline{h}^{\ast \prime}() \le 0,$ while (65) states that ![]() $(\underline{h}^{\ast}(\hat{h}),\hat{h})$ lies on the upward sloping marriage viability frontier. Thus, (65) holds for at most one value of
$(\underline{h}^{\ast}(\hat{h}),\hat{h})$ lies on the upward sloping marriage viability frontier. Thus, (65) holds for at most one value of ![]() $\hat{h}.$ Furthermore, at
$\hat{h}.$ Furthermore, at ![]() $\hat{h}=h_{\max}$, we have that
$\hat{h}=h_{\max}$, we have that ![]() $\underline{h}^{\ast}(h_{\max})=h_{\min}$ and that
$\underline{h}^{\ast}(h_{\max})=h_{\min}$ and that
To see this, note that since (18) is violated by assumption, we have that
Clearly, the inequality
holds. This proves that (66) holds and therefore that the LHS of (65) is smaller than its RHS at ![]() $\hat{h}=h_{\max}.$ Next, for B small enough, at
$\hat{h}=h_{\max}.$ Next, for B small enough, at ![]() $\hat{h}=h_{\min},$ we have that
$\hat{h}=h_{\min},$ we have that ![]() $\underline{h}^{\ast}(h_{\min})=h_{\max}$ and that
$\underline{h}^{\ast}(h_{\min})=h_{\max}$ and that
To see this, note that if (18) holds with equality, then
or equivalently
 $$\begin{eqnarray*}
e^{k}-2^{\gamma n\psi} &=&2^{\gamma n\psi}\frac{h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}}{h_{\max}^{1+\gamma n\psi}} \\
& < &2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi},
\end{eqnarray*}$$
$$\begin{eqnarray*}
e^{k}-2^{\gamma n\psi} &=&2^{\gamma n\psi}\frac{h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}}{h_{\max}^{1+\gamma n\psi}} \\
& < &2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi},
\end{eqnarray*}$$
where the inequality comes from the fact that ![]() $h_{\min} > \underline{h}(\beta) \ge \tilde{h},$ hence the numerator in the fraction is ⩾ 0. This inequality is then equivalent to
$h_{\min} > \underline{h}(\beta) \ge \tilde{h},$ hence the numerator in the fraction is ⩾ 0. This inequality is then equivalent to
Observe that the function ![]() $(x+h_{\min})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}x$ is increasing with x for x ⩾ 0 since
$(x+h_{\min})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}x$ is increasing with x for x ⩾ 0 since ![]() $h_{\min} > \tilde{h},$ which implies that (67) holds since (68) does. Since (67) holds if (18) holds with equality, by continuity it will also hold if it is marginally violated, i.e. if B is small enough.
$h_{\min} > \tilde{h},$ which implies that (67) holds since (68) does. Since (67) holds if (18) holds with equality, by continuity it will also hold if it is marginally violated, i.e. if B is small enough.
Together, (66) and (67) imply that ![]() $\hat{h}$ exists and satisfies
$\hat{h}$ exists and satisfies ![]() $h_{\min} \le \hat{h} \le h_{\max}.$
$h_{\min} \le \hat{h} \le h_{\max}.$
We now show that ![]() $\mu _{H}(\hat{h}) \le \mu _{L}(\hat{h}).$ Substituting (65) into (63), we see that this is equivalent to
$\mu _{H}(\hat{h}) \le \mu _{L}(\hat{h}).$ Substituting (65) into (63), we see that this is equivalent to
 $$\begin{equation*}
(\hat{h}+\underline{h}^{\ast}(\hat{h}))^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{\hat{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \le (h_{\min}+\underline{h}^{\ast}(\hat{h}))^{1+\gamma n\psi}.
\end{equation*}$$
$$\begin{equation*}
(\hat{h}+\underline{h}^{\ast}(\hat{h}))^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{\hat{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \le (h_{\min}+\underline{h}^{\ast}(\hat{h}))^{1+\gamma n\psi}.
\end{equation*}$$ This inequality always holds.Footnote 41 Thus, by the intermediate value theorem, there exists a solution to (63)–(64) such that ![]() $ \hat{h} \le \bar{h} \le h_{\max}.$ Since
$ \hat{h} \le \bar{h} \le h_{\max}.$ Since ![]() $\bar{h} \ge \hat{h},$ by construction, no single woman in this solution wants to marry a single men; hence condition (iii) in Definition 2 holds for pairs of singles.
$\bar{h} \ge \hat{h},$ by construction, no single woman in this solution wants to marry a single men; hence condition (iii) in Definition 2 holds for pairs of singles.
• We have proved that there exists a pair
 $(\mu,\bar{h})$ such that (63)–(64) hold and that condition (iii) in Definition 2 holds for (h, h*) ∈ [h min, h max] − S × [h min, h max] − S*.
$(\mu,\bar{h})$ such that (63)–(64) hold and that condition (iii) in Definition 2 holds for (h, h*) ∈ [h min, h max] − S × [h min, h max] − S*.
E. Checking that the constructed solution satisfies (iii) for a single woman and a married man
Another requirement is that condition (iii) hold for ![]() $h \ge \bar{h}$ and h* ∈ S*. Using (5) and (11), we must have
$h \ge \bar{h}$ and h* ∈ S*. Using (5) and (11), we must have
Using (12), we see that (17) is equivalent to
 $$\begin{eqnarray}
&&\ln (1-\hat{\theta}(h,h^{\ast}))+(1+\gamma n\psi) (\ln A+\ln (h+h^{\ast}))+\pi _{\beta} \nonumber \\
&\le &V^{\ast}(h^{\ast}) \nonumber \\
&=&\ln (1-\theta (h^{\ast -1}(h^{\ast})))+(1+\gamma n\psi) (\ln A+\ln (h^{\ast}+h^{\ast -1}(h^{\ast})))+\pi _{\beta .}
\end{eqnarray}$$
$$\begin{eqnarray}
&&\ln (1-\hat{\theta}(h,h^{\ast}))+(1+\gamma n\psi) (\ln A+\ln (h+h^{\ast}))+\pi _{\beta} \nonumber \\
&\le &V^{\ast}(h^{\ast}) \nonumber \\
&=&\ln (1-\theta (h^{\ast -1}(h^{\ast})))+(1+\gamma n\psi) (\ln A+\ln (h^{\ast}+h^{\ast -1}(h^{\ast})))+\pi _{\beta .}
\end{eqnarray}$$Substituting (69) and (23), we see that this is equivalent to
 $$\begin{eqnarray}
\mu &\le &(h^{\ast}+h^{\ast -1}(h^{\ast}))^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{h^{\ast -1}(h^{\ast})}(z+h^{\ast}(z))^{\gamma n\psi}dz \\
&&+e^{k}h^{1+\gamma n\psi}-(h+h^{\ast})^{1+\gamma n\psi} \nonumber \\
&=&\phi (h,h^{\ast}). \nonumber
\end{eqnarray}$$
$$\begin{eqnarray}
\mu &\le &(h^{\ast}+h^{\ast -1}(h^{\ast}))^{1+\gamma n\psi}-\left( 1+\gamma n\psi \right) \int _{h_{\min}}^{h^{\ast -1}(h^{\ast})}(z+h^{\ast}(z))^{\gamma n\psi}dz \\
&&+e^{k}h^{1+\gamma n\psi}-(h+h^{\ast})^{1+\gamma n\psi} \nonumber \\
&=&\phi (h,h^{\ast}). \nonumber
\end{eqnarray}$$ Since (63) holds by construction, we have that ![]() $\phi (\bar{h},h_{\max})=\mu .$ Furthermore,
$\phi (\bar{h},h_{\max})=\mu .$ Furthermore,
Therefore, ![]() $\frac{\partial \phi}{\partial h}\ge 0$ if and only if
$\frac{\partial \phi}{\partial h}\ge 0$ if and only if ![]() $e^{k}\ge (1+\frac{h^{\ast}}{h})^{\gamma n\psi}.$ Let us assume that
$e^{k}\ge (1+\frac{h^{\ast}}{h})^{\gamma n\psi}.$ Let us assume that
It must then be that ![]() $e^{k}\ge (1+\frac{h^{\ast}}{h})^{\gamma n\psi}$ for all h* ∈ S* and for all
$e^{k}\ge (1+\frac{h^{\ast}}{h})^{\gamma n\psi}$ for all h* ∈ S* and for all ![]() $h\ge \bar{h}.$ Consequently,
$h\ge \bar{h}.$ Consequently, ![]() $\phi (h,h^{\ast})\ge \phi (\bar{h},h^{\ast}).$
$\phi (h,h^{\ast})\ge \phi (\bar{h},h^{\ast}).$
Furthermore, since ![]() $\bar{h}\in S,$ (17) is equivalent to (54) for
$\bar{h}\in S,$ (17) is equivalent to (54) for ![]() $(\bar{h},h^{\ast}),$ and we already know that from part B of this proof that (54) holds for
$(\bar{h},h^{\ast}),$ and we already know that from part B of this proof that (54) holds for ![]() $(\bar{h},h^{\ast}).$ Using (23) and rearranging, we see that this is equivalent to
$(\bar{h},h^{\ast}).$ Using (23) and rearranging, we see that this is equivalent to
 $$\begin{equation*}
(\bar{h}+h^{\ast})^{1+\gamma n\psi}\le \left( 1+\gamma n\psi \right) \int _{h^{\ast -1}(h^{\ast})}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz+(h^{\ast}+h^{\ast -1}(h^{\ast}))^{1+\gamma n\psi};
\end{equation*}$$
$$\begin{equation*}
(\bar{h}+h^{\ast})^{1+\gamma n\psi}\le \left( 1+\gamma n\psi \right) \int _{h^{\ast -1}(h^{\ast})}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz+(h^{\ast}+h^{\ast -1}(h^{\ast}))^{1+\gamma n\psi};
\end{equation*}$$
but inspection of (71) and making use of (63) shows that this condition is equivalent to ![]() $\phi (\bar{h},h^{\ast})\ge \mu .$ Therefore, ϕ(h, h*) ⩾ μ.
$\phi (\bar{h},h^{\ast})\ge \mu .$ Therefore, ϕ(h, h*) ⩾ μ.
Hence, condition (72) is sufficient for (iii) in Definition 2 to hold for h* ∈ S* and ![]() $h \ge \bar{h}.$ Furthermore, if condition (18) holds with equality, we have that ek ⩾ 2γnψ,and in this limit case the solution to (63)–(64) is
$h \ge \bar{h}.$ Furthermore, if condition (18) holds with equality, we have that ek ⩾ 2γnψ,and in this limit case the solution to (63)–(64) is ![]() $\bar{h}=h_{\max}.$ Condition (72) then strictly holds. By continuity, if h max is such that (18) is not violated by too much, i.e. B in (21) is not too large, then (72) will hold.
$\bar{h}=h_{\max}.$ Condition (72) then strictly holds. By continuity, if h max is such that (18) is not violated by too much, i.e. B in (21) is not too large, then (72) will hold.
• We have proved that we can choose B such that the values of μ and
 $\bar{h}$ constructed in D are such that condition (iii) in Definition 2 holds for (h, h*) ∈ [h min, h max] − S × S*.
$\bar{h}$ constructed in D are such that condition (iii) in Definition 2 holds for (h, h*) ∈ [h min, h max] − S × S*.
F. Checking that the constructed solution satisfies (i)
The condition that married women are better-off than if they were single can be written
or equivalently using the formula for θ(h):
 $$\begin{equation}
\mu \ge e^{k}h^{1+\gamma n\psi}-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz=\phi (h).
\end{equation}$$
$$\begin{equation}
\mu \ge e^{k}h^{1+\gamma n\psi}-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz=\phi (h).
\end{equation}$$ Again, (63) implies that it holds with equality at ![]() $h=\bar{h}.$ Furthermore, ϕ′(h) = (1 + γnψ)(ekh γnψ − (h + h*(h))γnψ). We have ϕ′(h) ⩾ 0 if and only if
$h=\bar{h}.$ Furthermore, ϕ′(h) = (1 + γnψ)(ekh γnψ − (h + h*(h))γnψ). We have ϕ′(h) ⩾ 0 if and only if ![]() $h^{\ast}(h)/h \le e^{\frac{k}{\gamma n\psi}}-1.$ This is again true in the limit case where (18) holds with equality, since we then have h*(h) = h and
$h^{\ast}(h)/h \le e^{\frac{k}{\gamma n\psi}}-1.$ This is again true in the limit case where (18) holds with equality, since we then have h*(h) = h and ![]() $e^{\frac{k}{\gamma n\psi}} \ge 2.$ Therefore, in this limit equilibrium we have
$e^{\frac{k}{\gamma n\psi}} \ge 2.$ Therefore, in this limit equilibrium we have ![]() $\phi (h) \le \phi (\bar{h}).$ By continuity, this remains true if (18) is not violated by too much. Then (74) holds, and condition (i) in Definition 2 is satisfied.
$\phi (h) \le \phi (\bar{h}).$ By continuity, this remains true if (18) is not violated by too much. Then (74) holds, and condition (i) in Definition 2 is satisfied.
• We have proved that we can choose B such that, in addition to the properties spelled out above, condition (i) in Definition 2 holds.
G. Checking that the constructed solution satisfies (iii) for a single man and a married woman
We now prove that (iii) holds when ![]() $h^{\ast} \le \underline{h}^{\ast}$ and
$h^{\ast} \le \underline{h}^{\ast}$ and ![]() $h \le \bar{h}.$ Here, it is more convenient to use the alternative formulation defined in footnote 30. Using (3) and (12) allows us to compute
$h \le \bar{h}.$ Here, it is more convenient to use the alternative formulation defined in footnote 30. Using (3) and (12) allows us to compute ![]() $\hat{\theta}^{\ast}(h,h^{\ast}):$
$\hat{\theta}^{\ast}(h,h^{\ast}):$
Comparing (11) for ![]() $\theta =\hat{\theta}^{\ast}(h,h^{\ast})$ and for θ = θ(h) and h*(h) instead of h*, we see that the condition in footnote 30 holds if and only if
$\theta =\hat{\theta}^{\ast}(h,h^{\ast})$ and for θ = θ(h) and h*(h) instead of h*, we see that the condition in footnote 30 holds if and only if
Substituting (23) and (75), we get the following condition
 $$\begin{equation}
(h+h^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h^{\ast}\le \mu +(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$
$$\begin{equation}
(h+h^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h^{\ast}\le \mu +(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$ Note that this holds with equality for h = h min and ![]() $h^{\ast}= \underline{h}^{\ast},$ by virtue of (64). Next, note that the LHS is an increasing function of h*. Therefore, (76) holds for all
$h^{\ast}= \underline{h}^{\ast},$ by virtue of (64). Next, note that the LHS is an increasing function of h*. Therefore, (76) holds for all ![]() $ h^{\ast} \le \underline{h}^{\ast}$ if and only if it holds for
$ h^{\ast} \le \underline{h}^{\ast}$ if and only if it holds for ![]() $h^{\ast}= \underline{h}^{\ast}.~$Next, note that the derivative of the RHS with respect to h is (1 + γnψ)(h + h*(h))γnψ, while the derivative of the LHS with respect to h at
$h^{\ast}= \underline{h}^{\ast}.~$Next, note that the derivative of the RHS with respect to h is (1 + γnψ)(h + h*(h))γnψ, while the derivative of the LHS with respect to h at ![]() $h^{\ast}=\underline{h} ^{\ast}$ is
$h^{\ast}=\underline{h} ^{\ast}$ is ![]() $(1+\gamma n\psi) (h+\underline{h}^{\ast})^{\gamma n\psi}.$ The former is clearly larger than the latter since
$(1+\gamma n\psi) (h+\underline{h}^{\ast})^{\gamma n\psi}.$ The former is clearly larger than the latter since ![]() $h^{\ast}(h)\ge \underline{h}^{\ast}.$ Therefore, the difference between the RHS of (76) and its LHS at
$h^{\ast}(h)\ge \underline{h}^{\ast}.$ Therefore, the difference between the RHS of (76) and its LHS at ![]() $h^{\ast}=\underline{h}^{\ast}$ is an increasing function of h. Since (76) holds with equality for h = h min and
$h^{\ast}=\underline{h}^{\ast}$ is an increasing function of h. Since (76) holds with equality for h = h min and ![]() $h^{\ast}=\underline{h}^{\ast},$ it also holds for any h ⩾ h min and
$h^{\ast}=\underline{h}^{\ast},$ it also holds for any h ⩾ h min and ![]() $h^{\ast}=\underline{h}^{\ast}.$ As we have already seen, that in turn implies that it holds for any h ⩾ h min and
$h^{\ast}=\underline{h}^{\ast}.$ As we have already seen, that in turn implies that it holds for any h ⩾ h min and ![]() $h^{\ast} \le \underline{h} ^{\ast}.$ This completes the proof that (iii) holds for single men underbidders.
$h^{\ast} \le \underline{h} ^{\ast}.$ This completes the proof that (iii) holds for single men underbidders.
• We have proved that the values of μ and
 $\bar{h}$ constructed in D are such that condition (iii) in Definition 2 holds for (h, h*) ∈ S × [h min, h max] − S*.
$\bar{h}$ constructed in D are such that condition (iii) in Definition 2 holds for (h, h*) ∈ S × [h min, h max] − S*.
H. Proof that (ii) holds for the constructed solution
The last thing we have to check is that (ii) holds, that is, married men are better-off than if they were single. Denoting by h the wife of a married man and by h*(h) this man, we see that this is equivalent to
Substituting in (23), we see that this is equivalent to
 $$\begin{equation}
\mu \le (h+h^{\ast}(h))^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h^{\ast}(h)-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$
$$\begin{equation}
\mu \le (h+h^{\ast}(h))^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h^{\ast}(h)-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$ Again, this holds with equality for h = h min, because of (64). Furthermore, the RHS’s derivative with respect to h is equal to ![]() $h^{\ast \prime}(h)\left[ (1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi}-\tilde{h} ^{\gamma n\psi}\right],$ which is clearly positive since h*() is increasing and
$h^{\ast \prime}(h)\left[ (1+\gamma n\psi) (h+h^{\ast}(h))^{\gamma n\psi}-\tilde{h} ^{\gamma n\psi}\right],$ which is clearly positive since h*() is increasing and ![]() $h+h^{\ast}(h) \ge \underline{h}(\beta) \ge \tilde{h}.$ Therefore, the RHS of (77) is an increasing function of h and is always greater for h ⩾ h min than for h = h min, where it holds with equality. Hence, (77) always holds:
$h+h^{\ast}(h) \ge \underline{h}(\beta) \ge \tilde{h}.$ Therefore, the RHS of (77) is an increasing function of h and is always greater for h ⩾ h min than for h = h min, where it holds with equality. Hence, (77) always holds:
• We have proved that the values of μ and
 $\bar{h}$ constructed in D are such that condition (ii) in Definition 2 holds.
$\bar{h}$ constructed in D are such that condition (ii) in Definition 2 holds.
This completes the proof of Proposition 7.
A.8. Proof of Proposition 8
The discussion in D in the proof of proposition 7 implies that we can always pick an equilibrium such that locally, the RHS of (63) as a function of ![]() $\underline{h}^{\ast}$ is flatter than that of (64). It is then clear that a rise in k shifts the RHS of (63) up, and has no effect on (64). Therefore, both
$\underline{h}^{\ast}$ is flatter than that of (64). It is then clear that a rise in k shifts the RHS of (63) up, and has no effect on (64). Therefore, both ![]() $\underline{h}^{\ast}$ and μ go up, which proves claim (i). Similarly, a greater
$\underline{h}^{\ast}$ and μ go up, which proves claim (i). Similarly, a greater ![]() $\tilde{h}$ reduces the RHS of (64), with no effect on (63), so that
$\tilde{h}$ reduces the RHS of (64), with no effect on (63), so that ![]() $\underline{h} ^{\ast}$ goes up again while μ falls. This proves claim (ii).
$\underline{h} ^{\ast}$ goes up again while μ falls. This proves claim (ii).
Finally, note that h max does not enter in (64) and that for a uniform distribution, (63) is equivalent to
where ![]() $\delta =\underline{h}^{\ast}-h_{\min},$ and h*(h) = h + δ. The constructed equilibrium is such that (72) holds. This also implies that the RHS of (78) is increasing in h max, holding
$\delta =\underline{h}^{\ast}-h_{\min},$ and h*(h) = h + δ. The constructed equilibrium is such that (72) holds. This also implies that the RHS of (78) is increasing in h max, holding ![]() $\underline{h}^{\ast}$ or equivalently δ constant. Consequently, a greater h max raises the RHS of (63), so that the equilibrium values of μ and
$\underline{h}^{\ast}$ or equivalently δ constant. Consequently, a greater h max raises the RHS of (63), so that the equilibrium values of μ and ![]() $\underline{h}^{\ast}$ go up. The proportion of married people is
$\underline{h}^{\ast}$ go up. The proportion of married people is ![]() $1-\frac{\delta}{h_{\max}-h_{\min}},$ and it must go down. It falls iff
$1-\frac{\delta}{h_{\max}-h_{\min}},$ and it must go down. It falls iff ![]() $\frac{d\delta}{dh_{\max}} \ge \frac{\delta}{h_{\max}-h_{\min}},$ which is true if δ is small enough, which is true in the constructed equilibrium of Prop. 7.
$\frac{d\delta}{dh_{\max}} \ge \frac{\delta}{h_{\max}-h_{\min}},$ which is true if δ is small enough, which is true in the constructed equilibrium of Prop. 7.
A.9. Proof of Proposition 9
Since the formula for θ(h) is the same as in Proposition 6, it is clear that (54) is still satisfied by our candidate equilibrium and therefore that equilibrium condition (iii) in Definition 2 holds.
The utility of a beta woman outside marriage is now given by ![]() $\bar{U}_{\beta}^{\ast}(h)$; like beta men, they cannot have children and are therefore in a symmetrical situation. Using (51) and (3), we see that condition (i) in Definition 2 holds if and only if
$\bar{U}_{\beta}^{\ast}(h)$; like beta men, they cannot have children and are therefore in a symmetrical situation. Using (51) and (3), we see that condition (i) in Definition 2 holds if and only if
This condition always holds for λ ⩾ 0 since ![]() $h \ge \tilde{h}.$ For λ ⩽ 0, the LHS is clearly an increasing function of h, so (i) holds provided the above holds for h = h min, that is
$h \ge \tilde{h}.$ For λ ⩽ 0, the LHS is clearly an increasing function of h, so (i) holds provided the above holds for h = h min, that is ![]() $\lambda \ge -\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},$ which defines the lower bound of (25).
$\lambda \ge -\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},$ which defines the lower bound of (25).
For men, the proof that (ii) holds if ![]() $\lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ is the same as in the proof of Proposition 6.
$\lambda \le \left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ is the same as in the proof of Proposition 6.
A.10. Proof of Proposition 10
We compare utility in the two types of equilibria for all agents. In all what follows, h*(h) refers to the assignment function in the SATC equilibrium, since in the Victorian equilibrium we can readily replace it by h.
We start with women who are married in the SATC equilibrium. Using (11) for both equilibria, we see that VS(h) ⩾ VV(h; λ) if and only if
where θS(h) and θV(h; λ) are the appropriate shares, i.e.
 $$\begin{eqnarray*}
\theta _{V}(h,\lambda) &=&\frac{1}{2}(1+\lambda h^{-(1+\gamma n\psi) }), \\
\theta _{S}(h) &=&\left( h+h^{\ast}(h)\right) ^{-(1+\gamma n\psi) }\left[ (1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \right] .
\end{eqnarray*}$$
$$\begin{eqnarray*}
\theta _{V}(h,\lambda) &=&\frac{1}{2}(1+\lambda h^{-(1+\gamma n\psi) }), \\
\theta _{S}(h) &=&\left( h+h^{\ast}(h)\right) ^{-(1+\gamma n\psi) }\left[ (1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \right] .
\end{eqnarray*}$$Condition (79) is equivalent to
 $$\begin{equation}
(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \ge 2^{\gamma n\psi}(\lambda +h^{1+\gamma n\psi}).
\end{equation}$$
$$\begin{equation}
(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \ge 2^{\gamma n\psi}(\lambda +h^{1+\gamma n\psi}).
\end{equation}$$ Clearly, if it holds for the maximum possible value of λ, it must hold for any equilibrium value of λ. Hence, substituting ![]() $\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ into (80), we get
$\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ into (80), we get
 $$\begin{equation}
\mu \ge 2^{\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}+2^{\gamma n\psi}h^{1+\gamma n\psi}-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$
$$\begin{equation}
\mu \ge 2^{\gamma n\psi}h_{\min}^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}+2^{\gamma n\psi}h^{1+\gamma n\psi}-(1+\gamma n\psi) \int _{h_{\min}}^{h}(z+h^{\ast}(z))^{\gamma n\psi}dz.
\end{equation}$$By differentiating the last two terms with respect to h, we see that together they are a decreasing function of h. Therefore the preceding inequality will hold for all h iff it holds for h min, that is
Subsituting (64), which must hold in any SATC equilibrium, we get
Noting that there is equality at ![]() $\underline{h}^{\ast}=h_{\min}$ and that the LHS is increasing with
$\underline{h}^{\ast}=h_{\min}$ and that the LHS is increasing with ![]() $\underline{h}^{\ast},$ we conclude that this always holds. Consequently, (81) holds for all
$\underline{h}^{\ast},$ we conclude that this always holds. Consequently, (81) holds for all ![]() $h \le \bar{h}.$ Therefore, (80) holds for all λ and
$h \le \bar{h}.$ Therefore, (80) holds for all λ and ![]() $h \le \bar{h}.$ Hence married beta women have a greater utility under the SATC equilibrium than under the Victorian one.
$h \le \bar{h}.$ Hence married beta women have a greater utility under the SATC equilibrium than under the Victorian one.
We next consider single women. Comparing (5) and (11), we get that VS(h) ⩾ VV(h; λ) iff
or equivalently
Again, if this inequality holds for the maximum equilibrium value of λ, it will hold for any of them. Furthermore, at this maximum λ, which is positive, the RHS is decreasing in h, so that the inequality holds for all ![]() $h\ge \bar{h}$ iff it holds for
$h\ge \bar{h}$ iff it holds for ![]() $h=\bar{h}.$ Substituting both
$h=\bar{h}.$ Substituting both ![]() $\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ and
$\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}$ and ![]() $h= \bar{h}$ into (82) we get, after rearranging:
$h= \bar{h}$ into (82) we get, after rearranging:
Substituting (63) and then (64), this is equivalent to
 $$\begin{eqnarray}
&&(h_{\min}+\underline{h}^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}\underline{h}^{\ast}+(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}\bar{h}^{1+\gamma n\psi}+h_{\min}^{1+\gamma n\psi}2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}.
\end{eqnarray}$$
$$\begin{eqnarray}
&&(h_{\min}+\underline{h}^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}\underline{h}^{\ast}+(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}\bar{h}^{1+\gamma n\psi}+h_{\min}^{1+\gamma n\psi}2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}.
\end{eqnarray}$$ Since ![]() $(h_{\min}+\underline{h}^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}\underline{h}^{\ast} \ge h_{\min}^{1+\gamma n\psi}2^{1+\gamma n\psi}- \tilde{h}^{\gamma n\psi}h_{\min},$ a sufficient condition for (83) to hold is
$(h_{\min}+\underline{h}^{\ast})^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}\underline{h}^{\ast} \ge h_{\min}^{1+\gamma n\psi}2^{1+\gamma n\psi}- \tilde{h}^{\gamma n\psi}h_{\min},$ a sufficient condition for (83) to hold is
 $$\begin{eqnarray*}
&&h_{\min}^{1+\gamma n\psi}2^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}+(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}\bar{h}^{1+\gamma n\psi}+h_{\min}^{1+\gamma n\psi}2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min},
\end{eqnarray*}$$
$$\begin{eqnarray*}
&&h_{\min}^{1+\gamma n\psi}2^{1+\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min}+(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}\bar{h}^{1+\gamma n\psi}+h_{\min}^{1+\gamma n\psi}2^{\gamma n\psi}-\tilde{h}^{\gamma n\psi}h_{\min},
\end{eqnarray*}$$that is
 $$\begin{eqnarray*}
&&(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}(\bar{h}^{1+\gamma n\psi}-h_{\min}^{1+\gamma n\psi}).
\end{eqnarray*}$$
$$\begin{eqnarray*}
&&(1+\gamma n\psi) \int _{h_{\min}}^{\bar{h}}(z+h^{\ast}(z))^{\gamma n\psi}dz \nonumber\\
&&\quad \ge 2^{\gamma n\psi}(\bar{h}^{1+\gamma n\psi}-h_{\min}^{1+\gamma n\psi}).
\end{eqnarray*}$$This inequality holds since the RHS equals the LHS for ![]() $\bar{h}=h_{\min},$ while differentiation shows that the LHS increases faster with
$\bar{h}=h_{\min},$ while differentiation shows that the LHS increases faster with ![]() $\bar{h}$ than the RHS. Therefore, (82) holds for the highest λ and for
$\bar{h}$ than the RHS. Therefore, (82) holds for the highest λ and for ![]() $\bar{h},$ i.e. for any equilibrium λ and all
$\bar{h},$ i.e. for any equilibrium λ and all ![]() $h\ge \bar{h}.$ Thus single women prefer the SATC equilibrium as well.
$h\ge \bar{h}.$ Thus single women prefer the SATC equilibrium as well.
We now turn to married men. Using the same steps as for married women, we get that they prefer the Victorian equilibrium iff
 $$\begin{equation*}
(1+\gamma n\psi) \int _{h_{\min}}^{h^{\ast -1}(h^{\ast})}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \ge 2^{\gamma n\psi}(\lambda -h^{\ast 1+\gamma n\psi})+(h^{\ast -1}(h^{\ast})+h^{\ast})^{1+\gamma n\psi}.
\end{equation*}$$
$$\begin{equation*}
(1+\gamma n\psi) \int _{h_{\min}}^{h^{\ast -1}(h^{\ast})}(z+h^{\ast}(z))^{\gamma n\psi}dz+\mu \ge 2^{\gamma n\psi}(\lambda -h^{\ast 1+\gamma n\psi})+(h^{\ast -1}(h^{\ast})+h^{\ast})^{1+\gamma n\psi}.
\end{equation*}$$ Again, this holds for all equilibrium values of λ if it holds for the largest one, ![]() $\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.$ Substituting, we get
$\lambda =\left( 1-2^{-\gamma n\psi}\tilde{h}^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi}.$ Substituting, we get
where ϕ(h) ≡ (h + h*(h))1 + γnψ − 2γnψh*(h)1 + γnψ − (1 + γnψ)∫hh min(z + h*(z))γnψdz. Now, note that ϕ′(h) = h*′(h)(1 + γnψ)[(h + h*(h))γnψ − 2γnψh*(h)γnψ] ⩽ 0. Thus, (84) holds for all ![]() $h^{\ast}\ge \underline{h}^{\ast}$ iff it holds at
$h^{\ast}\ge \underline{h}^{\ast}$ iff it holds at ![]() $h^{\ast}=\underline{h}^{\ast},$ that is
$h^{\ast}=\underline{h}^{\ast},$ that is
Substituting (64), we see that this is equivalent to
As the RHS equates the LHS for ![]() $\underline{h}^{\ast}=h_{\min},$ and as the LHS increases more with
$\underline{h}^{\ast}=h_{\min},$ and as the LHS increases more with ![]() $\underline{h}^{\ast}$ than the RHS, this inequality clearly holds, which proves that married beta men are better-off in the sexually repressed Victorian equilibrium than in the SATC equilibrium regardless of λ.
$\underline{h}^{\ast}$ than the RHS, this inequality clearly holds, which proves that married beta men are better-off in the sexually repressed Victorian equilibrium than in the SATC equilibrium regardless of λ.
We finally consider the case of single men. Using (3) and (12) and substituting ![]() $\theta =\theta _{V}(h^{\ast},\lambda) =\frac{1}{2} (1+\lambda h^{\ast -(1+\gamma n\psi) }),$ then rearranging, we see that they prefer the Victorian equilibrium iff
$\theta =\theta _{V}(h^{\ast},\lambda) =\frac{1}{2} (1+\lambda h^{\ast -(1+\gamma n\psi) }),$ then rearranging, we see that they prefer the Victorian equilibrium iff
This holds for the maximum ![]() $\lambda,\left( 1-2^{-\gamma n\psi}\tilde{h} ^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},$ iff
$\lambda,\left( 1-2^{-\gamma n\psi}\tilde{h} ^{\gamma n\psi}h_{\min}^{-\gamma n\psi}\right) h_{\min}^{1+\gamma n\psi},$ iff
This is clearly satisfied since the RHS grows with h* and is equal to the LHS at h* = h min.
Therefore single men also prefer the Victorian outcome. This completes the proof of Proposition 10.
A.11. Proof of Proposition 11
First, note that a Victorian equilibrium among the alphas always exists. This is because the alpha’s marriage problem is identical to the beta’s except that k = 0 in this case: women marrying an alpha would access the same genetic material if they were single instead. Since Proposition 6 holds for k = 0, such an equilibrium exists.
Next, assume that at date t, all alpha agents have a human capital level lower than hLR max, α, and all beta agents have a human capital lower than hLR max, β.
Clearly, for generation t + 1, the human capital level of an alpha cannot exceed that of the alpha children of a couple such that the man is alpha and both spouses have human capital hLR max, α. This can be written as
By construction, the RHS is equal to hLR max, α. Therefore, all the alphas in generation t + 1 have a human capital which cannot exceed hLR max, α. As for the betas of that generation, they cannot do better than the beta children of an alpha couple with human capital hLR max, α:
Therefore, the property that h ⩽ hLR max, β for all the betas and h ⩽ hLR max, α for all the alphas will remain true across all generations, regardless of how the betas mate, as long as the conditions of Proposition 2 hold.
Assume that at date t, all agents have a human capital level larger than hLR min, β. We know that (18) is more likely to hold, the smaller h max and the larger h min. By assumption, (18) holds for h min = hLR min, β and h max = hLR max, β. By assumption, the distribution of the beta’s human capital at t is such that hLR min, β ⩽ h min, t ⩽ h max, t ⩽ hLR max, β. Therefore, (18) holds. Also, by virtue of (28), so does the condition ![]() $h_{\min} \ge \underline{h}(\beta) $ and therefore the conditions of Proposition 2. Hence, there exists a marriage market equilibrium at t which is Victorian for the betas. Furthermore, in generation t + 1, the lowest human capital level of a beta cannot exceed that of a beta offspring of a beta couple with human capital hLR min, β:
$h_{\min} \ge \underline{h}(\beta) $ and therefore the conditions of Proposition 2. Hence, there exists a marriage market equilibrium at t which is Victorian for the betas. Furthermore, in generation t + 1, the lowest human capital level of a beta cannot exceed that of a beta offspring of a beta couple with human capital hLR min, β:
Therefore, the property that h ⩾ hLR min, β still holds among the betas (and, a fortiori, among the alphasFootnote 42) of generation t + 1, implying that a Victorian equilibrium also exists for them. By induction, h ⩾ hLR min, β for all generations and a Victorian equilibrium exists at all dates. This proves claim (i) in Proposition 11.
Claim (ii) derives straightforwardly from the fact that all agents marry, so that a fraction ρt of children have alpha fathers.
Claim (iii) derives straightforwardly from aggregating log human capital among all offsprings of types alpha and beta.
A.12. Proof of Proposition 12
The proof is straightforward from (27), (29), (30) and from noting that the expected human capital of the betas evolves according to
Finally note that (31) is equivalent to
and compare with (26).
A.13. Proof of Proposition 13
The required inequality holds for married women, since they get a higher quality beta husband in the SATC assignment than in the Victorian one. Let us thus focus on unmarried ones. In the Victorian assignment, their average offspring log human capital would be equal to
In the SATC equilibrium, we get
The inequality holds iff
This condition is equivalent to ek ⩾ 2γnψ, i.e to (86) being violated. We can show that this is a necessary condition for an SATC equilibrium to exist. Going back to the proof of Proposition 5, part E, we can see that (72) is a necessary condition for an SATC equilibrium. If it does not hold, we can show that women slightly above ![]() $\bar{h}$ can profitably underbid the women at
$\bar{h}$ can profitably underbid the women at ![]() $h=\bar{h},$ by just inverting the reasoning in part E and (72) clearly implies that ek ⩾ 2γnψ.
$h=\bar{h},$ by just inverting the reasoning in part E and (72) clearly implies that ek ⩾ 2γnψ.