Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cheung, Lawrence C.
Pastouchenko, Nikolai N.
Mani, Ramani
and
Paliath, Umesh
2015.
Fine-Scale Turbulent Noise Predictions from Non-Axisymmetric Jets.
International Journal of Aeroacoustics,
Vol. 14,
Issue. 3-4,
p.
457.
Clark di Leoni, Patricio
and
Mininni, Pablo D.
2016.
Quantifying resonant and near-resonant interactions in rotating turbulence.
Journal of Fluid Mechanics,
Vol. 809,
Issue. ,
p.
821.
Valente, P. C.
da Silva, C. B.
and
Pinho, F. T.
2016.
Energy spectra in elasto-inertial turbulence.
Physics of Fluids,
Vol. 28,
Issue. 7,
McKeon, B. J.
2017.
The engine behind (wall) turbulence: perspectives on scale interactions.
Journal of Fluid Mechanics,
Vol. 817,
Issue. ,
Wood, H.G.
Roman, A.
and
Hanna, J.A.
2018.
The saturation bifurcation in coupled oscillators.
Physics Letters A,
Vol. 382,
Issue. 30,
p.
1968.
Schmidt, Oliver T.
2020.
Bispectral mode decomposition of nonlinear flows.
Nonlinear Dynamics,
Vol. 102,
Issue. 4,
p.
2479.
Zhou, Ye
2021.
Turbulence theories and statistical closure approaches.
Physics Reports,
Vol. 935,
Issue. ,
p.
1.
Cheung, Lawrence C.
and
Zaki, Tamer A.
2023.
An eigen-representation of the Navier–Stokes equations.
Journal of Computational and Applied Mathematics,
Vol. 423,
Issue. ,
p.
114921.
Akhavan-Safaei, Ali
and
Zayernouri, Mohsen
2023.
A non-local spectral transfer model and new scaling law for scalar turbulence.
Journal of Fluid Mechanics,
Vol. 956,
Issue. ,
Blanco, Diego C.P.
Hanifi, Ardeshir
Henningson, Dan S.
and
Cavalieri, André V.G.
2024.
Linear and nonlinear receptivity mechanisms in boundary layers subject to free-stream turbulence.
Journal of Fluid Mechanics,
Vol. 979,
Issue. ,
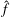 $\hat{f}$ and
$\hat{f}$ and 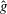 $\hat{g}$. An exact, tractable representation of the nonlinear terms in spectral space is introduced, and relies on the definition and manipulation of a combination matrix. A spectral energy equation is derived where the nonlinear triad interactions are expressed using the combination matrix. The formulation is applied to the problem of homogeneous, isotropic turbulence. By finding the solution in an appropriate canonical basis, the energy spectrum in the inertial range
$\hat{g}$. An exact, tractable representation of the nonlinear terms in spectral space is introduced, and relies on the definition and manipulation of a combination matrix. A spectral energy equation is derived where the nonlinear triad interactions are expressed using the combination matrix. The formulation is applied to the problem of homogeneous, isotropic turbulence. By finding the solution in an appropriate canonical basis, the energy spectrum in the inertial range 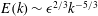 $E(k)\sim \epsilon ^{2/3}k^{-5/3}$ is derived from the Navier–Stokes equations without invoking dimensional scaling arguments.
$E(k)\sim \epsilon ^{2/3}k^{-5/3}$ is derived from the Navier–Stokes equations without invoking dimensional scaling arguments.