No CrossRef data available.
Article contents
An experimental and numerical study of the resonant flow between a hull and a wall
Published online by Cambridge University Press: 11 November 2021
Abstract
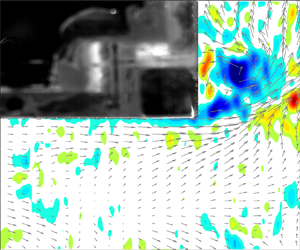
The wave-induced resonant flow in a narrow gap between a stationary hull and a vertical wall is studied experimentally and numerically. Vortex shedding from the sharp bilge edge of the hull gives rise to a quadratically damped free surface response in the gap, where the damping coefficient is approximately independent of wave steepness and frequency. Particle image velocimetry and direct numerical simulations were used to characterise the shedding dynamics and explore the influence of discretisation in the measurements and computations. Secondary separation was identified as a particular feature which occurred at the hull bilge in these gap flows. This can result in the generation of a system with multiple vortical regions and asymmetries between the inflow and outflow. The shedding dynamics was found to exhibit a high degree of invariance to the amplitude in the gap and the spanwise position of the barge. The new measurements and the evaluation of numerical models of varying fidelity can assist in informing offshore operations such as the side by side offloading from floating liquefied natural gas facilities.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press


