Article contents
Analysis of degenerate mechanisms triggering finite-amplitude thermo-acoustic oscillations in annular combustors
Published online by Cambridge University Press: 24 October 2019
Abstract
A simplified model is introduced to study finite-amplitude thermo-acoustic oscillations in 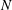 $N$-periodic annular combustion devices. Such oscillations yield undesirable effects and can be triggered by a positive feedback between heat-release and pressure fluctuations. The proposed model, comprising the governing equations linearized in the acoustic limit, and with each burner modelled as a one-dimensional system with acoustic damping and a compact heat source, is used to study the instability caused by cross-sector coupling. The coupling between the sectors is included by solving the one-dimensional acoustic jump conditions at the locations where the burners are coupled to the annular chambers of the combustion device. The analysis takes advantage of the block-circulant structure of the underlying stability equations to develop an efficient methodology to describe the onset of azimuthally synchronized motion. A modal analysis reveals the dominance of global instabilities (encompassing the large-scale dynamics of the entire system), while a non-modal analysis reveals a strong response to harmonic excitation at forcing frequencies far from the eigenfrequencies, when the overall system is linearly stable. In all presented cases, large-scale, azimuthally synchronized (coupled) motion is observed. The relevance of the non-modal response is further emphasized by demonstrating the subcritical nature of the system’s Hopf point via an asymptotic expansion of a nonlinear model representing the compact heat source within each burner.
$N$-periodic annular combustion devices. Such oscillations yield undesirable effects and can be triggered by a positive feedback between heat-release and pressure fluctuations. The proposed model, comprising the governing equations linearized in the acoustic limit, and with each burner modelled as a one-dimensional system with acoustic damping and a compact heat source, is used to study the instability caused by cross-sector coupling. The coupling between the sectors is included by solving the one-dimensional acoustic jump conditions at the locations where the burners are coupled to the annular chambers of the combustion device. The analysis takes advantage of the block-circulant structure of the underlying stability equations to develop an efficient methodology to describe the onset of azimuthally synchronized motion. A modal analysis reveals the dominance of global instabilities (encompassing the large-scale dynamics of the entire system), while a non-modal analysis reveals a strong response to harmonic excitation at forcing frequencies far from the eigenfrequencies, when the overall system is linearly stable. In all presented cases, large-scale, azimuthally synchronized (coupled) motion is observed. The relevance of the non-modal response is further emphasized by demonstrating the subcritical nature of the system’s Hopf point via an asymptotic expansion of a nonlinear model representing the compact heat source within each burner.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 6
- Cited by


