Article contents
Capillary levelling of immiscible bilayer films
Published online by Cambridge University Press: 25 January 2021
Abstract
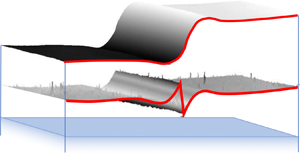
Flow in thin films is highly dependent on the boundary conditions. Here, we study the capillary levelling of thin bilayer films composed of two immiscible liquids. Specifically, a stepped polymer layer is placed atop another, flat polymer layer. The Laplace pressure gradient resulting from the curvature of the step induces flow in both layers, which dissipates the excess capillary energy stored in the stepped interface. The effect of different viscosity ratios between the bottom and top layers is investigated. We invoke a long-wave expansion of the low-Reynolds-number hydrodynamics to model the energy dissipation due to the coupled viscous flows in the two layers. Good agreement is found between the experiments and the model. Analysis of the latter further reveals an interesting double cross-over in time, from Poiseuille flow, to plug flow and finally to Couette flow. The cross-over time scales depend on the viscosity ratio between the two liquids, allowing for the dissipation mechanisms to be selected and finely tuned by varying this ratio.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 9
- Cited by



