Article contents
Detailed numerical investigation of the drop aerobreakup in the bag breakup regime
Published online by Cambridge University Press: 03 October 2023
Abstract
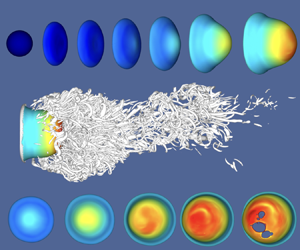
Aerobreakup of drops is a fundamental two-phase flow problem that is essential to many spray applications. A parametric numerical study was performed by varying the gas stream velocity, focusing on the regime of moderate Weber numbers, in which the drop deforms to a forward bag. When the bag is unstable, it inflates and disintegrates into small droplets. Detailed numerical simulations were conducted using the volume-of-fluid method on an adaptive octree mesh to investigate the aerobreakup dynamics. Grid-refinement studies show that converged three-dimensional simulation results for drop deformation and bag formation are achieved by the refinement level equivalent to 512 cells across the initial drop diameter. To resolve the thin liquid sheet when the bag inflates, the mesh is refined further to 2048 cells across the initial drop diameter. The simulation results for the drop length and radius were validated against previous experiments, and good agreement was achieved. The high-resolution results of drop morphological evolution were used to identify the different phases in the aerobreakup process, and to characterize the distinct flow features and dominant mechanisms in each phase. In the early time, the drop deformation and velocity are independent of the Weber number, and a new internal-flow deformation model, which respects this asymptotic limit, has been developed. The pressure and velocity fields around the drop were shown to better understand the internal flow and interfacial instability that dictate the drop deformation. Finally, the impact of drop deformation on the drop dynamics was discussed.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
Ling and Mahmood Supplementary Movie 1
Time evolutions of the drop surface and vortical structures for drop aerobreakup at We=12.0 and Re=2483. The color on the drop surface represents the interfacial velocity magnitude.
Ling and Mahmood Supplementary Movie 2
Time evolutions of the drop surface and vortical structures for drop aerobreakup at We=15.3 and Re=2800. The color on the drop surface represents the interfacial velocity magnitude.
- 10
- Cited by



