Article contents
Direct numerical simulation of turbulent flow through a ribbed square duct
Published online by Cambridge University Press: 06 August 2020
Abstract
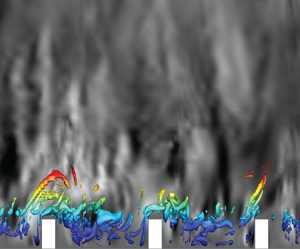
In this research turbulent flow in a ribbed square duct of different blockage ratios ( $Br=0.05$, 0.1 and 0.2) at a fixed Reynolds number of
$Br=0.05$, 0.1 and 0.2) at a fixed Reynolds number of  $Re_b=5600$ is studied using direct numerical simulation. The results are compared with those of a smooth duct flow. In contrast to the classical two-dimensional rib-roughened boundary-layer flow over a flat plate, the turbulence field is influenced by not only the rib elements but also the four duct sidewalls. The results detail out the three-dimensional effects of the sidewalls and ribs on flow statistics and structures. This study also aims at investigating the effect of blockage ratio on local non-equilibrium of turbulence, large- and small-scale flow anisotropy, and transport of turbulence kinetic energy. It is observed that as the rib height increases, the pressure near the windward face of the rib increases significantly, associated with an accelerated streamwise flow in the duct. Furthermore, an augmentation of the blockage ratio concurrently generates stronger turbulent secondary flow motions, resulting in larger magnitudes of Reynolds stresses near the rib crest. The secondary flow motions drastically alter the turbulent transport processes between the sidewall and duct centre, giving rise to high degrees of non-equilibrium states. The dynamics of coherent structures are studied by examining characteristics of the instantaneous velocity field, swirling strength, temporal auto-correlations, spatial two-point auto-correlations and velocity spectra. The results show that an increase of rib height significantly promotes the ejection and sweep events, which subsequently amplify the strength of vortical motions near the rib crest.
$Re_b=5600$ is studied using direct numerical simulation. The results are compared with those of a smooth duct flow. In contrast to the classical two-dimensional rib-roughened boundary-layer flow over a flat plate, the turbulence field is influenced by not only the rib elements but also the four duct sidewalls. The results detail out the three-dimensional effects of the sidewalls and ribs on flow statistics and structures. This study also aims at investigating the effect of blockage ratio on local non-equilibrium of turbulence, large- and small-scale flow anisotropy, and transport of turbulence kinetic energy. It is observed that as the rib height increases, the pressure near the windward face of the rib increases significantly, associated with an accelerated streamwise flow in the duct. Furthermore, an augmentation of the blockage ratio concurrently generates stronger turbulent secondary flow motions, resulting in larger magnitudes of Reynolds stresses near the rib crest. The secondary flow motions drastically alter the turbulent transport processes between the sidewall and duct centre, giving rise to high degrees of non-equilibrium states. The dynamics of coherent structures are studied by examining characteristics of the instantaneous velocity field, swirling strength, temporal auto-correlations, spatial two-point auto-correlations and velocity spectra. The results show that an increase of rib height significantly promotes the ejection and sweep events, which subsequently amplify the strength of vortical motions near the rib crest.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 28
- Cited by


