Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2025-01-01T09:16:29.187Z Has data issue: false hasContentIssue false
  • English
  • Français

Falling clouds of particles in vortical flows

Published online by Cambridge University Press:  10 December 2020

Benjamin Marchetti ,
Laurence Bergougnoux Open the ORCID record for Laurence Bergougnoux [Opens in a new window]  and
Elisabeth Guazzelli Open the ORCID record for Elisabeth Guazzelli [Opens in a new window]
Benjamin Marchetti
Affiliation:
Aix Marseille Université, CNRS, IUSTI, 13453Marseille, France
Laurence Bergougnoux*
Affiliation:
Aix Marseille Université, CNRS, IUSTI, 13453Marseille, France
Elisabeth Guazzelli
Affiliation:
Université de Paris, CNRS, Matière et Systèmes Complexes (MSC) UMR 7057, 75205Paris, France
*
†Email address for correspondence: laurence.bergougnoux@univ-amu.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

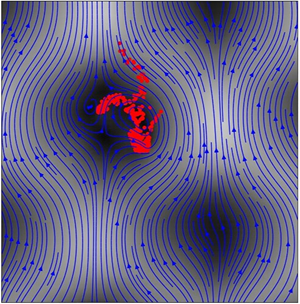
The coupling between particle–particle and particle–fluid interactions is examined by studying the sedimentation of clouds of spheres in a model cellular flow at a small but finite Reynolds number. The model flow consists of counter-rotating vortices and is aimed at capturing key features of the vortical effects on particles. The dynamics of clouds settling in this vortical flow is investigated through a comparison between experiments and point-particle simulations.

JFM classification

Complex Fluids: Suspensions Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 908 , 10 February 2021 , A30
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Akutina, Y., Revil-Baudard, T., Chauchat, J. & Eiff, O. 2020 Experimental evidence of settling retardation in a turbulence column. Phys. Rev. Fluids 5, 014303.CrossRefGoogle Scholar
Aliseda, A., Cartellier, A., Hainaux, F. & Lasheras, J. C. 2002 Effect of preferential concentration on the settling velocity of heavy particles in homogeneous isotropic turbulence. J. Fluid Mech. 468, 77–105.CrossRefGoogle Scholar
Balachandar, S. & Eaton, J. K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.CrossRefGoogle Scholar
Bergougnoux, L., Bouchet, G., Lopez, D. & Guazzelli, É. 2014 The motion of solid spherical particles falling in a cellular flow field at low Stokes number. Phys. Fluids 26, 093302.CrossRefGoogle Scholar
Blake, J. R. 1971 A note on the image system for a stokeslet in a no-slip boundary. Proc. Camb. Phil. Soc. 70, 303–310.CrossRefGoogle Scholar
Bosse, T., Kleiser, L., Härtel, C. & Meiburg, E. 2005 Numerical simulation of finite Reynolds number suspension drops settling under gravity. Phys. Fluids 17, 037101.CrossRefGoogle Scholar
Drótos, G., Monroy, P., Hernández-García, E. & López, C. 2019 Inhomogeneities and caustics in the sedimentation of noninertial particles in incompressible flows. Chaos 29, 013115.Google ScholarPubMed
Gatignol, R. 1983 The Faxen formulae for a rigid particle in an unsteady non-uniform Stokes flow. J. Méc. 2, 143–160.Google Scholar
Good, G. H., Ireland, P. J., Bewley, G. P., Bodenschatz, E., Collins, L. R. & Warhaft, Z. 2014 Settling regimes of inertial particles in isotropic turbulence. J. Fluid Mech. 759, R3.CrossRefGoogle Scholar
Guazzelli, E. & Hinch, J. 2011 Fluctuations and instability in sedimentation. Annu. Rev. Fluid Mech. 43, 97–116.CrossRefGoogle Scholar
Hadamard, J. 1911 Mouvement permanent lent d'une sphère liquide et visqueuse dans un liquide visqueux. C. R. Acad. Sci. Paris 152, 1735–1738.Google Scholar
Huck, P. D., Bateson, C., Volk, R., Cartellier, A., Bourgoin, M. & Aliseda, A. 2018 The role of collective effects on settling velocity enhancement for inertial particles in turbulence. J. Fluid Mech. 846, 1059–1075.CrossRefGoogle Scholar
Lopez, D. & Guazzelli, É. 2017 Inertial effects on fibers settling in a vortical flow. Phys. Rev. Fluids 2, 024306.CrossRefGoogle Scholar
Lovalenti, P. M. & Brady, J. F. 1993 The hydrodynamic force on a rigid particle undergoing arbitrary time-dependent motion at small Reynolds number. J. Fluid Mech. 256, 561–605.CrossRefGoogle Scholar
Machu, G., Meile, W., Nitsche, L. C. & Schaflinger, U. 2001 Coalescence, torus formation and breakup of sedimenting drops: experiments and computer simulations. J. Fluid Mech. 447, 299–336.CrossRefGoogle Scholar
Maxey, M. R. 1987 The motion of small spherical-particles in a cellular-flow field. Phys. Fluids 30, 1915–1928.CrossRefGoogle Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26, 883–889.CrossRefGoogle Scholar
Mei, R. 1994 Effect of turbulence on the particle settling velocity in the nonlinear drag range. Intl J. Multiphase Flow 20, 273–284.CrossRefGoogle Scholar
Metzger, B., Nicolas, M. & Guazzelli, É. 2007 Falling clouds of particles in viscous fluids. J. Fluid Mech. 580, 283–301.Google Scholar
Meunier, P. & Leweke, T. 2003 Analysis and treatment of errors due to high velocity gradients in particle image velocimetry. Exp. Fluids 35 (5), 408–421.Google Scholar
Myłyk, A., Meile, W., Brenn, G. & Ekiel-Jeżewska, M. L. 2011 Break-up of suspension drops settling under gravity in a viscous fluid close to a vertical wall. Phys. Fluids 23, 063302.CrossRefGoogle Scholar
Nielsen, P. 1993 Turbulence effects on the settling of suspended particles. J. Sedim. Petrol. 63, 835–838.Google Scholar
Nitsche, J. & Batchelor, G. 1997 Break-up of a falling drop containing dispersed particles. J. Fluid Mech. 340, 161–175.CrossRefGoogle Scholar
Noh, Y. & Fernando, H. J. S. 1993 The transition in the sedimentation pattern of a particle cloud. Phys. Fluids 5, 3049–3055.CrossRefGoogle Scholar
Pignatel, F., Nicolas, M. & Guazzelli, É. 2011 A falling cloud of particles at a small but finite Reynolds number. J. Fluid Mech. 671, 34–51.CrossRefGoogle Scholar
Reeks, M. W. 2014 Transport, mixing and agglomeration of particles in turbulent flows. J. Phys.: Conf. Ser. 530, 012003.Google Scholar
Rybczyński, W. 1911 Über die fortschreitende Bewegung einer flüssigen Kugel in einem zähen Medium. Bull. Acad. Sci. Cracovie A 1, 40–46.Google Scholar
Stommel, H. 1949 Trajectories of small bodies sinking slowly through convection cells. J. Mar. Res. 8, 24–29.Google Scholar
Subramanian, G. & Koch, D. L. 2008 Evolution of clusters of sedimenting low-Reynolds-number particles with Oseen interactions. J. Fluid Mech. 603, 63–100.CrossRefGoogle Scholar
Toschi, F. & Bodenschatz, E. 2009 Lagrangian properties of particles in turbulence. Annu. Rev. Fluid Mech. 41, 375–404.CrossRefGoogle Scholar
Wang, L. P. & Maxey, M. R. 1993 Settling velocity and concentration distribution of heavy-particles in homogeneous isotropic turbulence. J. Fluid Mech. 256, 27–68.CrossRefGoogle Scholar

Marchetti et al. supplementary movie 1

See pdf file for movie caption

Download Marchetti et al. supplementary movie 1(Video)
Video 792.5 KB

Marchetti et al. supplementary movie 2

See pdf for movie caption

Download Marchetti et al. supplementary movie 2(Video)
Video 1.3 MB

Marchetti et al. supplementary movie 3

See pdf file for movie caption

Download Marchetti et al. supplementary movie 3(Video)
Video 831 KB

Marchetti et al. supplementary movie 4

See pdf file for movie caption

Download Marchetti et al. supplementary movie 4(Video)
Video 5.4 MB

Marchetti et al. supplementary movie 5

See pdf file for movie caption

Download Marchetti et al. supplementary movie 5(Video)
Video 2.8 MB

Marchetti et al. supplementary movie 6

See pdf file for movie caption

Download Marchetti et al. supplementary movie 6(Video)
Video 3.6 MB

Marchetti et al. supplementary movie 7

See pdf file for movie caption

Download Marchetti et al. supplementary movie 7(Video)
Video 585 KB
Supplementary material: PDF

Marchetti et al. supplementary material

Captions for movies 1-7

Download Marchetti et al. supplementary material(PDF)
PDF 49.7 KB