Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-28T19:33:33.342Z Has data issue: false hasContentIssue false
  • English
  • Français

Flame wrinkle destruction processes in harmonically forced, turbulent premixed flames

Published online by Cambridge University Press:  19 March 2013

Dong-Hyuk Shin  and
Timothy Lieuwen
Dong-Hyuk Shin
Affiliation:
School of Aerospace Engineering, Georgia Institute of Technology, Atlanta, GA 30332, USA
Timothy Lieuwen*
Affiliation:
School of Aerospace Engineering, Georgia Institute of Technology, Atlanta, GA 30332, USA
*
†Email address for correspondence: tim.lieuwen@aerospace.gatech.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper describes analyses of the nonlinear dynamics of harmonically forced, turbulent premixed flames. A key objective of this work is to analyse the ensemble-averaged dynamics of the flame front position, 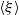$\langle \xi \rangle $, excited by harmonic forcing of amplitude $\varepsilon $, in the presence of stochastic flow fluctuations of amplitude 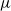$\mu $. Low-amplitude and/or near-field effects are quantified by a third-order perturbation analysis, while the more general case is analysed computationally by solving the three-dimensional level-set equation, extracting the instantaneous flame position, and ensemble averaging the results. We show that different mechanisms contribute to smoothing of flame wrinkles, manifested as progressive decay in the magnitude of $\langle \xi \rangle $. Near the flame holder, random phase jitter, associated with stochastic velocity fluctuations tangential to the flame, is dominant. Farther downstream, propagation of the ensemble-averaged front normal to itself at the time-averaged turbulent burning velocity, 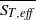$ \overline{{S}_{T, eff} } $, leads to destruction of wrinkles, analogous to the laminar case, an effect that scales with 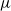$\mu $. A second, new result is the demonstration that the ensemble-averaged turbulent burning velocity, ${S}_{T, eff} (s, t)$, is modulated by the harmonic forcing, i.e. 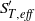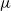${S}_{T, eff} (s, t)= \overline{{S}_{T, eff} (s)} + { S}_{T, eff}^{\prime } (s, t)$, where ${ S}_{T, eff}^{\prime } $ has an inverse dependence upon the instantaneous, ensemble averaged-flame curvature, an effect that scales with $\varepsilon $ and $\mu $. We show that this curvature dependence follows from basic application of Huygens propagation to flames with stochastic wrinkling superimposed upon base curvature. This effect also leads to smoothing of flame wrinkles and is analogous to stretch processes in positive-Markstein-length, laminar flames.

JFM classification

Reacting Flows: Combustion Reacting Flows: Reacting Flows Reacting Flows: Turbulent reacting flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 721 , 25 April 2013 , pp. 484 - 513
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdel-Gayed, R., Bradley, D. & Lawes, M. 1987 Turbulent burning velocities: a general correlation in terms of straining rates. Proc. R. Soc. Lond. A 414, 389–413.Google Scholar
Aldredge, R. C. & Williams, F. A. 1991 Influence of wrinkled premixed-flame dynamics on large-scale, low-intensity turbulent-flow. J. Fluid Mech. 228, 487–511.Google Scholar
Ayache, S., Dawson, J. R., Triantafyllidis, A., Balachandran, R. & Mastorakos, E. 2010 Experiments and large-eddy simulations of acoustically forced bluff-body flows. Intl J. Heat Fluid Flow 31, 754–766.Google Scholar
Bendat, J. S. & Piersol, A. G. 2011 Random Data: Analysis and Measurement Procedures. Wiley.Google Scholar
Cardell, G. S. 1993 Flow Past a Circular Cylinder with a Permeable Splitter Plate. California Institute of Technology.Google Scholar
Coats, C. M. 1996 Coherent structures in combustion. Prog. Energy Combust. Sci. 22, 427–509.Google Scholar
Creta, F., Fogla, N. & Matalon, M. 2011 Turbulent propagation of premixed flames in the presence of Darrieus–Landau instability. Combust. Theor. Model. 15, 267–298.Google Scholar
Creta, F. & Matalon, M. 2011 Propagation of wrinkled turbulent flames in the context of hydrodynamic theory. J. Fluid Mech. 680, 225–264.Google Scholar
Dally, B. B., Riesmeier, E. & Peters, N. 2004 Effect of fuel mixture on moderate and intense low oxygen dilution combustion. Combust. Flame 137, 418–431.Google Scholar
Dowling, A. P. 1999 A kinematic model of a ducted flame. J. Fluid Mech. 394, 51–72.Google Scholar
Driscoll, J. F. 2008 Turbulent premixed combustion: flamelet structure and its effect on turbulent burning velocities. Prog. Energy Combust. Sci. 34, 91–134.Google Scholar
Dupont, T. F. & Liu, Y. J. 2007 Back and forth error compensation and correction methods for semi-Lagrangian schemes with application to level set interface computations. Maths Comput. 76, 647–668.Google Scholar
Evans, L. C. 1997 Partial Differential Equations. American Mathematical Society.Google Scholar
Frenkiel, F. N. & Klebanoff, P. S. 1967 Higher-order correlations in a turbulent field. Phys. Fluids 10, 507–520.Google Scholar
Groff, E. G. 1987 An experimental evaluation of an entrainment flame-propagation model. Combust. Flame 67, 153–162.CrossRefGoogle Scholar
Hemchandra, S. & Lieuwen, T. 2010 Local consumption speed of turbulent premixed flames – an analysis of ‘memory effects’. Combust. Flame 157, 955–965.Google Scholar
Hemchandra, S., Peters, N. & Lieuwen, T. 2011 Heat release response of acoustically forced turbulent premixed flames-role of kinematic restoration. Proc. Combust. Inst. 33, 1609–1617.Google Scholar
Hinze, J. O. 1975 Turbulence. McGraw-Hill.Google Scholar
Huang, Y., Ratner, A., Yang, V. & Lu, T. 2009 Experimental investigation of thermoacoustic coupling for low-swirl lean premixed flames. J. Propul. Power 25, 365–373.CrossRefGoogle Scholar
Huang, Y. & Yang, V. 2009 Dynamics and stability of lean-premixed swirl-stabilized combustion. Prog. Energy Combust. Sci. 35, 293–364.Google Scholar
Huygens, C. 1962 Treatise on Light. Dover.Google Scholar
Karimi, N., Brear, M. J., Jin, S.-H. & Monty, J. P. 2009 Linear and nonlinear forced response of a conical, ducted, laminar premixed flame. Combust. Flame 156, 2201–2212.Google Scholar
Kim, K. T. & Hochgreb, S. 2011 The nonlinear heat release response of stratified lean-premixed flames to acoustic velocity oscillations. Combust. Flame 158, 2482–2499.Google Scholar
Kuramoto, Y. & Tsuzuki, T. 1976 Persistent propagation of concentration waves in dissipative media far from thermal equilibrium. Prog. Theor. Phys. 55, 356–369.Google Scholar
Law, C. K. & Sung, C. J. 2000 Structure, aerodynamics, and geometry of premixed flamelets. Prog. Energy Combust. Sci. 26, 459–505.Google Scholar
Lee, D. H. & Lieuwen, T. C. 2003 Premixed flame kinematics in a longitudinal acoustic field. J. Propul. Power 19, 837–846.Google Scholar
Lee, J. G. & Santavicca, D. A. 2003 Experimental diagnostics for the study of combustion instabilities in lean premixed combustors. J. Propul. Power 19, 735–750.CrossRefGoogle Scholar
Lieuwen, T. 2003 Modeling premixed combustion-acoustic wave interactions: a review. J. Propul. Power 19, 765–781.Google Scholar
Lieuwen, T. C. & Yang, V. 2005 Combustion Instabilities in Gas Turbine Engines: Operational Experience, Fundamental Mechanisms and Modeling. AIAA.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2002 Turbulent flame speed and thickness: phenomenology, evaluation, and application in multi-dimensional simulations. Prog. Energy Combust. Sci. 28, 1–74.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2004 Application of the Markstein number concept to curved turbulent flames. Combust. Sci. Technol. 176, 331–358.Google Scholar
Lipatnikov, A. & Chomiak, J. 2007 Global stretch effects in premixed turbulent combustion. Proc. Combust. Inst. 31, 1361–1368.Google Scholar
Lipatnikov, A. N. & Sathiah, P. 2005 Effects of turbulent flame development on thermoacoustic oscillations. Combust. Flame 142, 130–139.Google Scholar
Matalon, M. 1983 On flame stretch. Combust. Sci. Technol. 31, 169–181.Google Scholar
Matalon, M. & Matkowsky, B. J. 1982 Flames as gas-dynamic discontinuities. J. Fluid Mech. 124, 239–259.Google Scholar
McManus, K. R., Poinsot, T. & Candel, S. M. 1993 A review of active control of combustion instabilities. Prog. Energy Combust. Sci. 19, 1–29.Google Scholar
Moureau, V., Fiorina, B. & Pitsch, H. 2009 A level set formulation for premixed combustion LES considering the turbulent flame structure. Combust. Flame 156, 801–812.Google Scholar
Osher, S. & Fedkiw, R. P. 2003 Level Set Methods and Dynamic Implicit Surfaces. Springer.Google Scholar
Palies, P., Schuller, T., Durox, D. & Candel, S. 2011 Modeling of premixed swirling flames transfer functions. Proc. Combust. Inst. 33, 2967–2974.CrossRefGoogle Scholar
Peng, D. P., Merriman, B., Osher, S., Zhao, H. K. & Kang, M. J. 1999 A PDE-based fast local level set method. J. Comput. Phys. 155, 410–438.CrossRefGoogle Scholar
Peter, S. 2006 The numerical approximation of a delta function with application to level set methods. J. Comput. Phys. 211, 77–90.Google Scholar
Petersen, R. E. & Emmons, H. W. 1961 Stability of laminar flames. Phys. Fluids 4, 456–464.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Prudnikov, A. 1964 Combustion of homogeneous fuel-air mixtures in turbulent flows. In Physical Basis of Processes in Combustion Chambers of Airbreathing Engines (ed. Raushenbakh, B. V., Belyi, S. A., Bespalov, I. V., Borodachev, V. Y., Volynskii, M. S. & Prudnikov, A. G.). pp. 255–347. Mashinostroenie.Google Scholar
Rastigejev, Y. & Matalon, M. 2006 Numerical simulation of flames as gas-dynamic discontinuities. Combust. Theor. Model. 10, 459–481.CrossRefGoogle Scholar
Searby, G. & Rochwerger, D. 1991 A parametric acoustic instability in premixed flames. J. Fluid Mech. 231, 529–543.Google Scholar
Sethian, J. A. 1999 Level Set Methods and Fast Marching Methods: Evolving Interfaces in Computational Geometry, Fluid Mechanics, Computer Vision, and Materials Science. Cambridge University Press.Google Scholar
Shanbhogue, S. 2008 Dynamics of Perturbed Exothermic Bluff-body Flow Fields. Georgia Institute of Technology.Google Scholar
Shanbhogue, S., Shin, D. H., Hemchandra, S., Plaks, D. & Lieuwen, T. 2009a Flame sheet dynamics of bluff-body stabilized flames during longitudinal acoustic forcing. Proc. Combust. Inst. 32, 1787–1794.Google Scholar
Shanbhogue, S. J., Seelhorst, M. & Lieuwen, T. 2009b Vortex phase-jitter in acoustically excited bluff body flames. Intl J. Spray Combust. Dyn. 1, 365–387.Google Scholar
Shin, D. H. 2012 Premixed Flame Kinematics in a Harmonically Oscillating Velocity Field. Georgia Institute of Technology.Google Scholar
Shin, D.-H. & Lieuwen, T. 2012 Flame wrinkle destruction processes in harmonically forced, laminar premixed flames. Combust. Flame 159, 3312–3322.Google Scholar
Shin, D. H., Plaks, D. V., Lieuwen, T., Mondragon, U. M., Brown, C. T. & McDonell, V. G. 2011 Dynamics of a longitudinally forced, bluff body stabilized flame. J. Propul. Power 27, 105–116.Google Scholar
Sivashinsky, G. I. 1977 Nonlinear analysis of hydrodynamic instability in laminar flames – I. Derivation of basic equations. Acta Astron. 4, 1177–1206.Google Scholar
Thumuluru, S. K. 2010 Effect of Harmonic Forcing on Turbulent Flame Properties. Georgia Institute of Technology.Google Scholar
Tsuji, H., Gupta, A. K., Hasegawa, T., Katsuki, M., Kishimoto, K. & Morita, M. 2002 High Temperature Air Combustion: From Energy Conservation to Pollution Reduction. CRC.Google Scholar
Wang, H. Y., Law, C. K. & Lieuwen, T. 2009 Linear response of stretch-affected premixed flames to flow oscillations. Combust. Flame 156, 889–895.Google Scholar
Williams, F. A. 1985 Combustion Theory. Addison-Wesley.Google Scholar
Zimont, V. 1977 To computations of turbulent combustion of partially premixed gases. In Chemical Physics of Combustion and Explosion Processes. Combustion of Multi-Phase and Gas Systems, pp. 77–80 USSR Academy of Science.Google Scholar
Zimont, V. & Lipatnikov, A. 1995 A numerical model of premixed turbulent combustion of gases. Chem. Phys. Rep. 14, 993–1025.Google Scholar