Article contents
Fluid dynamics of frozen precipitation at the air–water interface
Published online by Cambridge University Press: 29 December 2021
Abstract
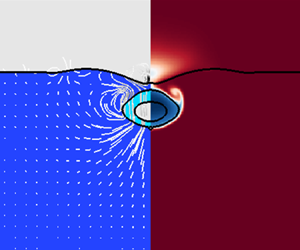
Precipitation in the forms of snow, hail, and rain plays a critical role in the exchange of mass, momentum and heat at the surfaces of lakes and seas. However, the microphysics of these interactions are not well understood. Motivated by recent observations, we study the physics of the impact of a single frozen canonical particle, such as snow and hail, onto the surface of a liquid bath using a numerical model. The descent, melting, bubble formation and thermal transport characteristics of this system are examined. Three distinct response regimes, namely particle impact, ice melting and vortex ring descent, have been identified and characterized. The melting rate and air content of the snow particle are found to be leading factors affecting the formation of a coherent vortex ring, the vertical descent of melted liquid and the vortex-induced transportation of the released gas bubble to lower depths. It is found that the water temperature can substantially alter the rate of phase change and subsequent flow and thermal transport, while the particle temperature has minimal effect on the process. Finally, the effects of the Reynolds, Weber and Stefan numbers are examined and it is shown that the Reynolds number modifies the strength of the vortex ring and induces the most significant effect on the flow dynamics of the snow particle. Also, the change of Weber number primarily alters the initial phases of snow–bath interaction while modifying the Stefan number of the snow particle essentially determines the system response in its later stages.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 3
- Cited by


