Article contents
Interfaces of uniform momentum zones in turbulent boundary layers
Published online by Cambridge University Press: 12 May 2017
Abstract
In this paper we examine the characteristics of the interfaces that demarcate regions of relatively uniform streamwise momentum in turbulent boundary layers. The analysis utilises particle image velocimetry databases that span more than an order of magnitude of friction Reynolds number ( 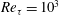 $Re_{\unicode[STIX]{x1D70F}}=10^{3}$ –
$Re_{\unicode[STIX]{x1D70F}}=10^{3}$ –  $10^{4}$ ), enabling us to provide a detailed description of the interfacial layers as a function of Reynolds number. As reported by Adrian et al. (J. Fluid Mech., vol. 422, 2000, pp. 1–54), these interfaces appear as persistent regions of strong shear with distinct patches of vorticity consistent with a packet-like structure. Here, however, we treat these interfaces as continuous lines, thus averaging the properties of the vortical patches, and find that their geometry is highly contorted and exhibits self-similarity across a wide range of scales. Specifically, the lengths of the edges of uniform momentum zones exhibit a power-law behaviour with a fractal scaling that has a constant exponent across the boundary layer, while the topmost edge or the turbulent/non-turbulent interface shows a sudden increase in the exponent. The accompanying sharp changes in velocity that occur at these edges are found to change in magnitude as a function of wall-normal height, being larger closer to the wall. Further, a Reynolds number invariance is exhibited when the magnitude of the step-like changes in velocity is scaled by the skin-friction velocity, meanwhile, the width across which it occurs is shown to be of the order of the Taylor microscale. Based on these quantitative measures, the Reynolds number scaling observed and the persistent presence of sharp changes in momentum in turbulent boundary layers, a simple model is used to reconstruct the mean velocity profile. Insight gained from the model enhances our understanding of how instantaneous phenomena (such as a zonal-like structural arrangement) manifests in the averaged flow statistics and confirms that the instantaneous momentum in a turbulent boundary layer appears to mainly consist of a step-like profile as a function of wall-normal distance.
$10^{4}$ ), enabling us to provide a detailed description of the interfacial layers as a function of Reynolds number. As reported by Adrian et al. (J. Fluid Mech., vol. 422, 2000, pp. 1–54), these interfaces appear as persistent regions of strong shear with distinct patches of vorticity consistent with a packet-like structure. Here, however, we treat these interfaces as continuous lines, thus averaging the properties of the vortical patches, and find that their geometry is highly contorted and exhibits self-similarity across a wide range of scales. Specifically, the lengths of the edges of uniform momentum zones exhibit a power-law behaviour with a fractal scaling that has a constant exponent across the boundary layer, while the topmost edge or the turbulent/non-turbulent interface shows a sudden increase in the exponent. The accompanying sharp changes in velocity that occur at these edges are found to change in magnitude as a function of wall-normal height, being larger closer to the wall. Further, a Reynolds number invariance is exhibited when the magnitude of the step-like changes in velocity is scaled by the skin-friction velocity, meanwhile, the width across which it occurs is shown to be of the order of the Taylor microscale. Based on these quantitative measures, the Reynolds number scaling observed and the persistent presence of sharp changes in momentum in turbulent boundary layers, a simple model is used to reconstruct the mean velocity profile. Insight gained from the model enhances our understanding of how instantaneous phenomena (such as a zonal-like structural arrangement) manifests in the averaged flow statistics and confirms that the instantaneous momentum in a turbulent boundary layer appears to mainly consist of a step-like profile as a function of wall-normal distance.
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 67
- Cited by


