Article contents
Investigation of high-pressure turbulent jets using direct numerical simulation
Published online by Cambridge University Press: 13 July 2021
Abstract
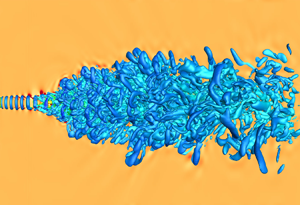
Direct numerical simulations of free round jets at a Reynolds number ( $Re_{D}$) of
$Re_{D}$) of  $5000$, based on jet diameter (
$5000$, based on jet diameter ( $D$) and jet-exit bulk velocity (
$D$) and jet-exit bulk velocity ( $U_{e}$), are performed to study jet turbulence characteristics at supercritical pressures. The jet consists of nitrogen (
$U_{e}$), are performed to study jet turbulence characteristics at supercritical pressures. The jet consists of nitrogen ( $\mathrm {N}_{2}$) that is injected into
$\mathrm {N}_{2}$) that is injected into  $\mathrm {N}_{2}$ at the same temperature. To understand turbulent mixing, a passive scalar is transported with the flow at unity Schmidt number. Two sets of inflow conditions that model jets issuing from either a smooth contraction nozzle (laminar inflow) or a long pipe nozzle (turbulent inflow) are considered. By changing one parameter at a time, the simulations examine the jet-flow sensitivity to the thermodynamic condition (characterized in terms of the compressibility factor (
$\mathrm {N}_{2}$ at the same temperature. To understand turbulent mixing, a passive scalar is transported with the flow at unity Schmidt number. Two sets of inflow conditions that model jets issuing from either a smooth contraction nozzle (laminar inflow) or a long pipe nozzle (turbulent inflow) are considered. By changing one parameter at a time, the simulations examine the jet-flow sensitivity to the thermodynamic condition (characterized in terms of the compressibility factor ( $Z$) and the normalized isothermal compressibility), inflow condition and ambient pressure (
$Z$) and the normalized isothermal compressibility), inflow condition and ambient pressure ( $p_{\infty }$) spanning perfect- to real-gas conditions. The inflow affects flow statistics in the near field (containing the potential core closure and the transition region) as well as further downstream (containing fully developed flow with self-similar statistics) at both atmospheric and supercritical
$p_{\infty }$) spanning perfect- to real-gas conditions. The inflow affects flow statistics in the near field (containing the potential core closure and the transition region) as well as further downstream (containing fully developed flow with self-similar statistics) at both atmospheric and supercritical  $p_{\infty }$. The sensitivity to inflow is larger in the transition region, where the laminar-inflow jets exhibit dominant coherent structures that produce higher mean strain rates and higher turbulent kinetic energy than in turbulent-inflow jets. Decreasing
$p_{\infty }$. The sensitivity to inflow is larger in the transition region, where the laminar-inflow jets exhibit dominant coherent structures that produce higher mean strain rates and higher turbulent kinetic energy than in turbulent-inflow jets. Decreasing  $Z$ at a fixed supercritical
$Z$ at a fixed supercritical  $p_{\infty }$ enhances pressure and density fluctuations (non-dimensionalized by local mean pressure and density, respectively), but the effect on velocity fluctuations depends also on the local flow dynamics. When
$p_{\infty }$ enhances pressure and density fluctuations (non-dimensionalized by local mean pressure and density, respectively), but the effect on velocity fluctuations depends also on the local flow dynamics. When  $Z$ is reduced, large mean strain rates in the transition region of laminar-inflow jets significantly enhance velocity fluctuations (non-dimensionalized by local mean velocity) and scalar mixing, whereas the effects are minimal in jets from turbulent inflow.
$Z$ is reduced, large mean strain rates in the transition region of laminar-inflow jets significantly enhance velocity fluctuations (non-dimensionalized by local mean velocity) and scalar mixing, whereas the effects are minimal in jets from turbulent inflow.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
Footnotes
Present address: CCS-2, Los Alamos National Laboratory, Los Alamos, NM 87544, USA.
References
REFERENCES
- 10
- Cited by


