Published online by Cambridge University Press: 07 January 2019
We experimentally investigate the temporal decay of homogeneous anisotropic turbulence, monitoring the evolution of velocity fluctuations, dissipation and turbulent length scales over time. We employ an apparatus in which two facing random jet arrays of water pumps generate turbulence with negligible mean flow and shear over a volume that is much larger than the initial characteristic turbulent large scale of the flow. The Reynolds number based on the Taylor microscale for forced turbulence is 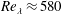 $Re_{\unicode[STIX]{x1D706}}\approx 580$ and the axial-to-radial ratio of the root mean square velocity fluctuations is
$Re_{\unicode[STIX]{x1D706}}\approx 580$ and the axial-to-radial ratio of the root mean square velocity fluctuations is 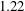 $1.22$. Two velocity components are measured by particle image velocimetry at the symmetry plane of the water tank. Measurements are taken for both ‘stationary’ forced turbulence and natural decaying turbulence. For decaying turbulence, power-law fits to the decay of turbulent kinetic energy reveal two regions over time; in the near-field region (
$1.22$. Two velocity components are measured by particle image velocimetry at the symmetry plane of the water tank. Measurements are taken for both ‘stationary’ forced turbulence and natural decaying turbulence. For decaying turbulence, power-law fits to the decay of turbulent kinetic energy reveal two regions over time; in the near-field region (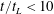 $t/t_{L}<10$,
$t/t_{L}<10$, 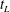 $t_{L}$ is the integral time scale of the forced turbulence) a decay exponent
$t_{L}$ is the integral time scale of the forced turbulence) a decay exponent 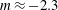 $m\approx -2.3$ is found whereas for the far-field region (
$m\approx -2.3$ is found whereas for the far-field region ( $t/t_{L}>10$) the value of the decay exponent was found to be affected by turbulence saturation. The near-field exhibits features of non-equilibrium turbulence with constant
$t/t_{L}>10$) the value of the decay exponent was found to be affected by turbulence saturation. The near-field exhibits features of non-equilibrium turbulence with constant 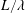 $L/\unicode[STIX]{x1D706}$ and varying
$L/\unicode[STIX]{x1D706}$ and varying 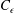 $C_{\unicode[STIX]{x1D716}}$ (dissipation constant). We found a decay exponent
$C_{\unicode[STIX]{x1D716}}$ (dissipation constant). We found a decay exponent 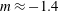 $m\approx -1.4$ for the unsaturated regime and
$m\approx -1.4$ for the unsaturated regime and  $m\approx -1.8$ for the saturated regime, in good agreement with previous numerical and experimental studies. We also observe a fast evolution towards isotropy at small scales, whereas anisotropy at large scales remains in the flow over more than
$m\approx -1.8$ for the saturated regime, in good agreement with previous numerical and experimental studies. We also observe a fast evolution towards isotropy at small scales, whereas anisotropy at large scales remains in the flow over more than  $100t_{L}$. Direct estimates of dissipation are obtained and the decay exponent agrees well with the prediction
$100t_{L}$. Direct estimates of dissipation are obtained and the decay exponent agrees well with the prediction 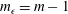 $m_{\unicode[STIX]{x1D716}}=m-1$ throughout the decay process.
$m_{\unicode[STIX]{x1D716}}=m-1$ throughout the decay process.