Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Durst, F.
Haddad, K.
and
Ertunç, Ö.
2009.
Advances in Turbulence XII.
Vol. 132,
Issue. ,
p.
131.
Zanoun, E.-S.
Kito, M.
and
Egbers, C.
2009.
A Study on Flow Transition and Development in Circular and Rectangular Ducts.
Journal of Fluids Engineering,
Vol. 131,
Issue. 6,
KUIK, D. J.
POELMA, C.
and
WESTERWEEL, J.
2010.
Quantitative measurement of the lifetime of localized turbulence in pipe flow.
Journal of Fluid Mechanics,
Vol. 645,
Issue. ,
p.
529.
Duguet, Yoann
Willis, A. P.
and
Kerswell, R. R.
2010.
États limites et bouffées turbulentes en conduite cylindrique.
Mécanique & Industries,
Vol. 11,
Issue. 2,
p.
157.
Dou, Hua-shu
and
Khoo, Boo Cheong
2010.
Energy gradient method for turbulent transition with consideration of effect of disturbance frequency.
Journal of Hydrodynamics,
Vol. 22,
Issue. S1,
p.
23.
Nishi, Mina
Ertunç, Özgür
and
Delgado, Antonio
2010.
Seventh IUTAM Symposium on Laminar-Turbulent Transition.
Vol. 18,
Issue. ,
p.
537.
DUGUET, Y.
WILLIS, A. P.
and
KERSWELL, R. R.
2010.
Slug genesis in cylindrical pipe flow.
Journal of Fluid Mechanics,
Vol. 663,
Issue. ,
p.
180.
Dou, Hua-Shu
Khoo, Boo Cheong
and
Tsai, Her Mann
2010.
Determining the critical condition for turbulent transition in a full-developed annulus flow.
Journal of Petroleum Science and Engineering,
Vol. 73,
Issue. 1-2,
p.
41.
DOU, HUA-SHU
and
KHOO, BOO CHEONG
2010.
CRITERIA OF TURBULENT TRANSITION IN PARALLEL FLOWS.
Modern Physics Letters B,
Vol. 24,
Issue. 13,
p.
1437.
Walton, Andrew G.
2011.
The stability of developing pipe flow at high Reynolds number and the existence of nonlinear neutral centre modes.
Journal of Fluid Mechanics,
Vol. 684,
Issue. ,
p.
284.
Dou, Hua-Shu
and
Khoo, Boo-Cheong
2011.
Theory and Prediction of Turbulent Transition.
International Journal of Fluid Machinery and Systems,
Vol. 4,
Issue. 1,
p.
114.
Barkley, Dwight
2011.
Modeling the transition to turbulence in shear flows.
Journal of Physics: Conference Series,
Vol. 318,
Issue. 3,
p.
032001.
Eckhardt, Bruno
2011.
A Critical Point for Turbulence.
Science,
Vol. 333,
Issue. 6039,
p.
165.
Barkley, Dwight
2011.
Simplifying the complexity of pipe flow.
Physical Review E,
Vol. 84,
Issue. 1,
Kurokawa, Junichi
2011.
J-Groove Technique for Suppressing Various Anomalous Flow Phenomena in Turbomachines.
International Journal of Fluid Machinery and Systems,
Vol. 4,
Issue. 1,
p.
1.
ICHIMIYA, Masashi
FUJIMURA, Hayato
and
TAMATANI, Junji
2011.
Laminar-Turbulent Transition of an Inlet Boundary Layer in a Circular Pipe Induced by Periodic Ejection (Condition for Generating an Isolated Turbulent Patch).
Journal of Fluid Science and Technology,
Vol. 6,
Issue. 6,
p.
902.
Sipos, Maksim
and
Goldenfeld, Nigel
2011.
Directed percolation describes lifetime and growth of turbulent puffs and slugs.
Physical Review E,
Vol. 84,
Issue. 3,
Mullin, T.
2011.
Experimental Studies of Transition to Turbulence in a Pipe.
Annual Review of Fluid Mechanics,
Vol. 43,
Issue. 1,
p.
1.
ICHIMIYA, Masashi
FUJIMURA, Hayato
and
TAMATANI, Junji
2011.
Laminar-Turbulent Transition of an Inlet Boundary Layer in a Circular Pipe Induced by Periodic Ejection (Condition for Generating an Isolated Turbulent Patch).
TRANSACTIONS OF THE JAPAN SOCIETY OF MECHANICAL ENGINEERS Series B,
Vol. 77,
Issue. 774,
p.
214.
Krauss, J
Ertunç, Ö
Ostwald, Ch
Lienhart, H
and
Delgado, A
2011.
Evolution of transitional structures from puff to slug through multiple splitting in a pipe flow at low Reynolds number.
Journal of Physics: Conference Series,
Vol. 318,
Issue. 3,
p.
032012.
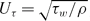 ; τw is the wall shear stress and ρ is the density of the fluid).
; τw is the wall shear stress and ρ is the density of the fluid).

