Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Konstantinidis, Efstathios
2019.
Active Control of Bluff-Body Flows Using Plasma Actuators.
Actuators,
Vol. 8,
Issue. 3,
p.
66.
Ping, Huan
Zhu, Hongbo
Zhang, Kai
Wang, Rui
Zhou, Dai
Bao, Yan
and
Han, Zhaolong
2020.
Wake dynamics behind a rotary oscillating cylinder analyzed with proper orthogonal decomposition.
Ocean Engineering,
Vol. 218,
Issue. ,
p.
108185.
Erfan, Iman
Abbaspour, Mohammad
and
Martinuzzi, Robert J.
2021.
Investigation of the influence of low-frequency forcing on the 3-D turbulent wake of a cantilevered triangular prism.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Konstantinidis, Efstathios
Dorogi, Dániel
and
Baranyi, László
2021.
Resonance in vortex-induced in-line vibration at low Reynolds numbers.
Journal of Fluid Mechanics,
Vol. 907,
Issue. ,
Hong, Seongjin
and
Son, Gihun
2021.
Numerical simulation of cavitating flows around an oscillating circular cylinder.
Ocean Engineering,
Vol. 226,
Issue. ,
p.
108739.
Ali, Ussama
Islam, M.D.
and
Janajreh, Isam
2022.
Flow over rotationally oscillating heated circular cylinder at low Reynolds number.
Ocean Engineering,
Vol. 265,
Issue. ,
p.
112515.
Chen, Wen-Li
Huang, Yewei
Chen, Changlong
Yu, Haiyang
and
Gao, Donglai
2022.
Review of active control of circular cylinder flow.
Ocean Engineering,
Vol. 258,
Issue. ,
p.
111840.
Hamdoon, Farouk Omar
Jaber, Alaa Abdulhady
and
Flaieh, Enass H.
2022.
An overset mesh approach for a vibrating cylinder in uniform flow.
Curved and Layered Structures,
Vol. 9,
Issue. 1,
p.
396.
Zhu, Yanqi
Jin, Hanhui
Guo, Yu
Ku, Xiaoke
and
Fan, Jianren
2022.
Lock-in phenomenon in the wake flow past an oscillating circular nano-cylinder.
AIP Advances,
Vol. 12,
Issue. 8,
Sarout, Yuvraj
Islam, Md
Ali, Ussama
Fatt, Yap Yit
and
Janajreh, Isam
2023.
Flow-Induced vibration and heat transfer enhancement in tandem cylinders: Effects of cylinder shape, corner radii, and spacing ratio.
Ocean Engineering,
Vol. 285,
Issue. ,
p.
115397.
Pang, Dan
Cheng, Liang
Jiang, Hongyi
Tong, Feifei
and
An, Hongwei
2023.
Suppression of vortex shedding in the wake of a circular cylinder through high-frequency in-line oscillation.
Physics of Fluids,
Vol. 35,
Issue. 7,
Le, Anh Dinh
Vu, Truong V.
and
Cong, Truong Dinh
2023.
Cavitation Flows Past a Rotating Circular Cylinder.
Journal of Fluids Engineering,
Vol. 145,
Issue. 11,
Ali, Ussama
Islam, Md
Kadi, Khadije El
and
Janajreh, Isam
2023.
Energy Harvesting from Wake-Induced Vibrations of a Downstream Cylinder in Tandem Arrangement.
Procedia Computer Science,
Vol. 224,
Issue. ,
p.
280.
Khan, Izhar Hussain
Sunil, Puja
Bhattacharyya, Soumarup
Yadav, Rahul
Poddar, Kamal
and
Kumar, Sanjay
2023.
Flow past two rotationally oscillating cylinders.
Journal of Fluid Mechanics,
Vol. 969,
Issue. ,
Barandagh, Ali Rezaei
Barandagh, Adel Rezaei
and
Ghazanfarian, Jafar
2023.
Hybrid Heat Transfer Augmentation from Oscillating Cylinder with Partial Superhydrophobic Janus Surfaces.
Heat Transfer Engineering,
Vol. 44,
Issue. 20,
p.
1878.
Zhang, Kai
Zhu, Hongbo
Cao, Yong
and
Zhou, Dai
2023.
Reynolds number effects on the bistable flows over a wavy circular cylinder.
Ocean Engineering,
Vol. 271,
Issue. ,
p.
113776.
2023.
A spectral force representation and its physical implication for vortex shedding past a stationary or an oscillating circular cylinder at low Reynolds number.
Physics of Fluids,
Vol. 35,
Issue. 5,
Roy, Ravikant
and
Peter, Simon
2024.
Fluid Mechanics and Fluid Power, Volume 2.
p.
681.
Phan, Thi Dieu Thuy
Nguyen, Dinh Thang
Nguyen, Van Luc
Tran, Tien-Anh
Le, Anh Tuan
Nguyen, Van Tien
and
Duong, Viet Dung
2024.
Wake transitions of the flow around two side-by-side elliptic cylinders.
Physics of Fluids,
Vol. 36,
Issue. 12,
Chen, Jian
Geng, Linlin
Jou, Esteve
and
Escaler, Xavier
2024.
Comparative Performance Assessment between Incompressible and Compressible Solvers to Simulate a Cavitating Wake.
Fluids,
Vol. 9,
Issue. 9,
p.
218.
 $Re=100$, lock-in is categorized according to the spectral features of the lift coefficient as two different lock-in phenomena: harmonic and subharmonic lock-in. These lock-in phenomena are represented as maps on the driving amplitude–frequency plane, which have subharmonic lock-in regions and two harmonic lock-in regions. The frequency range of the subharmonic region is shifted to lower frequencies with increasing amplitude, and the lower boundary of this subharmonic region is successfully predicted. A symmetric harmonic region with a symmetric vortex pattern is observed in a certain velocity range for a moving cylinder. Aerodynamic features induced by different flow patterns in each region are presented on the driving amplitude–frequency plane. The lock-in region and aerodynamic features at
$Re=100$, lock-in is categorized according to the spectral features of the lift coefficient as two different lock-in phenomena: harmonic and subharmonic lock-in. These lock-in phenomena are represented as maps on the driving amplitude–frequency plane, which have subharmonic lock-in regions and two harmonic lock-in regions. The frequency range of the subharmonic region is shifted to lower frequencies with increasing amplitude, and the lower boundary of this subharmonic region is successfully predicted. A symmetric harmonic region with a symmetric vortex pattern is observed in a certain velocity range for a moving cylinder. Aerodynamic features induced by different flow patterns in each region are presented on the driving amplitude–frequency plane. The lock-in region and aerodynamic features at 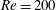 $Re=200$ and
$Re=200$ and 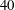 $40$ are compared with the results for
$40$ are compared with the results for 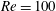 $Re=100$. A subharmonic region and two harmonic regions are observed at
$Re=100$. A subharmonic region and two harmonic regions are observed at  $Re=200$, and these show the same features as for
$Re=200$, and these show the same features as for 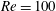 $Re=100$ at a low driving amplitude. Lock-in at
$Re=100$ at a low driving amplitude. Lock-in at 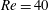 $Re=40$ also shows one subharmonic region and two harmonic regions. However, compared with the
$Re=40$ also shows one subharmonic region and two harmonic regions. However, compared with the 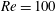 $Re=100$ case, the symmetric harmonic lock-in is dominant. The features of aerodynamic force at
$Re=100$ case, the symmetric harmonic lock-in is dominant. The features of aerodynamic force at 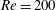 $Re=200$ and
$Re=200$ and 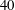 $40$ are represented on a force map, which shows similar characteristics in corresponding regions for the
$40$ are represented on a force map, which shows similar characteristics in corresponding regions for the  $Re=100$ case.
$Re=100$ case.