Article contents
Motion of a viscous slug on heterogeneous surfaces: crossover from stick–slip to steady sliding
Published online by Cambridge University Press: 10 October 2023
Abstract
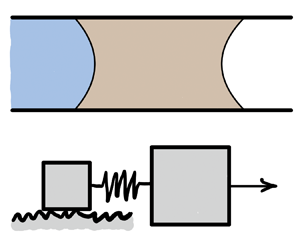
We present a theoretical study of viscous slug motion inside a microscopically rough capillary tube, where pronounced stick–slip motion can emerge at slow displacement rates. The mathematical description of this intermittent motion can be reduced to a system of ordinary differential equations, which also describe the motion of a pendulum inside a fluid-filled rotating drum. We use this analogy to show that the stick–slip motion transitions to steady sliding at high displacement rates. We characterize this crossover with a simple scaling relation and show that the crossover is accompanied by a shift in the dominant energy dissipation mechanisms within the system.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
Primkulov et al. Supplementary Movie
Video shows a transition from stick--slip to steady sliding with phase-field simulation of fluid-fluid displacement in a 2D channel. The crossover occurs as the capillary number increases from 1E-4 to 3E-2. Here, a dashed line represents an axis of symmetry in the 2D channel.
- 3
- Cited by


