Article contents
A numerical investigation of the turbulent Stokes–Ekman bottom boundary layer
Published online by Cambridge University Press: 06 September 2011
Abstract
In the present paper turbulent mixing in the Stokes–Ekman bottom boundary layer is investigated analytically and by wall-resolving large-eddy simulations (LES). The analytical solution shows that when the Rossby number  oscillation and rotation interact with each other, this gives rise to a thickening of the boundary layer compared with the purely oscillating or the purely rotating case. The solution also shows the presence of elliptical patterns developing on the horizontal planes that shrink when approaching the low latitudes. In the turbulent regime, a LES was applied for an east–west tidal current, considered at three different latitudes in the Northern Hemisphere, namely, the polar case, the mid-latitude case (
oscillation and rotation interact with each other, this gives rise to a thickening of the boundary layer compared with the purely oscillating or the purely rotating case. The solution also shows the presence of elliptical patterns developing on the horizontal planes that shrink when approaching the low latitudes. In the turbulent regime, a LES was applied for an east–west tidal current, considered at three different latitudes in the Northern Hemisphere, namely, the polar case, the mid-latitude case (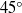 ) and the quasi-equatorial case (
) and the quasi-equatorial case ( ). The Reynolds number of the simulation, based on the viscous penetration depth and the frequency of the purely oscillatory flow, was set equal to
). The Reynolds number of the simulation, based on the viscous penetration depth and the frequency of the purely oscillatory flow, was set equal to  . The analysis suggests that rotation has two main effects on the flow field: in the polar case, rotation tends to delay the cyclic re-transition to turbulence and to narrow the turbulent phases of the cycle. Also, rotation suppresses vertical fluctuations of velocity and redistributes energy from the streamwise direction to the spanwise direction. It is noteworthy that the high-latitude effect makes the turbulent field substantially different from the reference Stokes boundary layer case, whereas the low-latitude effects appear to be of secondary importance, owing to the weakness of the rotation rate. Consequently, the study shows that the Stokes boundary layer may be representative of the oceanic bottom boundary layer in the low-latitude cases (
. The analysis suggests that rotation has two main effects on the flow field: in the polar case, rotation tends to delay the cyclic re-transition to turbulence and to narrow the turbulent phases of the cycle. Also, rotation suppresses vertical fluctuations of velocity and redistributes energy from the streamwise direction to the spanwise direction. It is noteworthy that the high-latitude effect makes the turbulent field substantially different from the reference Stokes boundary layer case, whereas the low-latitude effects appear to be of secondary importance, owing to the weakness of the rotation rate. Consequently, the study shows that the Stokes boundary layer may be representative of the oceanic bottom boundary layer in the low-latitude cases ( cases in our simulations). Conversely, it cannot be considered as archetypal of the oceanic boundary layer at high latitudes (
cases in our simulations). Conversely, it cannot be considered as archetypal of the oceanic boundary layer at high latitudes ( case of our study), where the vertical background vorticity profoundly modifies the turbulent field.
case of our study), where the vertical background vorticity profoundly modifies the turbulent field.
Information
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2011
References
- 10
- Cited by

