Article contents
Numerical simulations of Rayleigh–Bénard convection for Prandtl numbers between 10−1 and 104 and Rayleigh numbers between 105 and 109
Published online by Cambridge University Press: 14 September 2010
Abstract
We summarize the results of an extensive campaign of direct numerical simulations of Rayleigh–Bénard convection at moderate and high Prandtl numbers (10−1 ≤ Pr ≤ 104) and moderate Rayleigh numbers (105 ≤ Ra ≤ 109). The computational domain is a cylindrical cell of aspect ratio Γ = 1/2, with the no-slip condition imposed on all boundaries. By scaling the numerical results, we find that the free-fall velocity should be multiplied by 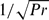 in order to obtain a more appropriate representation of the large-scale velocity at high Pr. We investigate the Nusselt and the Reynolds number dependences on Ra and Pr, comparing the outcome with previous numerical and experimental results. Depending on Pr, we obtain different power laws of the Nusselt number with respect to Ra, ranging from Ra2/7 for Pr = 1 up to Ra0.31 for Pr = 103. The Nusselt number is independent of Pr. The Reynolds number scales as
in order to obtain a more appropriate representation of the large-scale velocity at high Pr. We investigate the Nusselt and the Reynolds number dependences on Ra and Pr, comparing the outcome with previous numerical and experimental results. Depending on Pr, we obtain different power laws of the Nusselt number with respect to Ra, ranging from Ra2/7 for Pr = 1 up to Ra0.31 for Pr = 103. The Nusselt number is independent of Pr. The Reynolds number scales as  , neglecting logarithmic corrections. We analyse the global and local features of viscous and thermal boundary layers and their scaling behaviours with respect to Ra and Pr, and with respect to the Reynolds and Péclet numbers. We find that the flow approaches a saturation state when Reynolds number decreases below the critical value, Res ≃ 40. The thermal-boundary-layer thickness increases slightly (instead of decreasing) when the Péclet number increases, because of the moderating influence of the viscous boundary layer. The simulated ranges of Ra and Pr contain steady, periodic and turbulent solutions. A rough estimate of the transition from the steady to the unsteady state is obtained by monitoring the time evolution of the system until it reaches stationary solutions. We find multiple solutions as long-term phenomena at Ra = 108 and Pr = 103, which, however, do not result in significantly different Nusselt numbers. One of these multiple solutions, even if stable over a long time interval, shows a break in the mid-plane symmetry of the temperature profile. We analyse the flow structures through the transitional phases by direct visualizations of the temperature and velocity fields. A wide variety of large-scale circulation and plume structures has been found. The single-roll circulation is characteristic only of the steady and periodic solutions. For other regimes at lower Pr, the mean flow generally consists of two opposite toroidal structures; at higher Pr, the flow is organized in the form of multi-jet structures, extending mostly in the vertical direction. At high Pr, plumes mainly detach from sheet-like structures. The signatures of different large-scale structures are generally well reflected in the data trends with respect to Ra, less in those with respect to Pr.
, neglecting logarithmic corrections. We analyse the global and local features of viscous and thermal boundary layers and their scaling behaviours with respect to Ra and Pr, and with respect to the Reynolds and Péclet numbers. We find that the flow approaches a saturation state when Reynolds number decreases below the critical value, Res ≃ 40. The thermal-boundary-layer thickness increases slightly (instead of decreasing) when the Péclet number increases, because of the moderating influence of the viscous boundary layer. The simulated ranges of Ra and Pr contain steady, periodic and turbulent solutions. A rough estimate of the transition from the steady to the unsteady state is obtained by monitoring the time evolution of the system until it reaches stationary solutions. We find multiple solutions as long-term phenomena at Ra = 108 and Pr = 103, which, however, do not result in significantly different Nusselt numbers. One of these multiple solutions, even if stable over a long time interval, shows a break in the mid-plane symmetry of the temperature profile. We analyse the flow structures through the transitional phases by direct visualizations of the temperature and velocity fields. A wide variety of large-scale circulation and plume structures has been found. The single-roll circulation is characteristic only of the steady and periodic solutions. For other regimes at lower Pr, the mean flow generally consists of two opposite toroidal structures; at higher Pr, the flow is organized in the form of multi-jet structures, extending mostly in the vertical direction. At high Pr, plumes mainly detach from sheet-like structures. The signatures of different large-scale structures are generally well reflected in the data trends with respect to Ra, less in those with respect to Pr.
JFM classification
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2010
References
REFERENCES
- 74
- Cited by


