Article contents
Optimal perturbation for two-dimensional vortex systems: route to non-axisymmetric state
Published online by Cambridge University Press: 21 September 2018
Abstract
We investigate perturbations that maximize the gain of disturbance energy in a two-dimensional isolated vortex and a counter-rotating vortex pair. The optimization is carried out using the method of Lagrange multipliers. For low initial energy of the perturbation (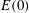 $E(0)$), the nonlinear optimal perturbation/gain is found to be the same as the linear optimal perturbation/gain. Beyond a certain threshold
$E(0)$), the nonlinear optimal perturbation/gain is found to be the same as the linear optimal perturbation/gain. Beyond a certain threshold 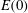 $E(0)$, the optimal perturbation/gain obtained from linear and nonlinear computations are different. There exists a range of
$E(0)$, the optimal perturbation/gain obtained from linear and nonlinear computations are different. There exists a range of 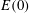 $E(0)$ for which the nonlinear optimal gain is higher than the linear optimal gain. For an isolated vortex, the higher value of nonlinear optimal gain is attributed to interaction among different azimuthal components, which is otherwise absent in a linearized system. Spiral dislocations are found in the nonlinear optimal perturbation at the radial location where the most dominant wavenumber changes. Long-time nonlinear evolution of linear and nonlinear optimal perturbations is studied. The evolution shows that, after the initial increment of perturbation energy, the vortex attains a quasi-steady state where the mean perturbation energy decreases on a slow time scale. The quasi-steady vortex state is non-axisymmetric and its shape depends on the initial perturbation. It is observed that the lifetime of a quasi-steady vortex state obtained using the nonlinear optimal perturbation is longer than that obtained using the linear optimal perturbation. For a counter-rotating vortex pair, the mechanism that maximizes the energy gain is found to be similar to that of the isolated vortex. Within the linear framework, the optimal perturbation for a vortex pair can be either symmetric or antisymmetric, whereas the structure of the nonlinear optimal perturbation, beyond the threshold
$E(0)$ for which the nonlinear optimal gain is higher than the linear optimal gain. For an isolated vortex, the higher value of nonlinear optimal gain is attributed to interaction among different azimuthal components, which is otherwise absent in a linearized system. Spiral dislocations are found in the nonlinear optimal perturbation at the radial location where the most dominant wavenumber changes. Long-time nonlinear evolution of linear and nonlinear optimal perturbations is studied. The evolution shows that, after the initial increment of perturbation energy, the vortex attains a quasi-steady state where the mean perturbation energy decreases on a slow time scale. The quasi-steady vortex state is non-axisymmetric and its shape depends on the initial perturbation. It is observed that the lifetime of a quasi-steady vortex state obtained using the nonlinear optimal perturbation is longer than that obtained using the linear optimal perturbation. For a counter-rotating vortex pair, the mechanism that maximizes the energy gain is found to be similar to that of the isolated vortex. Within the linear framework, the optimal perturbation for a vortex pair can be either symmetric or antisymmetric, whereas the structure of the nonlinear optimal perturbation, beyond the threshold 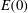 $E(0)$, is always asymmetric. No quasi-steady state for a counter-rotating vortex pair is observed.
$E(0)$, is always asymmetric. No quasi-steady state for a counter-rotating vortex pair is observed.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 7
- Cited by


