Article contents
Precursors of backflow events and their relationship with the near-wall self-sustaining process
Published online by Cambridge University Press: 29 December 2021
Abstract
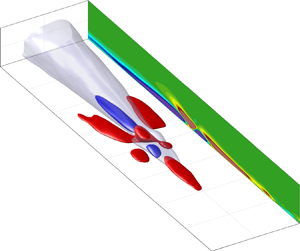
This study examines the precursors and consequences of rare backflow events at the wall using direct numerical simulation of turbulent pipe flow with a high spatiotemporal resolution. The results obtained from conditionally averaged fields reveal that the precursor of a backflow event is the asymmetric collision between a high- and a low-speed streak (LSS) associated with the sinuous mode of the streaks. As the collision occurs, a lifted shear layer with high local azimuthal enstrophy is formed at the trailing end of the LSS. Subsequently, a spanwise or an oblique vortex spontaneously arises. The dominant nonlinear mechanism by which this vortex is engendered is enstrophy intensification due to direct stretching of the lifted vorticity lines in the azimuthal direction. As time progresses, this vortex tilts and orientates towards the streamwise direction and, as its enstrophy increases, it induces the breakdown of the LSS located below it. Subsequently, this vortical structure advects as a quasi-streamwise vortex, as it tilts and stretches with time. As a result, it is shown that reverse flow events at the wall are the signature of the nonlinear mechanism of the self-sustaining process occurring at the near-wall region. Additionally, each backflow event has been tracked in space and time, showing that approximately 50 % of these events are followed by at least one additional vortex generation that gives rise to new backflow events. It is also found that up to a maximum of seven regenerations occur after a backflow event has appeared for the first time.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 11
- Cited by


