Article contents
Pressure losses in grooved channels
Published online by Cambridge University Press: 14 May 2013
Abstract
The effects of small-amplitude, two-dimensional grooves on pressure losses in a laminar channel flow have been analysed. Grooves with an arbitrary shape and an arbitrary orientation with respect to the flow direction have been considered. It has been demonstrated that losses can be expressed as a superposition of two parts, one associated with change in the mean positions of the walls and one induced by flow modulations associated with the geometry of the grooves. The former effect can be determined analytically, while the latter has to be determined numerically and can be captured with an acceptable accuracy using reduced-order geometry models. Projection of the wall shape onto a Fourier space has been used to generate such a model. It has been found that in most cases replacement of the actual wall geometry with the leading mode of the relevant Fourier expansion permits determination of pressure losses with an error of less than 10 %. Detailed results are given for sinusoidal grooves for the range of parameters of practical interest. These results describe the performance of arbitrary grooves with the accuracy set by the properties of the reduced-order geometry model and are exact for sinusoidal grooves. The results show a strong dependence of the pressure losses on the groove orientation. Longitudinal grooves produce the smallest drag, and oblique grooves with an inclination angle of 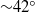 ${\sim }42\textdegree $ exhibit the largest flow turning potential. Detailed analyses of the extreme cases, i.e. transverse and longitudinal grooves, have been carried out. For transverse grooves with small wavenumbers, the dominant part of the drag is produced by shear, while the pressure form drag and the pressure interaction drag provide minor contributions. For the same grooves with large wavenumbers, the stream lifts up above the grooves due to their blocking effect, resulting in a change in the mechanics of drag formation: the contributions of shear decrease while the contributions of the pressure interaction drag increase, leading to an overall drag increase. In the case of longitudinal grooves, drag is produced by shear, and its rearrangement results in a drag decrease for long-wavelength grooves in spite of an increase of the wetted surface area. An increase of the wavenumber leads to the fluid being squeezed from the troughs and the stream being forced to lift up above the grooves. The shear is nearly eliminated from a large fraction of the wall but the overall drag increases due to reduction of the effective channel opening. It is shown that properly structured grooves are able to eliminate wall shear from the majority of the wetted surface area regardless of the groove orientation, thus exhibiting the potential for the creation of drag-reducing surfaces. Such surfaces can become practicable if a method for elimination of the undesired pressure and shear peaks through proper groove shaping can be found.
${\sim }42\textdegree $ exhibit the largest flow turning potential. Detailed analyses of the extreme cases, i.e. transverse and longitudinal grooves, have been carried out. For transverse grooves with small wavenumbers, the dominant part of the drag is produced by shear, while the pressure form drag and the pressure interaction drag provide minor contributions. For the same grooves with large wavenumbers, the stream lifts up above the grooves due to their blocking effect, resulting in a change in the mechanics of drag formation: the contributions of shear decrease while the contributions of the pressure interaction drag increase, leading to an overall drag increase. In the case of longitudinal grooves, drag is produced by shear, and its rearrangement results in a drag decrease for long-wavelength grooves in spite of an increase of the wetted surface area. An increase of the wavenumber leads to the fluid being squeezed from the troughs and the stream being forced to lift up above the grooves. The shear is nearly eliminated from a large fraction of the wall but the overall drag increases due to reduction of the effective channel opening. It is shown that properly structured grooves are able to eliminate wall shear from the majority of the wetted surface area regardless of the groove orientation, thus exhibiting the potential for the creation of drag-reducing surfaces. Such surfaces can become practicable if a method for elimination of the undesired pressure and shear peaks through proper groove shaping can be found.
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 59
- Cited by


