Article contents
Reduced-order representation of near-wall structures in the late transitional boundary layer
Published online by Cambridge University Press: 28 April 2014
Abstract
Direct numerical simulations (DNS) of controlled H- and K-type transitions to turbulence in an 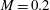 $M = 0.2$ (where
$M = 0.2$ (where 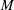 $M$ is the Mach number) nominally zero-pressure-gradient and spatially developing flat-plate boundary layer are considered. Sayadi, Hamman & Moin (J. Fluid Mech., vol. 724, 2013, pp. 480–509) showed that with the start of the transition process, the skin-friction profiles of these controlled transitions diverge abruptly from the laminar value and overshoot the turbulent estimation. The objective of this work is to identify the structures of dynamical importance throughout the transitional region. Dynamic mode decomposition (DMD) (Schmid, J. Fluid Mech., vol. 656, 2010, pp. 5–28) as an optimal phase-averaging process, together with triple decomposition (Reynolds & Hussain, J. Fluid Mech., vol. 54 (02), 1972, pp. 263–288), is employed to assess the contribution of each coherent structure to the total Reynolds shear stress. This analysis shows that low-frequency modes, corresponding to the legs of hairpin vortices, contribute most to the total Reynolds shear stress. The use of composite DMD of the vortical structures together with the skin-friction coefficient allows the assessment of the coupling between near-wall structures captured by the low-frequency modes and their contribution to the total skin-friction coefficient. We are able to show that the low-frequency modes provide an accurate estimate of the skin-friction coefficient through the transition process. This is of interest since large-eddy simulation (LES) of the same configuration fails to provide a good prediction of the rise to this overshoot. The reduced-order representation of the flow is used to compare the LES and the DNS results within this region. Application of this methodology to the LES of the H-type transition illustrates the effect of the grid resolution and the subgrid-scale model on the estimated shear stress of these low-frequency modes. The analysis shows that although the shapes and frequencies of the low-frequency modes are independent of the resolution, the amplitudes are underpredicted in the LES, resulting in underprediction of the Reynolds shear stress.
$M$ is the Mach number) nominally zero-pressure-gradient and spatially developing flat-plate boundary layer are considered. Sayadi, Hamman & Moin (J. Fluid Mech., vol. 724, 2013, pp. 480–509) showed that with the start of the transition process, the skin-friction profiles of these controlled transitions diverge abruptly from the laminar value and overshoot the turbulent estimation. The objective of this work is to identify the structures of dynamical importance throughout the transitional region. Dynamic mode decomposition (DMD) (Schmid, J. Fluid Mech., vol. 656, 2010, pp. 5–28) as an optimal phase-averaging process, together with triple decomposition (Reynolds & Hussain, J. Fluid Mech., vol. 54 (02), 1972, pp. 263–288), is employed to assess the contribution of each coherent structure to the total Reynolds shear stress. This analysis shows that low-frequency modes, corresponding to the legs of hairpin vortices, contribute most to the total Reynolds shear stress. The use of composite DMD of the vortical structures together with the skin-friction coefficient allows the assessment of the coupling between near-wall structures captured by the low-frequency modes and their contribution to the total skin-friction coefficient. We are able to show that the low-frequency modes provide an accurate estimate of the skin-friction coefficient through the transition process. This is of interest since large-eddy simulation (LES) of the same configuration fails to provide a good prediction of the rise to this overshoot. The reduced-order representation of the flow is used to compare the LES and the DNS results within this region. Application of this methodology to the LES of the H-type transition illustrates the effect of the grid resolution and the subgrid-scale model on the estimated shear stress of these low-frequency modes. The analysis shows that although the shapes and frequencies of the low-frequency modes are independent of the resolution, the amplitudes are underpredicted in the LES, resulting in underprediction of the Reynolds shear stress.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 63
- Cited by

