Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
McCraney, Joshua
Weislogel, Mark
and
Steen, Paul
2020.
OpenFOAM Simulations of Late Stage Container Draining in Microgravity.
Fluids,
Vol. 5,
Issue. 4,
p.
207.
McCraney, Joshua
Weislogel, Mark
and
Steen, Paul
2021.
The draining of capillary liquids from containers with interior corners aboard the ISS.
npj Microgravity,
Vol. 7,
Issue. 1,
Romero-Calvo, Álvaro
Schaub, Hanspeter
and
Cano-Gómez, Gabriel
2022.
Diamagnetically Enhanced Electrolysis and Phase Separation in Low Gravity.
Journal of Spacecraft and Rockets,
Vol. 59,
Issue. 1,
p.
59.
Romero-Calvo, Álvaro
Akay, Ömer
Schaub, Hanspeter
and
Brinkert, Katharina
2022.
Magnetic phase separation in microgravity.
npj Microgravity,
Vol. 8,
Issue. 1,
Wu, Zongyu
Li, Kai
Han, Wei
Li, Guangyu
Fan, Chengguang
and
Xu, Bingui
2022.
Simulation Analysis of Liquid Flow in a Vane-type Surface Tension Tank.
Microgravity Science and Technology,
Vol. 34,
Issue. 5,
Kubochkin, Nikolai
and
Gambaryan-Roisman, Tatiana
2022.
Capillary-driven flow in corner geometries.
Current Opinion in Colloid & Interface Science,
Vol. 59,
Issue. ,
p.
101575.
Weislogel, M. M.
Graf, J. C.
Wollman, A. P.
Turner, C. C.
Cardin, K. J. T.
Torres, L. J.
Goodman, J. E.
and
Buchli, J. C.
2022.
How advances in low-g plumbing enable space exploration.
npj Microgravity,
Vol. 8,
Issue. 1,
Chen, Shangtong
Wu, Di
Li, Yong
Liu, Jintao
Duan, Li
and
Li, Wen
2022.
Profiles of Liquid on the Surface of Revolution with Varying Cross-section under Microgravity.
Microgravity Science and Technology,
Vol. 34,
Issue. 6,
McCraney, J.
Ludwicki, J.
Bostwick, J.
Daniel, S.
and
Steen, P.
2022.
Coalescence-induced droplet spreading: Experiments aboard the International Space Station.
Physics of Fluids,
Vol. 34,
Issue. 12,
Chen, Shangtong
Wu, Di
Li, Wen
Ding, Fenglin
Kang, Qi
and
Li, Yong
2023.
Capillary Phenomena in Tubes Under Microgravity.
Brazilian Journal of Physics,
Vol. 53,
Issue. 5,
Chen, Shangtong
Zhang, Chu
Li, Wen
Li, Yong
Ding, Fenglin
and
Kang, Qi
2023.
Capillary phenomena in the corner of truncated-cone-shaped containers under microgravity.
Acta Mechanica Sinica,
Vol. 39,
Issue. 1,
McCraney, Joshua
Bostwick, Joshua
Weislogel, Mark
and
Steen, Paul
2023.
Bubble migration in containers with interior corners under microgravity conditions.
Experiments in Fluids,
Vol. 64,
Issue. 8,
Hart, Samuel T.
Lightsey, E. Glenn
and
Romero-Calvo, Álvaro
2024.
Thermoelectromagnetic SmallSat propellant positioning.
Acta Astronautica,
Vol. 214,
Issue. ,
p.
427.
 $t$, the nominal liquid depth is
$t$, the nominal liquid depth is  $h\sim t^{-1}$, the liquid volume is
$h\sim t^{-1}$, the liquid volume is 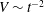 $V\sim t^{-2}$ and the maximum volumetric liquid removal rate is
$V\sim t^{-2}$ and the maximum volumetric liquid removal rate is  $Q\sim t^{-3}$. The constraints under which the solution is valid are provided. To qualitatively assess the value of the solution, representative experiments are conducted at larger length scales aboard the International Space Station and at microfluidic length scales in a terrestrial laboratory. Both sets of experiments confirm the predicted power-law dependences. We show that the separation of variables solution offers a method to predict maximum drain rates from related geometries where a single drain location provides the required symmetry of the problem.
$Q\sim t^{-3}$. The constraints under which the solution is valid are provided. To qualitatively assess the value of the solution, representative experiments are conducted at larger length scales aboard the International Space Station and at microfluidic length scales in a terrestrial laboratory. Both sets of experiments confirm the predicted power-law dependences. We show that the separation of variables solution offers a method to predict maximum drain rates from related geometries where a single drain location provides the required symmetry of the problem.