Article contents
Three-dimensionality of elliptical cylinder wakes at low angles of incidence
Published online by Cambridge University Press: 20 July 2017
Abstract
The wake of an elliptical cylinder at low incident angles is investigated for different aspect ratio (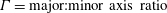 $\unicode[STIX]{x1D6E4}=\text{major:minor axis ratio}$) cylinders using stability analysis and direct simulations. In particular, two- and three-dimensional transitions are mapped for cylinders of aspect ratios between 1 and 4 using Floquet stability analysis. The transition scenario for near-unity aspect ratio cylinders resembles that for a circular cylinder wake as Reynolds number is increased to
$\unicode[STIX]{x1D6E4}=\text{major:minor axis ratio}$) cylinders using stability analysis and direct simulations. In particular, two- and three-dimensional transitions are mapped for cylinders of aspect ratios between 1 and 4 using Floquet stability analysis. The transition scenario for near-unity aspect ratio cylinders resembles that for a circular cylinder wake as Reynolds number is increased to  $Re\lesssim 400$; first, with the transition from steady two-dimensional flow to unsteady two-dimensional flow, followed by the onset of three-dimensional flow via a long-wavelength instability (mode A), then, a short-wavelength instability (mode B) and, finally, an intermediary wavelength instability which is quasi-periodic in nature (mode QP). The effect of the incident angle on this transition scenario for the low-aspect-ratio cylinders is minimal. As the aspect ratio is increased towards 2, two synchronous modes, modes
$Re\lesssim 400$; first, with the transition from steady two-dimensional flow to unsteady two-dimensional flow, followed by the onset of three-dimensional flow via a long-wavelength instability (mode A), then, a short-wavelength instability (mode B) and, finally, an intermediary wavelength instability which is quasi-periodic in nature (mode QP). The effect of the incident angle on this transition scenario for the low-aspect-ratio cylinders is minimal. As the aspect ratio is increased towards 2, two synchronous modes, modes 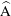 $\widehat{\text{A}}$ and
$\widehat{\text{A}}$ and 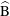 $\widehat{\text{B}}$, become unstable; these modes have spatio-temporal symmetries similar to their circular cylinder wake counterparts, modes A and mode B, respectively. While mode
$\widehat{\text{B}}$, become unstable; these modes have spatio-temporal symmetries similar to their circular cylinder wake counterparts, modes A and mode B, respectively. While mode 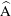 $\widehat{\text{A}}$ persists for all incident angles investigated here, mode
$\widehat{\text{A}}$ persists for all incident angles investigated here, mode 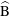 $\widehat{\text{B}}$ is found only to be unstable for incident angles up to
$\widehat{\text{B}}$ is found only to be unstable for incident angles up to 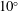 $10^{\circ }$. Surprisingly, for
$10^{\circ }$. Surprisingly, for 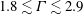 $1.8\lesssim \unicode[STIX]{x1D6E4}\lesssim 2.9$, the mode A instability observed at zero incident angle emerges as a quasi-periodic mode as the incident angle is increased even slightly. At higher incident angles, this quasi-periodic mode once again transforms to a real mode on increasing the Reynolds number. The parameter space maps for the various aspect ratios are presented in the Reynolds number–incident angle plane, and the three-dimensional modes are discussed in terms of similarities to and differences from existing modes. A key aim of the work is to map the different modes and various transition sequences as a simple body geometry is systematically changed and as the flow symmetry is systematically broken; thus, insight is provided on the overall path towards fully turbulent flow.
$1.8\lesssim \unicode[STIX]{x1D6E4}\lesssim 2.9$, the mode A instability observed at zero incident angle emerges as a quasi-periodic mode as the incident angle is increased even slightly. At higher incident angles, this quasi-periodic mode once again transforms to a real mode on increasing the Reynolds number. The parameter space maps for the various aspect ratios are presented in the Reynolds number–incident angle plane, and the three-dimensional modes are discussed in terms of similarities to and differences from existing modes. A key aim of the work is to map the different modes and various transition sequences as a simple body geometry is systematically changed and as the flow symmetry is systematically broken; thus, insight is provided on the overall path towards fully turbulent flow.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 15
- Cited by



