Published online by Cambridge University Press: 28 March 2013
Streamwise variation in vessel geometry is a feature of many multiphase flows of practical interest, ranging from natural porous media flows to man-made lab-on-the-chip applications. The variable streamwise geometry typically exerts a dominant influence on bubble motion, and can lead to undesirable phenomena such as clogging of the vessel. Here, we study clogging in a fundamental configuration, where a tube of square cross-section is suddenly expanded over a short streamwise distance. The extent to which a bubble driven by constant flux flow broadens to partially fill the expansion depends on the balance between viscous and surface tension stresses, measured by the capillary number 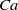 $\mathit{Ca}$. This broadening is accompanied by the slowing and momentary arrest of the bubble as
$\mathit{Ca}$. This broadening is accompanied by the slowing and momentary arrest of the bubble as 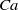 $\mathit{Ca}$ is reduced towards its critical value for trapping. For
$\mathit{Ca}$ is reduced towards its critical value for trapping. For  $\mathit{Ca}\lt {\mathit{Ca}}_{c} $ the pressure drag forces on the quasi-arrested bubble are insufficient to force the bubble out of the expanded region so it remains trapped. We examine the conditions for trapping by varying bubble volume, flow rate of the carrier fluid, relative influence of gravity and length of expanded region. We find specifically that
$\mathit{Ca}\lt {\mathit{Ca}}_{c} $ the pressure drag forces on the quasi-arrested bubble are insufficient to force the bubble out of the expanded region so it remains trapped. We examine the conditions for trapping by varying bubble volume, flow rate of the carrier fluid, relative influence of gravity and length of expanded region. We find specifically that 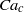 ${\mathit{Ca}}_{c} $ depends non-monotonically on the size of the bubble. We verify, with experiments and a capillary static model, that a bubble is released if the work of the pressure forces over the length of the trap exceeds the surface energy required for the trapped bubble to reenter the constricted square tube.
${\mathit{Ca}}_{c} $ depends non-monotonically on the size of the bubble. We verify, with experiments and a capillary static model, that a bubble is released if the work of the pressure forces over the length of the trap exceeds the surface energy required for the trapped bubble to reenter the constricted square tube.