Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-10T05:38:00.043Z Has data issue: false hasContentIssue false
  • English
  • Français

Bypass transition in a boundary layer flow induced by plasma actuators

Published online by Cambridge University Press:  19 October 2021

Dandan Xiao Open the ORCID record for Dandan Xiao [Opens in a new window] ,
Huw Borradaile ,
Kwing-So Choi Open the ORCID record for Kwing-So Choi [Opens in a new window] ,
Lihao Feng Open the ORCID record for Lihao Feng [Opens in a new window] ,
Jinjun Wang Open the ORCID record for Jinjun Wang [Opens in a new window]  and
Xuerui Mao Open the ORCID record for Xuerui Mao [Opens in a new window]
Dandan Xiao
Affiliation:
Faculty of Engineering, University of Nottingham, Nottingham NG7 2RD, UK
Huw Borradaile
Affiliation:
Faculty of Engineering, University of Nottingham, Nottingham NG7 2RD, UK
Kwing-So Choi
Affiliation:
Faculty of Engineering, University of Nottingham, Nottingham NG7 2RD, UK
Lihao Feng
Affiliation:
Institute of Fluid Mechanics, Beihang University, Beijing 100191, PR China
Jinjun Wang
Affiliation:
Institute of Fluid Mechanics, Beihang University, Beijing 100191, PR China
Xuerui Mao*
Affiliation:
Faculty of Engineering, University of Nottingham, Nottingham NG7 2RD, UK
*
†Email address for correspondence: maoxuerui@sina.com
Get access Rights & Permissions [Opens in a new window]

Abstract

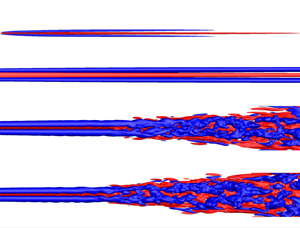
Bypass transition in flow over a flat plate triggered by a pair of dielectric-barrier-discharge plasma actuators mounted on the plate surface and aligned in the streamwise direction is investigated. A four-species plasma–fluid model is used to model the electrohydrodynamic force generated by the plasma actuation. A pair of counter-rotating streamwise vortices is created downstream of the actuators, leading to the formation of a high-speed streak in the centre and two low-speed streaks on each side. As the length of actuators increases, more momentum is added to the boundary layer and eventually a turbulent wedge is generated at an almost fixed location. With large spanwise distance between the actuators (wide layout), direct numerical simulations indicate that the low-speed streaks on both sides lose secondary stability via an inclined varicose-like mode simultaneously, leaving a symmetric perturbation pattern with respect to the centre of the actuators. Further downstream, the perturbations are tilted by the mean shear of the high- and low-speed streaks and consequently a ‘W’-shaped pattern is observed. When the pair of plasma actuators is placed closer (narrow layout) in the spanwise direction, the mean shear in the centre becomes stronger and secondary instability first occurs on the high-speed streak with an asymmetric pattern. Inclined varicose-like and sinuous-like instabilities coexist in the following breakdown of the negative streaks on the side and the perturbations remain asymmetric with respect to the centre. Here the tilting of disturbances is dominated by the mean shear in the centre and the perturbations display a ‘V’ shape. Linear analysis techniques, including biglobal stability and transient growth, are performed to further examine the fluid physics; the aforementioned phenomena at narrow and wide layouts, such as the secondary instabilities, the ‘V’ and ‘W’ shapes, and the symmetric and asymmetric breakdown, are all observed.

JFM classification

Boundary Layers: Boundary layer stability Instability: Transition to turbulence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 929 , 25 December 2021 , A6
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Andersson, P., Berggren, M. & Henningson, D.S. 1999 Optimal disturbances and bypass transition in boundary layers. Phys. Fluids 11, 134–150.CrossRefGoogle Scholar
Andersson, P., Brandt, L., Bottaro, A. & Henningson, D.S. 2001 On the breakdown of boundary layer streaks. J. Fluid Mech. 428, 29–60.CrossRefGoogle Scholar
Asai, M., Minagawa, M. & Nishioka, M. 2002 The instability and breakdown of a near-wall low-speed streak. J. Fluid Mech. 455, 289–314.CrossRefGoogle Scholar
Bade, K.M., Hanson, R.E., Belson, B.A., Naguib, A.M., Lavoie, P. & Rowley, C.W. 2016 Reactive control of isolated unsteady streaks in a laminar boundary layer. J. Fluid Mech. 795, 808–846.CrossRefGoogle Scholar
Barkley, D., Blackburn, H.M. & Sherwin, S.J. 2008 Direct optimal growth analysis for timesteppers. Intl J. Numer. Meth. Fluids 57, 1435–1458.CrossRefGoogle Scholar
Biancofiore, L., Brandt, L. & Zaki, T.A. 2017 Streak instability in viscoelastic Couette flow. Phys. Rev. Fluids 2, 043304.CrossRefGoogle Scholar
Boeuf, J.P., Lagmich, Y., Unfer, T. & Pitchford, L.C. 2007 Electrohydrodynamic force in dielectric barrier discharge plasma actuators. J. Phys. D: Appl. Phys 40, 652–662.CrossRefGoogle Scholar
Brandt, L. & Henningson, D.S. 2002 Transition of streamwise streaks in zero-pressure-gradient boundary layers. J. Fluid Mech. 472, 229–261.CrossRefGoogle Scholar
Brandt, L., Schlatter, P.S. & Henningson, D.S. 2004 Transition in boundary layers subject to free-stream turbulence. J. Fluid Mech. 517, 167–198.CrossRefGoogle Scholar
Bucci, M.A., Puckert, D.K., Andriano, C., Loiseau, J.C., Cherubini, S., Robinet, J.C. & Rist, U. 2018 Roughness-induced transition by quasi-resonance of a varicose global mode. J. Fluid Mech. 836, 167–191.CrossRefGoogle Scholar
Butler, K.M. & Farrell, B.F. 1992 Three-dimensional optimal perturbations in viscouss shear flow. Phys. Fluids A 4 (8), 1637–1650.CrossRefGoogle Scholar
Cherubini, S., De Tullio, M.D., De Palma, P. & Pascazio, G. 2013 Transient growth in the flow past a three-dimensional smooth roughness element. J. Fluid Mech. 724, 624–670.CrossRefGoogle Scholar
Choi, K.S., Jukes, T.N. & Whalley, R.D. 2011 Turbulent boundary-layer control with plasma actuators. Phil. Trans. R. Soc. Lond. A 369, 1443–1458.Google ScholarPubMed
Choi, K.S. & Kim, J.H. 2018 Plasma virtual roughness elements for cross-flow instability control. Exp. Fluids 59, 159.CrossRefGoogle Scholar
Cossu, C. & Brandt, L. 2002 Stabilization of Tollmien–Schlichting waves by finite amplitude optimal streaks in the blasius boundary layer. Phys. Fluids 14, L57.CrossRefGoogle Scholar
De Giorgi, M.G., Ficarella, A., Marra, F. & Pescini, E. 2017 Micro DBD plasma actuators for flow separation control on a low pressure turbine at high altitude flight operating conditions of aircraft engines. Appl. Thermal Eng. 114, 511–522.CrossRefGoogle Scholar
De Tullio, N., Paredes, P., Sandham, N.D. & Theofilis, V. 2013 Lamina-turbulent transition induced by a discrete roughness element in a supersonic boundary layer. J. Fluid Mech. 735, 613–646.CrossRefGoogle Scholar
von Deyn, L.H., Forooghi, P., Frohnapfel, B., Schlatter, P., Hanifi, A. & Henningson, D.S. 2020 Direct numerical simulations of bypass transition over distributed roughness. AIAA J. 58 (2), 702–711.CrossRefGoogle Scholar
Downs, R.S., White, E.B. & Denissen, N.A. 2008 Transient growth and transition induced by random distributed roughness. AIAA J. 46 (2), 451–462.CrossRefGoogle Scholar
Duchmann, A., Grundmann, S. & Tropea, C. 2014 Delay of natural transition with dielectric barrier discharges. Exp. Fluids 54, 1461.CrossRefGoogle Scholar
Dufour, G. & Rogier, F. 2015 Numerical modeling of dielectric barrier discharge based plasma actuators for flow control: the COPAIER/CEDRE example. AerospaceLab 10, 12726.Google Scholar
Feng, L., Jukes, T., Choi, K.S. & Wang, J. 2012 Flow control over a NACA 0012 airfoil using dielectric-barrier-discharge plasma actuator with a gurney flap. Exp. Fluids 52, 1533–1546.CrossRefGoogle Scholar
Fransson, J.H.M., Matsubara, M. & Alfredsson, P.H. 2005 Transition induced by free-stream turbulence. J. Fluid Mech. 527, 1–25.CrossRefGoogle Scholar
Gaster, M., Grosch, C.E. & Jackson, T.L. 1994 The velocity field created by a shallow bump in a boundary layer. Phys. Fluids 6 (9), 3079–3085.CrossRefGoogle Scholar
Goldstein, D.B., Chu, J. & Brown, G.L. 2017 Lateral spreading mechanism of a turbulent spot and a turbulent wedge. Flow Turbul. Combust. 98, 21–35.CrossRefGoogle Scholar
Gouder, K.A., Naguib, A.M., Lavoie, P.L. & Morrison, J.F. 2017 Control of boundary layer streaks induced by freestream turbulence using plasma actuators. In 10th International Symposium on Turbulence and Shear Flow Phenomena 2017.Google Scholar
Greenbelt, D., Schneider, T. & Schule, C.Y. 2012 Mechanism of flow separation control using plasma actuation. Phys. Fluids 24, 077102.CrossRefGoogle Scholar
Grundmann, S. & Tropea, C. 2008 Active cancellation of artificially induced tollmien-schlichting waves using plasma actuators. Exp. Fluids 44, 795–806.CrossRefGoogle Scholar
Hack, M. & Zaki, T.A. 2014 Streak instabilities in boundary layers beneath free-stream turbulence. J. Fluid Mech. 741, 280–315.CrossRefGoogle Scholar
Hack, M.J.P. & Moin, P. 2017 Algebraic disturbance growth by interaction of Orr and lift-up mechanisms. J. Fluid Mech. 829, 112–126.CrossRefGoogle Scholar
Hagelaar, G.J.M. & Pitchford, L.C. 2005 Solving the Boltzmann equation to obtain electron transport coefficients and rate coefficients for fluid modes. Plasma Sources Sci. Technol. 14, 722–733.CrossRefGoogle Scholar
Hanson, R.E., Lavoie, P., Naguib, A.M. & Morrison, J.F. 2010 Transient growth instability cancelation by a plasma actuator array. Exp. Fluids 49, 1339–1348.CrossRefGoogle Scholar
Hœpffner, J., Brandt, L. & Henningson, D.S. 2005 Transient growth on boundary layer streaks. J. Fluid Mech. 537, 91–100.CrossRefGoogle Scholar
Huang, W., Xiao, D., Ren, J., Wang, Z., Xi, G. & Mao, X. 2021 Bypass transition in flow over a vibrating flat plate. J. Fluid Mech. 909, A15.CrossRefGoogle Scholar
Hunt, J. & Durbin, P.A. 1999 Perturbed vortical layers and shear sheltering. Fluid Dyn. Res. 24, 375.CrossRefGoogle Scholar
Jacobs, R.G. & Durbin, P.A. 2001 Simulations of bypass transition. J. Fluid Mech. 428, 185–212.CrossRefGoogle Scholar
Jukes, T.N. & Choi, K.S. 2012 Dielectric-barrier-discharge vortex generators: characterisation and optimisation for flow separation control. Exp. Fluids 52 (2), 329–345.CrossRefGoogle Scholar
Jukes, T.N. & Choi, K.S. 2013 On the formation of streamwise vortices by plasma vortex generators. J. Fluid Mech. 733, 370–393.CrossRefGoogle Scholar
Jukes, T.N., Choi, K.S., Segawa, T. & Yoshida, H. 2008 Jet flow induced by a surface plasma actuator. Proc. Inst. Mech. Engrs 222, 347–356.Google Scholar
Kendall, J.M. 1985 Experiments on boundary-layer receptivity to freestream turbulence. AIAA Paper 1998-530.CrossRefGoogle Scholar
Kourtzanidis, K., Dufour, G. & Rogier, F. 2020 a Explaining the electrohydrodynamic force and ionic wind spatiotemporal distribution in surface ac dielectric barrier discharge actuators. arXiv:2007.01614.Google Scholar
Kourtzanidis, K., Dufour, G. & Rogier, F. 2020 b Self-consistent modeling of a surface ac dielectric barrier discharge actuator: in-depth analysis of positive and negative phases. J. Phys. D: Appl. Phys 54 (4), 045203.CrossRefGoogle Scholar
Landahl, M.T. 1975 Wave breakdown and turbulence. SIAM J. Appl. Maths 28, 735–756.CrossRefGoogle Scholar
Landahl, M.T. 1980 A note on an algebraic instability of inviscid parallel shear flows. J. Fluid Mech. 98, 243–251.CrossRefGoogle Scholar
Little, J., Nishinara, M., Adamovich, I. & Samimy, M. 2010 High-lift airfoil trailing edge separation control using a single dielectric barrier discharge plasma actuator. Exp. Fluids 48, 521–537.CrossRefGoogle Scholar
Loiseau, J.C., Robinet, J.C., Cherubini, S. & Leriche, E. 2014 Investigation of the roughness induced transition: global stability analyses and direct numerical simulations. J. Fluid Mech. 760, 175–211.CrossRefGoogle Scholar
Mahfoze, O. & Laizet, S. 2017 Skin-friction drag reduction in a channel flow with streamwise-aligned plasma actuators. Intl J. Heat Fluid Flow 66, 83–94.CrossRefGoogle Scholar
Mao, X. 2015 Effects of base flow modifications on noise amplifications: flow past a backward-facing step. J. Fluid Mech. 771, 229–263.CrossRefGoogle Scholar
Mao, X. & Hussain, F. 2017 Optimal transient growth on a vortex ring and its transition via cascade of ringlets. J. Fluid Mech. 832, 269–286.CrossRefGoogle Scholar
Mao, X., Zaki, T.A., Blackburn, H.M. & Sherwin, S.J. 2017 Transition induced by linear and nonlinear perturbation growth in flow past a compressor blade. J. Fluid Mech. 820, 604–632.CrossRefGoogle Scholar
Matsubara, M. & Alfredsson, P.H. 2001 Disturbance growtn in boundary layer subjected to free-stream turbulence. J. Fluid Mech. 430, 149–168.CrossRefGoogle Scholar
Mertz, B.E. & Corke, T.C. 2011 Single-dielectric barrier discharge plasma actuator modelling and validation. J. Fluid Mech. 669, 557–583.CrossRefGoogle Scholar
Monokrousos, A., Åkervik, E., Brandt, L. & Henningson, D.S. 2010 Global three-dimensional optimal disturbances in the blasius boundary-layer flow using time-steppers. J. Fluid Mech. 650, 181–214.CrossRefGoogle Scholar
Moreau, E. 2007 Airflow control by non-thermal plasma actuators. J. Phys. D: Appl. Phys 40 (3), 605–636.CrossRefGoogle Scholar
Nishida, H., Nonomura, T. & Abe, T. 2014 Three-dimensional simulations of discharge plasma evolution on a dielectric barrier discharge plasma actuator. J. Appl. Phys. 115 (13), 133301.CrossRefGoogle Scholar
Phelps, A.V. 2012 Phelps database. LXCat.Google Scholar
Pitchford, L.C. & Boeuf, J.P. 2012 Siglo database, lxcat. LXCat.Google Scholar
Puckert, D.K. & Rist, U. 2018 Global instability in a laminar boundary layer perturbed by an isolated roughness element. Exp. Fluids 59, 48.CrossRefGoogle Scholar
Puckert, D.K. & Rist, U. 2019 Experimental observation of frequency lock-in of roughness-induced instabilities in a laminar boundary layer. J. Fluid Mech. 870, 680–697.CrossRefGoogle Scholar
Riherd, M. & Roy, S. 2013 Damping Tollmien–Schlichting waves in a boundary layer using plasma actuators. J. Phys. D: Appl. Phys 45, 485203.CrossRefGoogle Scholar
Riherd, M. & Roy, S. 2014 Stabilization of boundary layer streaks by plasma actuators. J. Phys. D: Appl. Phys 47, 125203.CrossRefGoogle Scholar
Saikishan, S., Goldstein, D.B. & Brown, G.L. 2019 Roughness induced transition: a vorticity point of view. Phys. Fluids 31 (2), 024101.Google Scholar
Schatzman, D. & Thomas, F. 2010 Turbulent boundary-layer separation control with single dielectric barrier discharge plasma actuators. AIAA J. 48 (8), 1620–1634.CrossRefGoogle Scholar
Schmid, P.J. & Henningson, D.S. 2001 Stability and Transition in Shear Flows. AMS Book Series, vol. 142. Springer.CrossRefGoogle Scholar
Schubauer, G.B. & Klebanoff, P.S. 1955 Contributions on the mechanics of boundary-layer transition. NACA Tech. Rep. N-3489. National Advisory Committee for Aeronautics.Google Scholar
Shyy, W., Jayaraman, B. & Andersson, A. 2002 Single-dielectric barrier discharge plasma actuator modelling and validation. J. Fluid Mech. 92 (11), 6434–6443.Google Scholar
Suzen, Y., Huang, P., Jacob, J. & Ashpis, D. 2005 Numerical simulations of plasma based flow control applications. AIAA Paper 2005-4633.CrossRefGoogle Scholar
Swearingen, J.D. & Blackwelder, R.F. 1987 The growth and breakdown of streamwise vortices in the presence of a wall. J. Fluid Mech. 182, 255–290.CrossRefGoogle Scholar
Taylor, G.I. 1939 Some recent development in the study of turbulence. In Proceedings of the Fifth International Congress for Applied Mechanics (ed. J.P. Den Hartog & H. Peters), vol. 531, pp. 294–310.Google Scholar
Thomas, C.C., Enloe, C.L. & Wilkinson, S.P. 2010 Dielectric barrier discharge plasma actuators for flow control. Annu. Rev. Fluid Mech. 42, 505–529.Google Scholar
Vaughan, N. & Zaki, T.A. 2011 Stability of zero-pressure-gradient boundary layer distorted by unsteady Klebanoff streaks. J. Fluid Mech. 681, 116–153.CrossRefGoogle Scholar
Walker, S. & Segawa, T. 2012 Mitigation of flow separation using dbd plasma actuators on airfoils: a tool for more efficient wind turbine operation. J. Renew. Energy 42, 105–110.CrossRefGoogle Scholar
Wang, B., Mao, X. & Zaki, T.A. 2019 Low-frequency selectivity in flat-plate boundary layer with elliptic leading edge. J. Fluid Mech. 866, 239–262.CrossRefGoogle Scholar
Whalley, R.D. & Choi, K.S. 2013 The starting vortex in quiescent air induced by dielectric-barrier-discharge plasma. J. Fluid Mech. 703, 192–203.CrossRefGoogle Scholar
White, E.B. 2002 Transient growth of stationary disturbances in a flat plate boundary layer. Phys. Fluids 14 (12), 4429–4439.CrossRefGoogle Scholar
White, E.B., Rice, J.M. & Ergin, F.G. 2005 Receptivity of stationary transient disturbances to surface roughness. Phys. Fluids 17 (6), 064109.CrossRefGoogle Scholar
Wilkinson, S. 2003 Oscillating plasma for turbulent boundary layer drag reduction. 41st AIAA Aerospace Sciences Meeting. AIAA Paper 2003-1023.CrossRefGoogle Scholar
Zaki, T.A. & Durbin, P.A. 2005 Mode interaction and the bypass route to transition. J. Fluid Mech. 531, 85–111.CrossRefGoogle Scholar
Zhong, S., Chong, T.P. & Hodson, H.P. 2003 A comparison of spreading angles of turbulent wedges in velocity and thermal boundary layers. Trans. ASME J. Fluids Engng 125 (2), 267–274.CrossRefGoogle Scholar