Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-29T04:50:38.389Z Has data issue: false hasContentIssue false
  • English
  • Français

Characteristics of the pressure fluctuations generated in turbulent boundary layers over rough surfaces

Published online by Cambridge University Press:  20 November 2019

Liselle A. Joseph Open the ORCID record for Liselle A. Joseph [Opens in a new window] ,
Nicholas J. Molinaro ,
William J. Devenport  and
Timothy W. Meyers
Liselle A. Joseph
Affiliation:
Department of Aerospace and Ocean Engineering, Virginia Tech, Blacksburg, VA24060, USA
Nicholas J. Molinaro
Affiliation:
Department of Aerospace and Ocean Engineering, Virginia Tech, Blacksburg, VA24060, USA
William J. Devenport*
Affiliation:
Department of Aerospace and Ocean Engineering, Virginia Tech, Blacksburg, VA24060, USA
Timothy W. Meyers
Affiliation:
Department of Aerospace and Ocean Engineering, Virginia Tech, Blacksburg, VA24060, USA
*
†Email address for correspondence: devenport@vt.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

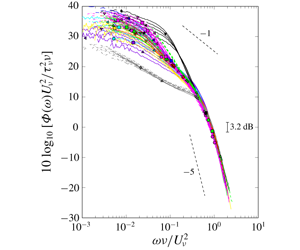
Experiments were carried out in high Reynolds number turbulent boundary layers over rough surfaces of diverse geometries. Roughness configurations varied in element height, distribution (random versus ordered), shape and spacing. Rough surfaces comprising of two superposed element geometries were also tested. All flows were free of transitional effects with $Re_{\unicode[STIX]{x1D703}}$ upwards of 40 000 and $\unicode[STIX]{x1D6FF}/k_{g}$ ratios above 73. The wall-pressure spectrum and turbulent velocity profiles revealed that the roughness element spacing has the greatest impact on the turbulent structures in the boundary layer. The high-frequency scaling on shear friction velocity, $U_{\unicode[STIX]{x1D708}}$, (Meyers et al.J. Fluid Mech., vol. 768, 2015, pp. 261–293) was validated and $U_{\unicode[STIX]{x1D708}}$ was shown to be the viscous contribution to the overall surface drag. An empirical formula for the pressure drag on roughness elements was developed to reflect the finding that the pressure drag is a function of only two variables: sparseness ratio $(\unicode[STIX]{x1D706})$ and roughness Reynolds number $(k_{g}^{+})$. Results also suggest that the viscous contribution to drag approaches a constant non-zero value at high Reynolds numbers, and ‘fully rough-wall flow’ may occur at higher $k_{g}^{+}$ than previously thought.

JFM classification

Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulence theory
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 883 , 25 January 2020 , A3
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antonia, R. A. & Krogstad, P. Å. 2001 Turbulence structure in boundary layers over different types of surface roughness. Fluid Dyn. Res. 28 (2), 139–157.CrossRefGoogle Scholar
Aupperle, F. A. & Lambert, R. F. 1970 Effects of roughness on measured wall-pressure fluctuations beneath a turbulent boundary layer. J. Acoust. Soc. Am. 47 (1), 359–370.CrossRefGoogle Scholar
Awasthi, M.2012 High Reynolds number turbulent boundary layer flow over small forward facing steps. Thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
Balachandar, R. & Blakely, D. 2004 Surface roughness effects on turbulent boundary layers on a flat plate located in an open channel. J. Hydraul Res. 42 (3), 247–261.Google Scholar
Balantrapu, N. A., Repasky, R. J., Joseph, L. A. & Devenport, W. J. 2018 The dynamic response of a pinhole microphone under flows of varying shear stress. In 2018 AIAA/CEAS Aeroacoustics Conference.Google Scholar
Bennington, J. L.2004 Effects of various shaped roughness elements in two-dimensional high Reynolds number turbulent boundary layers. PhD thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
Bhaganagara, K., Coleman, G. & Kim, J. 2007 Effect of roughness on pressure fluctuations in a turbulent channel flow. Phys. Fluids 19 (2), 028103.Google Scholar
Blake, W. 1970 Turbulent boundary-layer wall-pressure fluctuations on smooth and rough walls. J. Fluid Mech. 44 (4), 637–660.CrossRefGoogle Scholar
Blake, W. K. 2017 Chapter 2 – essentials of turbulent wall pressure fluctuations. In Mechanics of Flow-Induced Sound and Vibration, 2nd edn. (ed. Blake, William K.), vol. 2, pp. 81–177. Academic Press.CrossRefGoogle Scholar
Choi, H. & Moin, P. 1990 On the space-time characteristics of wall-pressure fluctuations. Phys. Fluids 2 (8), 1450–1460.CrossRefGoogle Scholar
De Graaff, D. B. & Eaton, J. K. 2000 Reynolds-number scaling of the flat-plate turbulent boundary layer. J. Fluid Mech. 422, 319–346.CrossRefGoogle Scholar
Devenport, W. J., Burdisso, R. A., Borgoltz, A., Ravetta, P. A., Barone, M. F., Brown, K. A. & Morton, M. A. 2013 The Kevlar-walled anechoic wind tunnel. J. Sound Vib. 332 (17), 3971–3991.Google Scholar
Devenport, W. J., Grissom, D. L., Nathan Alexander, W., Smith, B. S. & Glegg, S. A. L. 2011 Measurements of roughness noise. J. Sound Vib. 330 (17), 4250–4273.Google Scholar
Dvorak, F. A. 1969 Calculation of turbulent boundary layers on rough surfaces in pressure gradient. AIAA J. 7 (9), 1752–1759.CrossRefGoogle Scholar
Farabee, T. M. & Casarella, M. J. 1991 Spectral features of wall pressure fluctuations beneath turbulent boundary layers. Phys. Fluids A 3 (10), 2410–2420.CrossRefGoogle Scholar
Fernholz, H. H. & Finley, P. J. 1996 The incompressible zero-pressure-gradient turbulent boundary layer: An assessment of the data. Prog. Aerosp. Sci. 32 (4), 245–311.CrossRefGoogle Scholar
Flack, K. A., Schultz, M. P. & Shapiro, T. A. 2005 Experimental support for townsends Reynolds number similarity hypothesis on rough walls. Phys. Fluids 17 (3), 035102.CrossRefGoogle Scholar
Fontaine, A. A. & Deutsch, S. 1996 Structure of near wall turbulence downstream of a wall mounted protrusion: an interesting Reynolds stress suppression phenomena. Exp. Fluids 20 (5), 365–376.CrossRefGoogle Scholar
Forest, J. B.2012 The wall pressure spectrum of high Reynolds number rough-wall turbulent boundary layers. Master’s thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
Forest, J. B. & Devenport, W.2011 The wall pressure spectrum of high Reynolds number rough-wall turbulent boundary layers. In 17th AIAA/CEAS Aeroacoustics Conference, Portland, Oregon.CrossRefGoogle Scholar
George, J.2005 Structure of 2-d and 3-d turbulent boundary layers with sparsely distributed roughness elements. PhD thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
George, J. & Simpson, R. 2000 Some Effects of Sparsely Distributed Three-dimensional Roughness Elements on Two-dimensional Turbulent Boundary Layers. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Goody, M. 2004 Empirical spectral model of surface pressure fluctuations. AIAA J. 42 (9), 1788–1794.CrossRefGoogle Scholar
Goody, M. C. & Simpson, R. L.1999 An experimental investigation of pressure fluctuations in three-dimensional turbulent boundary layers. Tech. Rep. DTIC Document.CrossRefGoogle Scholar
Grass, A. J. 1971 Structural features of turbulent flow over smooth and rough boundaries. J. Fluid Mech. 50 (2), 233–255.CrossRefGoogle Scholar
Gravante, S. P., Naguib, A. M., Wark, C. E. & Nagib, H. M. 1998 Characterization of the pressure fluctuations under a fully developed turbulent boundary layer. AIAA J. 36 (10), 1808–1816.CrossRefGoogle Scholar
Hopkins, A.2010 Fluid dynamics and surface pressure fluctuations of two-dimensional turbulent boundary layers over densely distributed surface roughness. PhD thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
Jimenez, J. 2004 Turbulent flows over rough walls. Annu. Rev. Fluid Mech. 36 (1), 173–196.CrossRefGoogle Scholar
Joseph, L. A.2017 Pressure fluctuations in a high-Reynolds-number turbulent boundary layer over rough surfaces of different configurations. PhD thesis, Virginia Tech.CrossRefGoogle Scholar
Joseph, L. A., Meyers, T. W., Molinaro, N. J. & Devenport, W. J. 2016 Pressure fluctuations in a high-Reynolds-number turbulent boundary layer flow over rough surfaces. In 22nd AIAA Aeroacoustics Conference.Google Scholar
Joseph, L. A., Molinaro, N. J. & Devenport, W. J.2019 Rough Wall Boundary Layer Measurements to Accompany the paper ‘Characteristics of the Pressure Fluctuations Generated in Turbulent Boundary Layers over Rough Surfaces. University Libraries, Virginia Tech.CrossRefGoogle Scholar
Klewicki, J. C. 2013 Self-similar mean dynamics in turbulent wall flows. J. Fluid Mech. 718, 596–621.CrossRefGoogle Scholar
Klewicki, J. C., Priyadarshana, P. J. A. & Metzger, M. M. 2008 Statistical structure of the fluctuating wall pressure and its in-plane gradients at high Reynolds number. J. Fluid Mech. 609, 195–220.CrossRefGoogle Scholar
Krogstad, P. A., Antonia, R. A. & Browne, L. W. B. 1992 Comparison between rough-wall and smooth-wall turbulent boundary-layers. J. Fluid Mech. 245, 599–617.CrossRefGoogle Scholar
Lee, Y., Blake, W. K. & Farabee, T.2005 Prediction of wall pressure spectrum using a rans calculation. In 43rd AIAA Aerospace Sciences Meeting and Exhibit, AIAA Paper 2005-2802.Google Scholar
Leonardi, S., Orlandi, P., Smalley, R. J., Djenidi, L. & Antonia, R. A. 2003 Direct numerical simulations of turbulent channel flow with transverse square bars on one wall. J. Fluid Mech. 491, 229–238.CrossRefGoogle Scholar
Ligrani, P. M. & Bradshaw, P. 1987 Spatial resolution and measurement of turbulence in the viscous sublayer using subminiature hot-wire probes. Exp. Fluids 5 (6), 407–417.CrossRefGoogle Scholar
Ligrani, P. M. & Moffat, R. J. 1986 Structure of transitionally rough and fully rough turbulent boundary layers. J. Fluid Mech. 162 (1), 69–98.CrossRefGoogle Scholar
Marusic, I., Monty, J. P., Hultmark, M. & Smits, A. J. 2013 On the logarithmic region in wall turbulence. J. Fluid Mech. 716, R3.CrossRefGoogle Scholar
McGrath, B. E. & Simpson, R.1987 Some features of surface pressure fluctuations in turbulent boundary layers with zero and favorable pressure gradients. NASA Tech. Rep.Google Scholar
Mehdi, F., Klewicki, J. C. & White, C. M. 2010 Mean momentum balance analysis of rough-wall turbulent boundary layers. Physica D 239 (14), 1329–1337.CrossRefGoogle Scholar
Mehdi, F., Klewicki, J. C. & White, C. M. 2013 Mean force structure and its scaling in rough-wall turbulent boundary layers. J. Fluid Mech. 731, 682–712.CrossRefGoogle Scholar
Meyers, T., Forest, J. B. & Devenport, W. J. 2015 The wall-pressure spectrum of high-Reynolds-number turbulent boundary-layer flows over rough surfaces. J. Fluid Mech. 768, 261–293.CrossRefGoogle Scholar
Meyers, T. W.2014 The rough wall high Reynolds number turbulent boundary layer surface pressure spectrum. Master’s thesis, Virginia Polytechnic Institute and State University.Google Scholar
Mish, P. F.2003 An experimental investigation of unsteady surface pressure on single and multiple airfoils. PhD thesis, Virginia Polytechnic Institute and State University.Google Scholar
Nikuradse, J.1950 Laws of flow in rough pipes. Tech. Mem. 1292 National Advisory Committee for Aeronautics.Google Scholar
Oweis, G. F., Winkel, E. S., Cutbrith, J. M., Ceccio, S. L., Perlin, M. & Dowling, D. R. 2010 The mean velocity profile of a smooth-flat-plate turbulent boundary layer at high Reynolds number. J. Fluid Mech. 665, 357–381.CrossRefGoogle Scholar
Panton, R., Goldman, A. L., Lowery, R. I. & Reischman, M. M. 1980 Low-frequency pressure fluctuations in axisymmetric boundary layers. J. Fluid Mech. 97 (2), 299–319.CrossRefGoogle Scholar
Panton, R. & Linebarger, J. H. 1974 Wall pressure spectra calculations for equilibrium boundary layers. J. Fluid Mech. 65 (2), 261–287.CrossRefGoogle Scholar
Raupach, M. R. 1981 Conditional statistics of Reynolds stress in rough-wall and smooth-wall turbulent boundary layers. J. Fluid Mech. 108 (1), 363–382.CrossRefGoogle Scholar
Raupach, M. R., Antonia, R. A. & Rajagopalan, S. 1991 Rough-wall turbulent boundary layers. Appl. Mech. Rev. 44 (1), 1–25.CrossRefGoogle Scholar
Raupach, M. R., Thom, A. S. & Edwards, I. 1980 A wind-tunnel study of turbulent flow close to regularly arrayed rough surfaces. Boundary-Layer Meteorol. 18 (4), 373–397.CrossRefGoogle Scholar
Rusche, M. T. & Simpson, R. L. 2012 Structure of Turbulent Boundary Layers and Surface Pressure Fluctuations on a Patch of Roughness Elements. American Institute of Aeronautics and Astronautics Inc.CrossRefGoogle Scholar
Schlichting, H. 1979 Boundary-Layer Theory, 7th edn. McGraw-Hill.Google Scholar
Schultz, M. P. & Flack, K. A. 2007 The rough-wall turbulent boundary layer from the hydraulically smooth to the fully rough regime. J. Fluid Mech. 580, 381–405.CrossRefGoogle Scholar
Schultz, M. P. & Flack, K. A. 2009 Turbulent boundary layers on a systematically varied rough wall. Phys. Fluids 21 (1), 015104.CrossRefGoogle Scholar
Simpson, R. L. 1970 Characteristics of turbulent boundary layers at low Reynolds numbers with and without transpiration. J. Fluid Mech. 42 (4), 769–802.CrossRefGoogle Scholar
Simpson, R. L. 1973 A generalized correlation of roughness density effects on the turbulent boundary layer. AIAA J. 11 (2), 242–244.CrossRefGoogle Scholar
Smith, B. S.2008 Wall jet boundary layer flows over smooth and rough surfaces. PhD thesis, Virginia Polytechnic Institute and State University.Google Scholar
Smol’yakov, A. V. 2000 Calculation of the spectra of psuedosound wall-pressure fluctuations in turbulent boundary layers. Acoust. Phys. 46 (3), 342–347.CrossRefGoogle Scholar
Squire, D. T., Morrill-Winter, C., Hutchins, N., Schultz, M. P., Klewicki, J. C. & Marusic, I. 2016 Comparison of turbulent boundary layers over smooth and rough surfaces up to high Reynolds numbers. J. Fluid Mech. 795, 210–240.CrossRefGoogle Scholar
Sreenivasan, K. R. 1989 The Turbulent Boundary Layer. pp. 159–209. Springer.Google Scholar
Stewart, D. O.2005 Effects of spacing and geometry of distributed roughness elements on a two-dimensional turbulent boundary layer. Master’s thesis, Virginia Polytechnic Institute and State University.CrossRefGoogle Scholar
Tomkins, C. D.2001 The structure of turbulence over smooth and rough walls. PhD thesis, Virginia Polytechnic and State University.Google Scholar
Varano, N. D.2010 Fluid dynamics and surface pressure fluctuations of turbulent boundary layers over sparse roughness. PhD thesis, Virginia Polytechnic Institute and State University.Google Scholar
Varano, N. D. & Simpson, R. L. 2009 Flow Structure and Pressure Fluctuations of Turbulent Boundary Layers with Sparse Roughness. American Institute of Aeronautics and Astronautics Inc.CrossRefGoogle Scholar
Waigh, D. R. & Kind, R. J. 1998 Improved aerodynamic characterization of regular three-dimensional roughness. AIAA J. 36 (6), 1117–1119.CrossRefGoogle Scholar
Wittmer, K. S., Devenport, W. J. & Zsoldos, J. S. 1998 A four-sensor hot-wire probe system for three-component velocity measurement. Exp. Fluids 24 (5-6), 416–423.CrossRefGoogle Scholar
Womack, K. M. P., Schultz, M. & Meneveau, C. 2019 Outer-layer differences in boundary layer flow over surfaces with regular and random arrangements. In 11th International Symposium on Turbulence and Shear Flow Phenomena (TSFP11), Southampton, UK.Google Scholar
Yang, Q. & Wang, M. 2010 Boundary-layer noise induced by a roughness patch. In 16th AIAA/CEAS Aeroacoustics Conference.Google Scholar
Yang, Q. & Wang, M. 2011 Statistical analysis of acoustic-source field in rough-wall boundary layers. In 17th AIAA/CEAS Aeroacoustics Conference, Portland, Oregon.Google Scholar
Yang, Q. & Wang, M. 2013 Boundary-layer noise induced by arrays of roughness elements. J. Fluid Mech. 727, 282–317.CrossRefGoogle Scholar
Zhang, X., Pan, C., Shen, J. & Wang, J. 2015 Effect of surface roughness element on near wall turbulence with zero-pressure gradient. SCIENCE CHINA Phys. Mech. Astron. 58 (6), 1–8.Google Scholar