Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Mazzuoli, Marco
and
Uhlmann, Markus
2017.
Direct numerical simulation of open-channel flow over a fully rough wall at moderate relative submergence.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
722.
Wang, Yayun
and
Prosperetti, Andrea
2018.
Heat transfer from an array of resolved particles in turbulent flow.
Physical Review Fluids,
Vol. 3,
Issue. 8,
Gorokhovski, Mikhael
and
Zamansky, Rémi
2018.
Modeling the effects of small turbulent scales on the drag force for particles below and above the Kolmogorov scale.
Physical Review Fluids,
Vol. 3,
Issue. 3,
Dombrovsky, Leonid A.
Dembele, Siaka
and
Wen, Jennifer X.
2018.
An infrared scattering by evaporating droplets at the initial stage of a pool fire suppression by water sprays.
Infrared Physics & Technology,
Vol. 91,
Issue. ,
p.
55.
Jang, Juwon
and
Lee, Changhoon
2018.
Modification of turbulence and stratification of stably stratified turbulent channel flows by finite-size particles.
Physical Review Fluids,
Vol. 3,
Issue. 12,
Rahmani, M.
Geraci, G.
Iaccarino, G.
and
Mani, A.
2018.
Effects of particle polydispersity on radiative heat transfer in particle-laden turbulent flows.
International Journal of Multiphase Flow,
Vol. 104,
Issue. ,
p.
42.
Lalescu, Cristian C.
and
Wilczek, Michael
2018.
Acceleration statistics of tracer particles in filtered turbulent fields.
Journal of Fluid Mechanics,
Vol. 847,
Issue. ,
Peng, Cheng
Ayala, Orlando M.
and
Wang, Lian-Ping
2019.
A direct numerical investigation of two-way interactions in a particle-laden turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 875,
Issue. ,
p.
1096.
Chouippe, Agathe
and
Uhlmann, Markus
2019.
On the influence of forced homogeneous-isotropic turbulence on the settling and clustering of finite-size particles.
Acta Mechanica,
Vol. 230,
Issue. 2,
p.
387.
Mazzuoli, Marco
Kidanemariam, Aman G.
and
Uhlmann, Markus
2019.
Direct numerical simulations of ripples in an oscillatory flow.
Journal of Fluid Mechanics,
Vol. 863,
Issue. ,
p.
572.
Del Grosso, Nicolás F.
Cappelletti, Lucía M.
Sujovolsky, Nicolás E.
Mininni, Pablo D.
and
Cobelli, Pablo J.
2019.
Statistics of single and multiple floaters in experiments of surface wave turbulence.
Physical Review Fluids,
Vol. 4,
Issue. 7,
Ernst, Martin
Sommerfeld, Martin
and
Laín, Santiago
2019.
Quantification of preferential concentration of colliding particles in a homogeneous isotropic turbulent flow.
International Journal of Multiphase Flow,
Vol. 117,
Issue. ,
p.
163.
Uhlmann, Markus
2020.
Voronoï tessellation analysis of sets of randomly placed finite-size spheres.
Physica A: Statistical Mechanics and its Applications,
Vol. 555,
Issue. ,
p.
124618.
Horwitz, J. A. K.
and
Mani, A.
2020.
Two-way coupled particle-turbulence interaction: Effect of numerics and resolution on fluid and particle statistics.
Physical Review Fluids,
Vol. 5,
Issue. 10,
Ng, Chong Shen
Spandan, Vamsi
Verzicco, Roberto
and
Lohse, Detlef
2020.
Non-monotonic transport mechanisms in vertical natural convection with dispersed light droplets.
Journal of Fluid Mechanics,
Vol. 900,
Issue. ,
Vessaire, Jérémy
Plihon, Nicolas
Volk, Romain
and
Bourgoin, Mickaël
2020.
Sedimentation of a suspension of paramagnetic particles in an external magnetic field.
Physical Review E,
Vol. 102,
Issue. 2,
Xia, Yan
Xiong, Hongbing
Yu, Zhaosheng
and
Zhu, Chenlin
2020.
Effects of the collision model in interface-resolved simulations of particle-laden turbulent channel flows.
Physics of Fluids,
Vol. 32,
Issue. 10,
Bagheri, Meysam
and
Sabzpooshani, Majid
2020.
On the importance of the history force in dispersion of particles in von Kármán vortex street.
Advanced Powder Technology,
Vol. 31,
Issue. 9,
p.
3897.
Shen, Jie
Lu, Zhiming
Wang, Lian-Ping
and
Peng, Cheng
2021.
Influence of particle-fluid density ratio on the dynamics of finite-size particles in homogeneous isotropic turbulent flows.
Physical Review E,
Vol. 104,
Issue. 2,
Oka, Sunao
and
Goto, Susumu
2021.
Generalized sweep-stick mechanism of inertial-particle clustering in turbulence.
Physical Review Fluids,
Vol. 6,
Issue. 4,
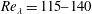 $Re_{\unicode[STIX]{x1D706}}=115{-}140$. The solid–fluid density ratio was set to
$Re_{\unicode[STIX]{x1D706}}=115{-}140$. The solid–fluid density ratio was set to 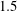 $1.5$, gravity was set to zero and two particle diameters were investigated corresponding to approximately
$1.5$, gravity was set to zero and two particle diameters were investigated corresponding to approximately 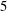 $5$ and
$5$ and 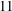 $11$ Kolmogorov lengths. Note that these particle sizes are clearly outside the range of validity of the point-particle approximation, as has been shown by Homann & Bec (J. Fluid Mech., vol. 651, 2010, pp. 81–91). At the present parameter points the global effect of the particles upon the fluid flow is weak. We observe that the dispersed phase exhibits clustering with moderate intensity. The tendency to cluster, which was quantified in terms of the standard deviation of Voronoï cell volumes, decreases with the particle diameter. We have analysed the relation between particle locations and the location of intense vortical flow structures. The results do not reveal any significant statistical correlation. Contrarily, we have detected a small but statistically significant preferential location of particles with respect to the ‘sticky points’ proposed by Goto & Vassilicos (Phys. Rev. Lett., vol. 100 (5), 2008, 054503), i.e. points where the fluid acceleration field is acting such as to increase the local particle concentration in one-way coupled point-particle models under Stokes drag. The presently found statistical correlation between the ‘sticky points’ and the particle locations further increases when focusing on regions with high local concentration. Our results suggest that small finite-size particles can be brought together along the expansive directions of the fluid acceleration field, as previously observed only for the simplest model for sub-Kolmogorov particles. We further discuss the effect of density ratio and collective particle motion upon the basic Eulerian and Lagrangian statistics.
$11$ Kolmogorov lengths. Note that these particle sizes are clearly outside the range of validity of the point-particle approximation, as has been shown by Homann & Bec (J. Fluid Mech., vol. 651, 2010, pp. 81–91). At the present parameter points the global effect of the particles upon the fluid flow is weak. We observe that the dispersed phase exhibits clustering with moderate intensity. The tendency to cluster, which was quantified in terms of the standard deviation of Voronoï cell volumes, decreases with the particle diameter. We have analysed the relation between particle locations and the location of intense vortical flow structures. The results do not reveal any significant statistical correlation. Contrarily, we have detected a small but statistically significant preferential location of particles with respect to the ‘sticky points’ proposed by Goto & Vassilicos (Phys. Rev. Lett., vol. 100 (5), 2008, 054503), i.e. points where the fluid acceleration field is acting such as to increase the local particle concentration in one-way coupled point-particle models under Stokes drag. The presently found statistical correlation between the ‘sticky points’ and the particle locations further increases when focusing on regions with high local concentration. Our results suggest that small finite-size particles can be brought together along the expansive directions of the fluid acceleration field, as previously observed only for the simplest model for sub-Kolmogorov particles. We further discuss the effect of density ratio and collective particle motion upon the basic Eulerian and Lagrangian statistics.