Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-13T15:57:28.290Z Has data issue: false hasContentIssue false
  • English
  • Français

Coins falling in water

Published online by Cambridge University Press:  21 February 2014

Luke Heisinger ,
Paul Newton  and
Eva Kanso
Luke Heisinger
Affiliation:
University of Southern California, Los Angeles, CA 90089, USA
Paul Newton
Affiliation:
University of Southern California, Los Angeles, CA 90089, USA
Eva Kanso*
Affiliation:
University of Southern California, Los Angeles, CA 90089, USA
*
†Email address for correspondence: kanso@usc.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

When a coin falls in water, its trajectory is one of four types, determined by its dimensionless moment of inertia 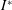$I^\ast $ and Reynolds number 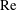$\text {Re}$: (A) steady; (B) fluttering; (C) chaotic; or (D) tumbling. The dynamics induced by the interaction of the water with the surface of the coin, however, makes the exact landing site difficult to predict a priori. Here, we describe a carefully designed experiment in which a coin is dropped repeatedly in water to determine the probability density functions (p.d.f.s) associated with the landing positions for each of the four trajectory types, all of which are radially symmetric about the centre drop-line. In the case of the steady mode, the p.d.f. is approximately Gaussian distributed with small variances, indicating that the coin is most likely to land at the centre, right below the point from which it is dropped. For the other falling modes, the centre is one of the least likely landing sites. Indeed, the p.d.f.s of the fluttering, chaotic and tumbling modes are characterized by a ‘dip’ around the centre. In the tumbling mode, the p.d.f. is a ring configuration about the centreline whereas in the chaotic mode, the p.d.f. is generally a broadband distribution spread out radially symmetrically about the centreline. For the steady and fluttering modes, the coin never flips, so the coin lands with the same side up as when it was dropped. The probability of heads or tails is close to 0.5 for the chaotic mode and, in the case of the tumbling mode, the probability of heads or tails is based on the height of the drop which determines whether the coin flips an even or odd number of times during descent.

JFM classification

Aerodynamics: Aerodynamics Aerodynamics: Flow-structure interactions Nonlinear Dynamical Systems: Nonlinear Dynamical Systems
Type
Papers
Information
Journal of Fluid Mechanics , Volume 742 , 10 March 2014 , pp. 243 - 253
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

The original version of this article was published with the incorrect order of authors. A notice detailing this has been published and the error rectified in the online and print PDF and HTML copies.

References

Alben, S. 2010 Flexible sheets falling in an inviscid fluid. Phys. Fluids 22, 061901.Google Scholar
Andersen, A., Pesavento, U. & Wang, Z. J. 2005a Unsteady aerodynamics of fluttering and tumbling plates. J. Fluid Mech. 541, 65–90.Google Scholar
Andersen, A., Pesavento, U. & Wang, Z. J. 2005b Analysis of transitions between fluttering, tumbling and steady descent of falling cards. J. Fluid Mech. 541, 91–104.Google Scholar
Auguste, F., Magnaudet, J. & Fabre, D. 2013 Falling styles of disks. J. Fluid Mech. 719, 388–405.CrossRefGoogle Scholar
Belmonte, A., Eisenberg, H. & Moses, E. 1998 From flutter to tumble: inertial drag and Froude similarity in falling paper. Phys. Rev. Lett. 81, 345–348.CrossRefGoogle Scholar
Diaconis, P., Holmes, S. & Montgomery, R. 2007 Dynamical bias in the coin toss. SIAM Rev. 49, 211–235.CrossRefGoogle Scholar
Dupleich, P. 1941 Rotation in free fall of rectangular wings of elongated shape. NACA Tech. Mem. 1201, 1–99.Google Scholar
Ern, P., Risso, F., Fabre, D. & Magnaudet, J. 2012 Wake-induced oscillatory paths of bodies freely rising or falling in fluids. Annu. Rev. Fluid Mech. 44, 97–121.CrossRefGoogle Scholar
Field, S. B., Klaus, M., Moore, M. G. & Nori, F. 1997 Chaotic dynamics of falling disks. Nature 388, 252–254.CrossRefGoogle Scholar
Hopf, E. 1934 On causality, statistics and probability. J. Math. Phys. 13, 51–102.CrossRefGoogle Scholar
Howe, H. F. & Smallwood, J. 1982 Ecology of seed dispersal,. Ann. Rev. Ecol. Syst. 3, 201–228.CrossRefGoogle Scholar
Jones, M. A. 2003 The separated flow of an inviscid fluid around a moving flat plate. J. Fluid Mech. 496, 405–441.CrossRefGoogle Scholar
Jones, M. A. & Shelley, M. J. 2005 Falling cards. J. Fluid Mech. 540, 393–425.Google Scholar
Keller, J. B. 1986 The probability of heads. Am. Math. Mon. 93, 191–197.Google Scholar
Mahadevan, L. 1996 Tumbling of a falling card. C. R. Acad. Sci. Paris II 323, 729–736.Google Scholar
Mahadevan, L., Ryu, W. S. & Samuel, A. D. T. 1999 Tumbling cards. Phys. Fluids 11 (1), 1–3.CrossRefGoogle Scholar
Maxwell, J. C. 1854 On a particular case of the descent of a heavy body in a resisting medium. Camb. Dublin Math. J. 9, 145–148.Google Scholar
Michelin, S. & Smith, S. G. L. 2009 An unsteady point vortex method for coupled fluid-solid problems. Theor. Comput. Fluid Dyn. 23, 127–153.CrossRefGoogle Scholar
Mittal, R., Seshadri, V. & Udaykumar, H. S. 2004 Flutter, tumble, and vortex induced autorotation. Theor. Comput. Fluid Dyn. 17, 165–170.Google Scholar
Nathan, R. & Muller-Landau, H. C. 2000 Spatial patterns of seed dispersal, their determinants and consequences for recruitment. Trends Ecol. Evol. 15 (7), 278–285.CrossRefGoogle ScholarPubMed
Paoletti, P. & Mahadevan, L. 2011 Planar controlled gliding, tumbling and descent. J. Fluid Mech. 689, 489–516.Google Scholar
Pesavento, U. & Wang, Z. J. 2004 Falling paper: Navier–Stokes solutions, model of fluid forces, and center of mass elevation. Phys. Rev. Lett. 93 (14), 144501.Google Scholar
Poincaré, H.(Ed.) 1912 Calcul des probabilités. Gauthier-Villars.Google Scholar
Smith, H. E. 1971 Autorotating wings: an experimental investigation. J. Fluid Mech. 50, 513–534.CrossRefGoogle Scholar
Tam, D., Bush, J. W. M., Robitaille, M. & Kudrolli, A. 2010 Tumbling Dynamics of Passive Flexible Wings. Phys. Rev. Lett. 104 (18), 184504.Google Scholar
Tanabe, Y. & Kaneko, K. 1994 Behavior of a falling paper. Phys. Rev. Lett. 73 (10), 1372–1375.CrossRefGoogle ScholarPubMed
Willmarth, W. W., Hawk, N. E. & Harvey, R. L. 1964 Steady and unsteady motions and wakes of freely falling disks. Phys. Fluids 7 (2), 197–208.CrossRefGoogle Scholar
Yong, E. H. & Mahadevan, L. 2011 Probability, geometry and dynamics in the toss of a thick coin. Am. J. Phys. 79 (12), 1195–1201.CrossRefGoogle Scholar
Zheng, L. & Jing, W.2006 Application of predictive guidance to re-entry vehicles. 1st International Symposium on Systems Control in Aerospace and Astronautics (ISSCAA 2006) 659–663.Google Scholar