Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-01-14T16:57:00.716Z Has data issue: false hasContentIssue false
  • English
  • Français

The connection between the spectrum of turbulent scales and the skin-friction statistics in channel flow at 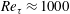$Re_{\unicode[STIX]{x1D70F}}\approx 1000$

Published online by Cambridge University Press:  17 May 2019

Lionel Agostini Open the ORCID record for Lionel Agostini [Opens in a new window]  and
Michael Leschziner Open the ORCID record for Michael Leschziner [Opens in a new window]
Lionel Agostini*
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
Michael Leschziner
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: l.agostini@imperial.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

Data from a direct numerical simulation for channel flow at a friction Reynolds number of 1000 are analysed to derive statistical properties that offer insight into the mechanisms by which large-scale structures in the log-law region affect the small-scale turbulence field close to the wall and the statistical skin-friction properties. The data comprise full-volume velocity fields at 150 time levels separated by 50 wall-scaled viscous time units. The scales are separated into wavelength bands by means of the ‘empirical mode decomposition’, of which the two lowest modes are considered to represent the small scales and three upper modes to represent the large scales. Joint and conditional probability density functions are then derived for various scale-specific statistics, with particular emphasis placed on the streamwise and shear stresses conditional on the large-scale fluctuations of the skin friction, generally referred to as ‘footprinting’. Statistics for the small-scale stresses, conditional on the footprints, allow the amplification and attenuation of the small-scale skin friction, generally referred to as ‘modulation’, to be quantified in dependence on the footprints. The analysis leads to the conclusion that modulation does not reflect a direct interaction between small scales and large scales, but arises from variations in shear-induced production that arise from corresponding changes in the conditional velocity profile. This causal relationship also explains the wall-normal change in sign in the correlation between large scales and small scales at a wall-scaled wall distance of approximately 100. The effects of different scales on the skin friction are investigated by means of two identities that describe the relationship between the shear-stress components and the skin friction, one identity based on integral momentum and the other on energy production/dissipation. The two identities yield significant differences in the balance of scale-specific contributions, and the origins of these differences are discussed.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 871 , 25 July 2019 , pp. 22 - 51
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agostini, L. & Leschziner, M. 2014 On the influence of outer large-scale structures on near-wall turbulence in channel flow. Phys. Fluids 26 (7), 075107.Google Scholar
Agostini, L. & Leschziner, M. 2016a On the validity of the quasi-steady-turbulence hypothesis in representing the effects of large scales on small scales in boundary layers. Phys. Fluids 28 (4), 045102.Google Scholar
Agostini, L. & Leschziner, M. 2016b Predicting the response of small-scale near-wall turbulence to large-scale outer motions. Phys. Fluids 28 (1), 015107.Google Scholar
Agostini, L. & Leschziner, M. 2017 Spectral analysis of near-wall turbulence in channel flow at Re 𝜏 = 4200 with emphasis on the attached-eddy hypothesis. Phys. Rev. Fluids 2, 014603.Google Scholar
Agostini, L. & Leschziner, M. 2018 The impact of footprints of large-scale outer structures on the near-wall layer in the presence of drag-reducing spanwise wall motion. Flow Turbul. Combust. 100, 1037–1061.Google Scholar
Agostini, L., Leschziner, M. & Gaitonde, D. 2016 Skewness-induced asymmetric modulation of small-scale turbulence by large-scale structures. Phys. Fluids 28 (1), 015110.Google Scholar
Agostini, L., Touber, E. & Leschziner, M. 2014 Spanwise oscillatory wall motion in channel flow: drag-reduction mechanisms inferred from DNS-predicted phase-wise property variations at Re 𝜏 = 1000. J. Fluid Mech. 743, 606–635.Google Scholar
Baars, W. J., Hutchins, N. & Marusic, I. 2016 Spectral stochastic estimation of high-Reynolds-number wall-bounded turbulence for a refined inner–outer interaction model. Phys. Rev. Fluids 1, 054406.Google Scholar
Baars, W. J. & Marusic, I.2018 Data-driven decomposition of the streamwise turbulence kinetic energy in boundary layers. Part 1. Energy spectra. arXiv:1810.03100.Google Scholar
Baars, W. J., Talluru, K. M., Hutchins, N. & Marusic, I. 2015 Wavelet analysis of wall turbulence to study large-scale modulation of small scales. Exp. Fluids 56 (10), 1–15.Google Scholar
Baidya, R., Philip, J., Hutchins, N., Monty, J. P. & Marusic, I. 2017 Distance-from-the-wall scaling of turbulent motions in wall-bounded flows. Phys. Fluids 29 (2), 020712.Google Scholar
Bandyopadhyay, P. R. & Hussain, A. 1984 The coupling between scales in shear flows. Phys. Fluids 27 (9), 2221–2228.Google Scholar
Bernardini, M. & Pirozzoli, S. 2011 Inner/outer layer interactions in turbulent boundary layers: a refined measure for the large-scale amplitude modulation mechanism. Phys. Fluids 23 (6), 061701.Google Scholar
Blackwelder, R. F. & Kovasznay, L. S. G. 1972 Time scales and correlations in a turbulent boundary layer. Phys. Fluids 15 (9), 1545–1554.Google Scholar
Bradshaw, P. 1967 ‘Inactive’ motion and pressure fluctuations in turbulent boundary layers. J. Fluid Mech. 30 (2), 241–258.Google Scholar
Brown, G. L. & Thomas, A. S. W. 1977 Large structure in a turbulent boundary layer. Phys. Fluids 20 (10), S243–S252.Google Scholar
Chandran, D., Baidya, R., Monty, J. P. & Marusic, I. 2017 Two-dimensional energy spectra in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 826, R1.Google Scholar
Chung, D., Marusic, I., Monty, J. P., Vallikivi, M. & Smits, A. J. 2015 On the universality of inertial energy in the log layer of turbulent boundary layer and pipe flows. Exp. Fluids 56 (7), 1–10.Google Scholar
Cormier, M., Gatti, D. & Frohnapfel, B. 2016 Interaction between inner and outer layer in drag-reduced turbulent flows. Proc. Appl. Maths Mech. 16 (1), 633–634.Google Scholar
Davidson, P. A., Krogstad, P-A. & Nickels, T. B. 2006 A refined interpretation of the logarithmic structure function law in wall layer turbulence. Phys. Fluids 18 (6), 065112.Google Scholar
Del Alamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.Google Scholar
Del Álamo, J. C., Jimenez, J., Zandonade, P. & Moser, R. D. 2006 Self-similar vortex clusters in the turbulent logarithmic region. J. Fluid Mech. 561, 329–358.Google Scholar
Dennis, D. & Nickels, T. 2011 Experimental measurement of large-scale three-dimensional structures in a turbulent boundary layer. Part 2. Long structures. J. Fluid Mech. 673, 218–244.Google Scholar
Dróżdż, A. & Elsner, W. 2017 Amplitude modulation and its relation to streamwise convection velocity. Intl J. Heat Fluid Flow 63, 67–74.Google Scholar
Duvvuri, S. & McKeon, B. J. 2015 Triadic scale interactions in a turbulent boundary layer. J. Fluid Mech. 767, R4.Google Scholar
Fiorini, T., Bellani, G., Örlü, R., Segalini, A., Alfredsson, P. H. & Talamelli, A. 2017 Turbulent pipe flow near-wall statistics. In Progress in Turbulence VII, pp. 89–94. Springer.Google Scholar
Flandrin, P., Rilling, G. & Goncalves, P. 2004 Empirical mode decomposition as a filter bank. IEEE Signal Process. Lett. 11 (2), 112–114.Google Scholar
Flores, O. & Jiménez, J. 2010 Hierarchy of minimal flow units in the logarithmic layer. Phys. Fluids 22 (7), 071704.Google Scholar
Ganapathisubramani, B., Hutchins, N., Monty, J. P., Chung, D. & Marusic, I. 2012 Amplitude and frequency modulation in wall turbulence. J. Fluid Mech. 712, 61–91.Google Scholar
de Giovanetti, M., Hwang, Y. & Choi, H. 2016 Skin-friction generation by attached eddies in turbulent channel flow. J. Fluid Mech. 808, 511–538.Google Scholar
Guala, M., Metzger, M. & McKeon, B. J. 2011 Interactions within the turbulent boundary layer at high Reynolds number. J. Fluid Mech. 666, 573–604.Google Scholar
Howland, M. F. & Yang, X. 2018 Dependence of small-scale energetics on large scales in turbulent flows. J. Fluid Mech. 852, 641–662.Google Scholar
Huang, N. E., Shen, Z., Long, S. R., Wu, M. C., Shih, H. H., Zheng, Q., Yen, N.-C., Tung, C. C. & Liu, H. H. 1998 The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. R. Soc. Lond. A 454 (1971), 903–995.Google Scholar
Hwang, J. & Sung, H. J. 2017 Influence of large-scale motions on the frictional drag in a turbulent boundary layer. J. Fluid Mech. 829, 751–779.Google Scholar
Hwang, J. & Sung, H. J. 2018 Wall-attached structures of velocity fluctuations in a turbulent boundary layer. J. Fluid Mech. 856, 958–983.Google Scholar
Hwang, Y. & Bengana, Y. 2016 Self-sustaining process of minimal attached eddies in turbulent channel flow. J. Fluid Mech. 795, 708–738.Google Scholar
Jacobi, I. & McKeon, B. J. 2013 Phase relationships between large and small scales in the turbulent boundary layer. Exp. Fluids 54 (3), 1–13.Google Scholar
Jiménez, J. 2013 Near-wall turbulence. Phys. Fluids 25 (10), 101302.Google Scholar
Kevin, K., Monty, J. & Hutchins, N. 2019 Turbulent structures in a statistically three-dimensional boundary layer. J. Fluid Mech. 859, 543–565.Google Scholar
Lee, M. & Moser, R. D. 2015 Direct numerical simulation of turbulent channel flow up to Re 𝜏 ≈ 5200. J. Fluid Mech. 774, 395–415.Google Scholar
Lozano-Durán, A. & Jiménez, J. 2014 Effect of the computational domain on direct simulations of turbulent channels up to Re 𝜏 = 4200. Phys. Fluids 26 (1), 011702.Google Scholar
Marusic, I., Mathis, R. & Hutchins, N. 2010 High Reynolds number effects in wall turbulence. Intl J. Heat Fluid Flow 31 (3), 418–428.Google Scholar
Marusic, I. & Monty, J. P. 2019 Attached eddy model of wall turbulence. Annu. Rev. Fluid Mech. 51, 49–74.Google Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2009 Large-scale amplitude modulation of the small-scale structures in turbulent boundary layers. J. Fluid Mech. 628, 311–337.Google Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2011 A predictive inner–outer model for streamwise turbulence statistics in wall-bounded flows. J. Fluid Mech. 681, 537–566.Google Scholar
Mathis, R., Marusic, I., Chernyshenko, S. I. & Hutchins, N. 2013 Estimating wall-shear-stress fluctuations given an outer region input. J. Fluid Mech. 715, 163–180.Google Scholar
Mizuno, Y. & Jiménez, J. 2013 Wall turbulence without walls. J. Fluid Mech. 723, 429–455.Google Scholar
Moser, R. D., Kim, J. & Mansour, N. N. 1999 Direct numerical simulation of turbulent channel flow up to Re 𝜏 = 590. Phys. Fluids 11 (4), 943–945.Google Scholar
Örlü, R., Fiorini, T., Segalini, A., Bellani, G., Talamelli, A. & Alfredsson, P. H. 2017 Reynolds stress scaling in pipe flow turbulence – first results from CICLoPE. Phil. Trans. R. Soc. Lond. A 375 (2089), 20160187.Google Scholar
Perry, A. E., Henbest, S. & Chong, M. S. 1986 A theoretical and experimental study of wall turbulence. J. Fluid Mech. 165, 163–199.Google Scholar
Renard, N. & Deck, S. 2016 A theoretical decomposition of mean skin friction generation into physical phenomena across the boundary layer. J. Fluid Mech. 790, 339–367.Google Scholar
Samie, M., Marusic, I., Hutchins, N., Fu, M. K., Fan, Y., Hultmark, M. & Smits, A. J. 2018 Fully resolved measurements of turbulent boundary layer flows up to Re 𝜏 = 20 000. J. Fluid Mech. 851, 391–415.Google Scholar
de Silva, C. M., Marusic, I., Woodcock, J. D. & Meneveau, C. 2015 Scaling of second- and higher-order structure functions in turbulent boundary layers. J. Fluid Mech. 769, 654–686.Google Scholar
Srinath, S., Vassilicos, J. C., Cuvier, C., Laval, J. P., Stanislas, M. & Foucaut, J.-M. 2018 Attached flow structure and streamwise energy spectra in a turbulent boundary layer. Phys. Rev. E 97, 053103.Google Scholar
Talluru, K. M., Baidya, R., Hutchins, N. & Marusic, I. 2014 Amplitude modulation of all three velocity components in turbulent boundary layers. J. Fluid Mech. 746, R1.Google Scholar
Townsend, A. A. 1980 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Vallikivi, M., Hultmark, M. & Smits, A. J. 2015 Turbulent boundary layer statistics at very high Reynolds number. J. Fluid Mech. 779, 371–389.Google Scholar
Vassilicos, J. C., Laval, J.-P., Foucaut, J.-M. & Stanislas, M. 2015 The streamwise turbulence intensity in the intermediate layer of turbulent pipe flow. J. Fluid Mech. 774, 324–341.Google Scholar
Willert, C. E., Soria, J., Stanislas, M., Klinner, J., Amili, O., Eisfelder, M., Cuvier, C., Bellani, G., Fiorini, T. & Talamelli, A. 2017 Near-wall statistics of a turbulent pipe flow at shear Reynolds numbers up to 40 000. J. Fluid Mech. 826, R5.Google Scholar
Woodcock, J. D. & Marusic, I. 2015 The statistical behaviour of attached eddies. Phys. Fluids 27 (1), 015104.Google Scholar
Yamamoto, Y. & Tsuji, Y. 2018 Numerical evidence of logarithmic regions in channel flow at Re 𝜏 = 8000. Phys. Rev. Fluids 3 (1), 012602.Google Scholar
Yang, X. I. A. & Howland, M. F. 2018 Implication of Taylor’s hypothesis on measuring flow modulation. J. Fluid Mech. 836, 222–237.Google Scholar
Zhang, C. & Chernyshenko, S. I. 2016 Quasisteady quasihomogeneous description of the scale interactions in near-wall turbulence. Phys. Rev. Fluids 1 (1), 014401.Google Scholar