Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T07:25:52.895Z Has data issue: false hasContentIssue false
  • English
  • Français

Curved and stretched flames: the two Markstein numbers

Published online by Cambridge University Press:  28 September 2011

Paul Clavin  and
José C. Graña-Otero
Paul Clavin*
Affiliation:
Institut de Recherche sur les Phénomènes Hors Equilibre, Universités d’Aix Marseille et CNRS, 49 rue Joliot Curie, BP 146, 13384 Marseille CEDEX 13, France
José C. Graña-Otero
Affiliation:
ETSI Aeronáuticos, Universidad Politécnica de Madrid, Pl. Cardenal Cisneros 3, Madrid 28040, Spain
*
†Email address for correspondence: clavin@irphe.univ-mrs.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

The analytical result concerning the Markstein number of adiabatic flames was obtained in 1982 with the one-step Arrhenius model in the limit of a large activation energy. This result is not relevant for real flames. The form of the law expressing the flame velocity in terms of the total stretch rate of the flame front through a single Markstein length is not conserved when the location of the front (surface of zero thickness) changes within the flame thickness. 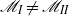It is shown in this paper that two different Markstein numbers characterize usual wrinkled flames sustained by a multiple-step chemical network, 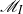for the modification of the flame velocity due to the curvature of the front and 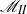for the effect of the flow strain rate. In contrast to , 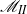depends on the location of the flame surface within the flame thickness, in such a way that the final result for the flame dynamics is not depending on this choice. The first part of the paper is devoted to present a general method of solution, valid for any multiple-step chemical network. The two Markstein numbers for two-step chain-branching models representing rich hydrogen–air flames and lean hydrocarbon–air flames are then computed analytically in the second part.

JFM classification

Reacting Flows: Flames
Type
Papers
Information
Journal of Fluid Mechanics , Volume 686 , 10 November 2011 , pp. 187 - 217
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Bechtold, J. K. & Matalon, M. 2001 The dependence of the Markstein length on stoichiometry. Combust. Flame 127, 1906–1913.CrossRefGoogle Scholar
2. Bradley, D. C., Baskell, P. H. & Gu, X. J. 1996 Burning velocities, markstein lengths, and flame quenching for spherical methane–air flames: a computational study. Combust. Flame 104, 176–198.CrossRefGoogle Scholar
3. Cambray, P. & Joulin, G. 2000 On a scaling law for coarsening celles of premixed flames: an approach to fractalization. Combust. Sci. Technol. 161, 139–164.CrossRefGoogle Scholar
4. Clavin, P. 1985 Dynamic behaviour of premixed flame fronts in laminar and turbulent flows. Prog. Energy Combust. Sci. 11, 1–59.CrossRefGoogle Scholar
5. Clavin, P. & Garcia, P. 1983 The influence of the temperature-dependence on the dynamics of flame fronts. J. Méc. Théor. Appl. 2, 245–263.Google Scholar
6. Clavin, P. & Joulin, G. 1983 Premixed flames in large scales and high intensity turbulent flow. J. Phys. Lett. 44, L1–L12.Google Scholar
7. Clavin, P. & Joulin, G. 1989 Flamelet library for turbulent wrinkled flames. In Turbulent Reactive Flows (ed. Borghi, R. & Murthy, S. N. B. ), Lecture Notes in Engineering , vol. 40, pp. 213–240. Springer.CrossRefGoogle Scholar
8. Clavin, P. & Joulin, G. 1997 High-frequency response of premixed flames to weak stretch and curvature: a variable-density analysis. Combust. Theory Model. 1, 429–446.CrossRefGoogle Scholar
9. Clavin, P. & Nicoli, C. 1985 Effects of heat Losses on the limits of stability of premixed flames propagating downwards. Combust. Flame 60, 1–14.CrossRefGoogle Scholar
10. Clavin, P. & Searby, G. 2008 Unsteady response of chain-branching premixed-flames to pressure waves. Combust. Theory Model. 12 (3), 545–567.CrossRefGoogle Scholar
11. Clavin, P. & Williams, F. A. 1982 Effects of molecular diffusion and of thermal expansion on the structure and dynamics of premixed flames in turbulent flows of large scale and low intensity. J. Fluid Mech. 116, 251–282.CrossRefGoogle Scholar
12. Creta, F., Fogla, N. & Matalon, M. 2011 Turbulent propagation of premixed flames in the presence of Darrieus–Landau instability. Combust. Theory Model. 15 (2), 267–298.CrossRefGoogle Scholar
13. Darrieus, G. 1938 Propagation d’un front de flamme. La Technique Moderne, unpublished.Google Scholar
14. Davis, S. G., Quinard, J. & Searby, G. 2002 Markstein numbers in couterflows, methane– and propane–air flames: a computational study. Combust. Flame 130, 123–136.CrossRefGoogle Scholar
15. Dold, J. W. 2007 Premixed flames modelled with thermally sensitive intermediate branching kinetics. Combust. Theory Model. 11 (6), 909–948.CrossRefGoogle Scholar
16. Frankel, M. L. & Sivashinsky, G. I. 1982 The effects of viscosity on hydrodynamic stability of a plane flame front. Combust. Sci. Technol. 29, 207–224.CrossRefGoogle Scholar
17. Garcia-Ybarra, P., Nicoli, C. & Clavin, P. 1984 Soret and dilution effects on premixed flames. Combust. Sci. Technol. 42, 87–109.CrossRefGoogle Scholar
18. He, L. & Clavin, P. 1993 Premixed hydrogen–oxygen flames part 2: quasi-isobaric ignition and flammability limits. Combust. Flame 93, 408–420.CrossRefGoogle Scholar
19. Joulin, G. & Clavin, P. 1979 Linear stability analysis of non-adiabatic flames: diffusional–thermal model. Combust. Flame 35, 139–153.CrossRefGoogle Scholar
20. Karlovitz, B., Denniston, J. R., Knapschaefer, D. H. & Wells, F. E. 1953 Studies in turbulent flames. In Proceedings of the 4th International Symposium on Combustion. The Combustion Institute, William and Wilkins.Google Scholar
21. Landau, L. 1944 On the theory of slow combustion. Acta Physicochim. USSR 19, 77–85.Google Scholar
22. Liñán, A. 1971 A theoretical analysis of premixed flame propagation with an isothermal chain reaction. AFOSR Contract No. E00AR68-0031 1. INTA Madrid.Google Scholar
23. Markstein, G. H. 1964 Nonsteady Flame Propagation. Pergamon.Google Scholar
24. Matalon, M., Cui, C. & Bechtold, J. K. 2003 Hydrodynamic theory of premixed flames: effects of stoichiometry, variable transport coefficients and arbitrary reaction orders. J. Fluid Mech. 487, 179–210.CrossRefGoogle Scholar
25. Matalon, M. & Matkowsky, B. J. 1982 Flames as gas dynamic discontinuities. J. Fluid Mech. 124, 239–259.CrossRefGoogle Scholar
26. Matkowsky, B. J. & Sivashinsky, G. I. 1979 Asymptotic derivation of two models in flame theory associated with the constant density approximation. SIAM J. Appl. Math. 37, 686–699.CrossRefGoogle Scholar
27. Nicoli, C. & Clavin, P. 1987 Effect of variable heat loss intensities on the dynamics of a premixed flame front. Combust. Flame 68, 69–71.CrossRefGoogle Scholar
28. Pelcé, P. & Clavin, P. 1982 Influence of hydrodynamics and diffusion upon the stability limits of laminar premixed flames. J. Fluid Mech. 124, 219–237.CrossRefGoogle Scholar
29. Peters, N. 1997 Kinetic foundation of thermal flame theory. Prog. Astronaut. Aeronaut. 173, 73–91.Google Scholar
30. Peters, N. & Williams, F. A. 1987 The asymptotic structure of stoichiometric methane air flames. Combust. Flame 68 (2), 185–207.CrossRefGoogle Scholar
31. Quinard, J. & Searby, G. 1990 Direct and indirect measurements of Markstein numbers of premixed flames. Combust. Flame 82 (3–4), 298–311.Google Scholar
32. Seshadri, K. & Peters, N. 1990 The inner structure of methane–air flames. Combust. Flame 81, 96–118.CrossRefGoogle Scholar
33. Sivashinsky, G. I. 1977 Nonlinear analysis of hydrodynamic instability in laminar flames-i. derivation of basic equations. Acta Astronaut. 4, 1177–1206.CrossRefGoogle Scholar
34. Zel’dovich, Ya. B. 1961 Chain reactions in hot flames – an approximate theory for flame velocity. Kinetika Katalis 2, 305–313.Google Scholar
35. Zel’dovich, Ya. B. & Frank-Kamenetskii, D. A. 1938 A theory of thermal flame propagation. Acta Physicochim. USSR IX, 341–350.Google Scholar