Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-27T11:46:20.988Z Has data issue: false hasContentIssue false
  • English
  • Français

Description of the transitional wake behind a strongly streamwise rotating sphere

Published online by Cambridge University Press:  01 June 2020

M. Lorite-Díez Open the ORCID record for M. Lorite-Díez [Opens in a new window]  and
J. I. Jiménez-González Open the ORCID record for J. I. Jiménez-González [Opens in a new window]
M. Lorite-Díez
Affiliation:
Departamento de Ingeniería Mecánica y Minera, Universidad de Jaén, Campus de las Lagunillas, 23071, Jaén, Spain
J. I. Jiménez-González*
Affiliation:
Departamento de Ingeniería Mecánica y Minera, Universidad de Jaén, Campus de las Lagunillas, 23071, Jaén, Spain
*
†Email address for correspondence: jignacio@ujaen.es
Get access Rights & Permissions [Opens in a new window]

Abstract

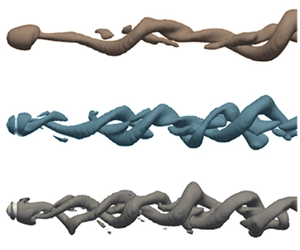
Direct numerical simulations are performed to study the flow regimes at the wake behind a strongly streamwise rotating sphere, covering the range of rotation parameters $0\leqslant \unicode[STIX]{x1D6FA}\leqslant 3$ and laminar and transitional Reynolds numbers $Re=250$, 500 and 1000. The wake dynamics is investigated in terms of flow topology, dominant modes and force coefficients. A higher wake complexity is found for growing values of the rotation parameter $\unicode[STIX]{x1D6FA}$ for all the Reynolds numbers investigated. In particular, at low and intermediate $Re$, successive bifurcations entail the development of periodic, quasi-periodic and irregular regimes, constituting a classical scenario of route to chaos, through the destabilization of different structures associated to incommensurate frequencies, which have been analysed by means of flow decomposition techniques. At low $Re$ and high rotation rates, the flow is governed by double-threaded structures due to the destabilization of helical symmetries of azimuthal wavenumber $m=2$, which are not dominant at larger $Re$. Interestingly, at intermediate values of $\unicode[STIX]{x1D6FA}$ and $Re=500$, a bistable dynamics is observed whereby the wake undergoes a random switching between a modulated quasi-periodic regime and an irregular regime, which is associated to a sudden increase of the drag coefficient, on account of the development of a double-celled recirculating bubble. Finally, for $Re=1000$, the flow is already chaotic at $\unicode[STIX]{x1D6FA}=0$, and the evolution with the rotation rate of the flow dynamics is simpler, with wake regimes being characterized by the rotation and massive shedding of vortex loops, that are a continuous deformation through axial rotation of the irregular wake behind the static sphere.

JFM classification

Wakes/Jets: Wakes
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 896 , 10 August 2020 , A18
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Auguste, F., Fabre, D. & Magnaudet, J. 2010 Bifurcations in the wake of a thick circular disk. Theor. Comput. Fluid Dyn. 24, 305–313.CrossRefGoogle Scholar
Barkla, H. M. & Auchterlone, L. J. 1971 The magnus or robins effect on rotating spheres. J. Fluid Mech. 47, 437–447.CrossRefGoogle Scholar
Cadot, O., Evrard, A. & Pastur, L. 2015 Imperfect supercritical bifurcation in a three-dimensional turbulent wake. Phys. Rev. E 91 (6), 063005.Google Scholar
Chrust, M., Goujon-Durand, S. & Wesfreid, J. E. 2013 Loss of a fixed plane symmetry in the wake of a sphere. J. Fluid Mech. 41, 51–56.CrossRefGoogle Scholar
Citro, V., Tchoufag, J., Fabre, D., Giannetti, F. & Luchini, P. 2016 Linear stability and weakly nonlinear analysis of the flow past rotating spheres. J. Fluid Mech. 807, 62–86.CrossRefGoogle Scholar
Clift, R., Grace, J. R. & Weber, M. E. 1978 Bubbles, Drops and Particles. Academic Press.Google Scholar
Dgheim, J., Abdallah, M., Habchi, R. & Zakhia, N. 2012 Heat and mass transfer investigation of rotating hydrocarbons droplet which behaves as a hard sphere. Appl. Math. Model. 36, 2646–2935.CrossRefGoogle Scholar
Fabre, D., Auguste, F. & Magnaudet, J. 2008 Bifurcations and symmetry breaking in the wake of axisymmetric bodies. Phys. Fluids 20, 051702.CrossRefGoogle Scholar
Geurts, B. J. 2004 Elements of Direct and Large-Eddy Simulation. R. T. Edwards, Inc.Google Scholar
Giacobello, M., Ooi, A. & Balachandar, S. 2009 Wake structure of a transversely rotating sphere at moderate Reynolds numbers. J. Fluid Mech. 621, 103–130.CrossRefGoogle Scholar
Gottwald, G. A. & Melbourne, I. 2004 Proceedings of the royal society of london. series a, mathematical and physical sciences. Proc. R. Soc. Lond. A 460 (2042), 603–611.CrossRefGoogle Scholar
Gottwald, G. A. & Melbourne, I. 2009 On the implementation of the 0–1 test for chaos. SIAM J. Appl. Dyn. Syst. 8 (1), 129–145.CrossRefGoogle Scholar
Issa, R. I. 1986 Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 62 (1), 40–65.CrossRefGoogle Scholar
Jiménez-González, J. I.2013 Study of the stability of jets and wakes. application to the wake past slender bodies with blunt trailing edge. PhD thesis, Universidad de Jaén.Google Scholar
Jiménez-González, J. I., Sanmiguel-Rojas, E., Sevilla, A. & Martínez-Bazán, C. 2013 Laminar flow past a spinning bullet-shaped body at moderate angular velocities. J. Fluid Mech. 43, 200–219.CrossRefGoogle Scholar
Jiménez-González, J. I., Sevilla, A., Sanmiguel-Rojas, E. & Martínez-Bazán, C. 2014 Global stability analysis of the axisymmetric wake past a spinning bullet-shaped body. Intl J. Fluid Mech. Res. 748, 302–327.CrossRefGoogle Scholar
Jiménez-González, J. I., Manglano-Villamarín, C. & Coenen, W. 2019 The role of geometry on the global instability of wakes behind streamwise rotating axisymmetric bodies. Eur. J. Mech. (B/Fluids) 76, 205–222.CrossRefGoogle Scholar
Johnson, T. A. & Patel, V. C. 1999 Flow past a sphere up to Reynolds number of 300. J. Fluid Mech. 378, 19–70.CrossRefGoogle Scholar
Kim, D. & Choi, H. 2002 Laminar flow past a sphere rotating in the streamwise direction. J. Fluid Mech. 461, 365–386.CrossRefGoogle Scholar
Klotz, L., Goujon-Durand, S., Rokicki, J. & Wesfreid, J. E. 2014 Experimental investigation of flow behind a cube for moderate Reynolds numbers. J. Fluid Mech. 750, 73–98.CrossRefGoogle Scholar
Kurien, S. & Taylor, M. 2005 Direct numerical simulation of turbulence: data generation and statistical analysis. Los Alamos Sci. 29, 142–151.Google Scholar
Kurose, R. & Komori, S. 1999 Drag and lift forces on rotating sphere in a linear shear flow. J. Fluid Mech. 384, 183–206.CrossRefGoogle Scholar
Lax, P. & Wendroff, B. 1960 Systems of conservation laws. Commun. Pure Appl. Maths 13 (2), 217–237.CrossRefGoogle Scholar
Le Clainche, S. & Vega, J. 2017a Higher order dynamic mode decomposition. SIAM J. Appl. Dyn. Syst. 16 (2), 882–925.CrossRefGoogle Scholar
Le Clainche, S. & Vega, J. M. 2017b Higher order dynamic mode decomposition to identify and extrapolate flow patterns. Phys. Fluids 29 (8), 084102.CrossRefGoogle Scholar
Liu, N. & Bogy, D. B. 2008 Forces on a rotating particle in a shear flow of a highly rarefied gas. Phys. Fluids 20, 107102.CrossRefGoogle Scholar
Neeraj, M. P. & Tiwari, S. 2018 Wake characteristics of a sphere performing streamwise rotary oscillations. Eur. J. Mech. (B/Fluids) 72, 485–500.CrossRefGoogle Scholar
Niazmand, H. & Renksizbulut, M. 2003 Surface effects on transient three-dimensional flows around rotating spheres at moderate Reynolds numbers. Comput. Fluids 32, 1405–1433.CrossRefGoogle Scholar
Niazmand, H. & Renksizbulut, M. 2005 Flow past a spinning sphere with surface blowing and heat transfer. J. Fluid Mech. 127 (1), 163–171.CrossRefGoogle Scholar
Peyer, K. E., Mahoney, A. W., Zhang, L., Abbott, J. J. & Nelson, B. J. 2012 Microbiorobotics: Biologically Inspired Microscale Robot Systems, Bacteria-Inspired Microrobots. Elsevier Inc.Google Scholar
Pier, B. 2013 Periodic and quasiperiodic vortex shedding in the wake of a rotating sphere. J. Fluids Struct. 41, 43–50.CrossRefGoogle Scholar
Poon, E. K. W., Ooi, A. S. H., Giacobello, M. & Cohen, R. C. Z. 2010 Laminar flow structures from a rotating sphere: effect of rotating axis angle. Intl J. Heat Fluid Flow 31 (5), 961–972.CrossRefGoogle Scholar
Poon, E. K. W., Ooi, A. S. H., Giacobello, M., Iaccarino, G. & Chung, D. 2014 Flow past a transversely rotating sphere at Reynolds numbers above the laminar regime. J. Fluid Mech. 759, 751–781.CrossRefGoogle Scholar
Roshko, A. 1993 Perspectives on bluff body aerodynamics. J. Wind Engng Ind. Aerodyn. 49 (1–3), 79–100.CrossRefGoogle Scholar
Ruith, M. R., Chen, P., Meiburg, E. & Maxworthy, T. 2003 Three-dimensional vortex breakdown in swirling jets and wakes: direct numerical simulation. J. Fluid Mech. 486, 331–378.CrossRefGoogle Scholar
Sakamoto, H. & Haniu, H. 1990 A study on vortex shedding from spheres in a uniform flow. Trans. ASME J. Fluids Engng 112 (4), 386–392.CrossRefGoogle Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.CrossRefGoogle Scholar
Skarysz, M., Rokicki, J., Goujon-Durand, S. & Wesfreid, J. E. 2018 Experimental investigation of the wake behind a rotating sphere. Phys. Rev. Fluids 3, 013905.Google Scholar
Spalart, P. R. & Squires, K. D. 2004 The Status of Detached-Eddy Simulation for Bluff Bodies, pp. 29–45. Springer.Google Scholar
Tomboulides, A. G. & Orszag, S. A. 2000 Numerical investigation of transitional and weak turbulent flow past a sphere. J. Fluid Mech. 416, 45–73.CrossRefGoogle Scholar
White, F. M. 2006 Fluid Mechanics, 6th edn. McGraw Hill College.Google Scholar
Wu, T. Y. T. 1972 Cavity and wake flows. Annu. Rev. Fluid Mech. 4 (1), 243–284.CrossRefGoogle Scholar

Lorite-Díez and Jiménez-González supplementary movie 1

Wake behind a streamwise rotating sphere (Re=250, Ω=0.4)

Download Lorite-Díez and Jiménez-González supplementary movie 1(Video)
Video 31.7 KB

Lorite-Díez and Jiménez-González supplementary movie 2

Wake behind a streamwise rotating sphere (Re=250, Ω=1.2)

Download Lorite-Díez and Jiménez-González supplementary movie 2(Video)
Video 230.3 KB

Lorite-Díez and Jiménez-González supplementary movie 3

Wake behind a streamwise rotating sphere (Re=250, Ω=1.6)

Download Lorite-Díez and Jiménez-González supplementary movie 3(Video)
Video 667.5 KB

Lorite-Díez and Jiménez-González supplementary movie 4

Wake behind a streamwise rotating sphere (Re=250, Ω=2.2)

Download Lorite-Díez and Jiménez-González supplementary movie 4(Video)
Video 602.5 KB

Lorite-Díez and Jiménez-González supplementary movie 5

Wake behind a streamwise rotating sphere (Re=250, Ω=3.0)

Download Lorite-Díez and Jiménez-González supplementary movie 5(Video)
Video 652.7 KB

Lorite-Díez and Jiménez-González supplementary movie 6

Wake behind a streamwise rotating sphere (Re=500, Ω=0.0)

Download Lorite-Díez and Jiménez-González supplementary movie 6(Video)
Video 173.6 KB

Lorite-Díez and Jiménez-González supplementary movie 7

Wake behind a streamwise rotating sphere (Re=500, Ω=0.4)

Download Lorite-Díez and Jiménez-González supplementary movie 7(Video)
Video 95.4 KB

Lorite-Díez and Jiménez-González supplementary movie 8

Wake behind a streamwise rotating sphere (Re=500, Ω=0.6)

Download Lorite-Díez and Jiménez-González supplementary movie 8(Video)
Video 357.9 KB

Lorite-Díez and Jiménez-González supplementary movie 9

Wake behind a streamwise rotating sphere (Re=500, Ω=1.1)

Download Lorite-Díez and Jiménez-González supplementary movie 9(Video)
Video 371.3 KB

Lorite-Díez and Jiménez-González supplementary movie 10

Wake behind a streamwise rotating sphere (Re=500, Ω=1.2)

Download Lorite-Díez and Jiménez-González supplementary movie 10(Video)
Video 412.1 KB

Lorite-Díez and Jiménez-González supplementary movie 11

Wake behind a streamwise rotating sphere (Re=500, Ω=3.0)

Download Lorite-Díez and Jiménez-González supplementary movie 11(Video)
Video 376.4 KB

Lorite-Díez and Jiménez-González supplementary movie 12

Wake behind a streamwise rotating sphere (Re=1000, Ω=1)

Download Lorite-Díez and Jiménez-González supplementary movie 12(Video)
Video 189.9 KB