Article contents
Diffusiophoresis of colloidal particles in neutral solute gradients at finite Péclet number
Published online by Cambridge University Press: 14 August 2013
Abstract
The role of neutral solute advection on the diffusiophoretic motion of colloidal particles is quantified. Theoretical analyses of this phenomenon usually assume that the solute concentration evolves solely via diffusion; that is, the Péclet number ( 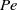 $\mathit{Pe}$ ) for solute transport is identically zero. This leads to the conclusion that the translational diffusiophoretic velocity of a colloid is independent of its size, shape, and orientation with respect to the imposed solute gradient, provided that the colloid has uniform surface properties and that the length scale of interaction between the solute and the particle surface is much smaller than the particle size (Morrison, J. Colloid Interface Sci. vol. 34, 1970, p. 210). For a single spherical colloid, we show that the particle velocity decreases monotonically with increasing
$\mathit{Pe}$ ) for solute transport is identically zero. This leads to the conclusion that the translational diffusiophoretic velocity of a colloid is independent of its size, shape, and orientation with respect to the imposed solute gradient, provided that the colloid has uniform surface properties and that the length scale of interaction between the solute and the particle surface is much smaller than the particle size (Morrison, J. Colloid Interface Sci. vol. 34, 1970, p. 210). For a single spherical colloid, we show that the particle velocity decreases monotonically with increasing 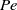 $\mathit{Pe}$ . Moreover, the solute concentration and fluid flow around the colloid become markedly fore–aft asymmetric as
$\mathit{Pe}$ . Moreover, the solute concentration and fluid flow around the colloid become markedly fore–aft asymmetric as  $\mathit{Pe}$ is increased. Next, an asymptotic expansion at small
$\mathit{Pe}$ is increased. Next, an asymptotic expansion at small 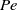 $\mathit{Pe}$ predicts that solute advection leads to relative phoretic motion between two identical spherical colloids, which ultimately align in a plane normal to the imposed gradient (there is no relative motion at
$\mathit{Pe}$ predicts that solute advection leads to relative phoretic motion between two identical spherical colloids, which ultimately align in a plane normal to the imposed gradient (there is no relative motion at 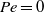 $\mathit{Pe}= 0$ ). Finally, asymptotic analysis of the diffusiophoretic motion of a slightly non-spherical colloid at small
$\mathit{Pe}= 0$ ). Finally, asymptotic analysis of the diffusiophoretic motion of a slightly non-spherical colloid at small 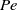 $\mathit{Pe}$ demonstrates that advection leads to a shape- and orientation-dependent particle velocity, in contrast to the insensitivity of the velocity to shape and orientation at
$\mathit{Pe}$ demonstrates that advection leads to a shape- and orientation-dependent particle velocity, in contrast to the insensitivity of the velocity to shape and orientation at  $\mathit{Pe}= 0$ .
$\mathit{Pe}= 0$ .
Information
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 36
- Cited by

