Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-14T06:52:11.623Z Has data issue: false hasContentIssue false
  • English
  • Français

A direct numerical simulation study of interface propagation in homogeneous turbulence

Published online by Cambridge University Press:  29 April 2015

R. Yu ,
X.-S. Bai  and
A. N. Lipatnikov
R. Yu
Affiliation:
Division of Fluid Mechanics, Lund University, Lund 221 00, Sweden
X.-S. Bai
Affiliation:
Division of Fluid Mechanics, Lund University, Lund 221 00, Sweden
A. N. Lipatnikov*
Affiliation:
Department of Applied Mechanics, Chalmers University of Technology, Göteborg 412 96, Sweden
*
†Email address for correspondence: lipatn@chalmers.se
Get access Rights & Permissions [Opens in a new window]

Abstract

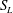A 3D direct numerical simulation (DNS) study of the evolution of a self-propagating interface in forced constant-density statistically stationary homogeneous isotropic turbulence was performed by solving Navier–Stokes and level-set equations under a wide range of conditions that cover various (from 0.1 to 2.0) ratios of the interface speed $S_{L}$ to the r.m.s. turbulent velocity  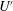$U^{\prime }$ and various (50, 100 and 200) turbulent Reynolds numbers $\mathit{Re}$. By analysing computed data, the following issues were addressed: (i) dependence of the speed and thickness of the fully developed statistically planar mean front that envelops the interface on $U^{\prime }/S_{L}$ and $\mathit{Re}$, (ii) dependence of the fully developed mean turbulent flux of a scalar $c$ that characterizes the state of the fluid ($c=0$ and 1 ahead and behind the interface respectively) on $U^{\prime }/S_{L}$ and $\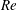mathit{Re}$, (iii) evolution of the mean front speed, its thickness, and the mean scalar flux during the front development after embedding a planar interface into the forced turbulence and (iv) relation between canonical and conditioned moments of the velocity, velocity gradient and pressure gradient fields.

JFM classification

Reacting Flows: Flames Turbulent Flows: Intermittency Reacting Flows: Turbulent reacting flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 772 , 10 June 2015 , pp. 127 - 164
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akkerman, V. & Bychkov, V. 2003 Turbulent flame and the Darrieus–Landau instability in a three-dimensional flow. Combust. Theor. Model. 7, 767–794.Google Scholar
Aldredge, R. C. 2006 The speed of iso-thermal front propagation in isotropic, weakly turbulent flows. Combust. Sci. Technol. 178, 1201–1215.Google Scholar
Aldredge, R. C. & Williams, F. A. 1991 Influence of wrinkled premixed-flame dynamics on large-scale, low-intensity turbulent flow. J. Fluid Mech. 228, 487–511.Google Scholar
Amato, A. & Lieuwen, T. C. 2014 Analysis of flamelet leading point dynamics in an inhomogeneous flow. Combust. Flame 161, 1337–1347.Google Scholar
Ashurst, W. T. & Sivashinsky, G. I. 1991 On flame propagation through periodic flow fields. Combust. Sci. Technol. 80, 159–164.Google Scholar
Aspden, A. J., Day, M. S. & Bell, J. B. 2015 Turbulence–chemistry interaction in lean premixed hydrogen combustion. Proc. Combust. Inst. 35, 1321–1329.Google Scholar
Batchelor, G. K. 1952 The effect of homogeneous turbulence on material lines and surfaces. Proc. R. Soc. Lond. A 213, 349–366.Google Scholar
Bilger, R. W., Pope, S. B., Bray, K. N. C. & Driscoll, J. F. 2005 Paradigms in turbulent combustion research. Proc. Combust. Inst. 30, 21–42.Google Scholar
Borghi, R. & Dutoya, D. 1978 On the scales of the fluctuations in turbulent combustion. Proc. Combust. Inst. 17, 235–244.CrossRefGoogle Scholar
Bray, K. N. C. 1995 Turbulent transport in flames. Proc. R. Soc. Lond. A 451, 231–256.Google Scholar
Bray, K. N. C., Libby, P. A. & Moss, J. B. 1985 Unified modeling approach for premixed turbulent combustion – part I: general formulation. Combust. Flame 61, 87–102.Google Scholar
Cambray, P. & Joulin, G. 1992 On moderately-forced premixed flames. Proc. Combust. Inst. 24, 61–67.Google Scholar
Carlsson, H., Yu, R. & Bai, X. S. 2014 Direct numerical simulation of lean premixed $\text{CH}_{4}$ /air and $\text{H}_{2}$ /air flames at high Karlovitz numbers. Intl J. Hydrog. Energy 39, 20216–20232.Google Scholar
Carlsson, H., Yu, R. & Bai, X. S. 2015 Flame structure analysis for categorization of lean premixed $\text{CH}_{4}$ /air and $\text{H}_{2}$ /air flames at high Karlovitz numbers: direct numerical simulation studies. Proc. Combust. Inst. 35, 1425–1432.CrossRefGoogle Scholar
Chaudhuri, S., Akkerman, V. & Law, C. K. 2011 Spectral formulation of turbulent flame speed with consideration of hydrodynamic instability. Phys. Rev. E 84, 026322.Google Scholar
Chen, J. H. 2011 Petascale direct numerical simulation of turbulent combustion – fundamental insights towards predictive models. Proc. Combust. Inst. 33, 99–123.CrossRefGoogle Scholar
Clavin, P. 1985 Dynamical behavior of premixed flame fronts in laminar and turbulent flows. Prog. Energy Combust. Sci. 11, 1–59.CrossRefGoogle Scholar
Clavin, P. & Williams, F. A. 1979 Theory of premixed-flame propagation in large-scale turbulence. J. Fluid Mech. 90, 589–604.Google Scholar
Corrsin, S. 1974 Limitations of gradient transport models in random walks and in turbulence. Adv. Geophys. 18A, 25–60.Google Scholar
Creta, F. & Matalon, M. 2011 Propagation of wrinkled turbulent flames in the context of hydrodynamic theory. J. Fluid Mech. 680, 225–264.Google Scholar
Damköhler, G. 1940 Der einfluss der turbulenz auf die flammengeschwindigkeit in gasgemischen. Z. Elektrochem. 46, 601–652.Google Scholar
Darrieus, G.1938 Propagation d’un front de flamme. Unpublished work, presented at La Technique Moderne (Paris), and in 1945 at Congrés de Mećanique Appliqueé (Paris).Google Scholar
Day, M., Tachibana, S., Bell, J., Lijewski, M., Beckner, V. & Cheng, R. K. 2012 A combined computational and experimental characterization of lean premixed low swirl laboratory flames I. Methane flames. Combust. Flame 159, 275–290.CrossRefGoogle Scholar
Dopazo, C. 1977 On conditioned averages for intermittent turbulent flows. J. Fluid Mech. 81, 433–438.Google Scholar
Eswaran, V. & Pope, S. B. 1988 An examination of forcing in direct numerical simulations of turbulence. Comput. Fluids 16, 257–278.Google Scholar
Fogla, N., Creta, F. & Matalon, M. 2013 Influence of the Darrieus–Landau instability on the propagation of planar turbulent flames. Proc. Combust. Inst. 34, 1509–1517.Google Scholar
Ghosal, S., Lund, T. S., Moin, P. & Akselvoll, K. 1995 A dynamic localization model for large-eddy simulation of turbulent flows. J. Fluid Mech. 286, 229–255.Google Scholar
Girimaji, S. S. & Pope, S. B. 1992 Propagating surfaces in isotropic turbulence. J. Fluid Mech. 234, 247–277.Google Scholar
Gottlieb, S. & Shu, C. W. 1998 Total variation diminishing Runge–Kutta schemes. Maths Comput. 67, 73–85.Google Scholar
Hemchandra, S. & Lieuwen, T. C. 2010 Local consumption speed of turbulent premixed flames – an analysis of memory effects. Combust. Flame 157, 955–965.Google Scholar
Im, Y. H., Huh, K. Y., Nishiki, S. & Hasegawa, T. 2004 Zone conditional assessment of flame-generated turbulence with DNS database of a turbulent premixed flame. Combust. Flame 137, 478–488.CrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Jiang, G. S. & Peng, D. 2000 Weighted ENO schemes for Hamilton–Jacobi equations. SIAM J. Sci. Comput. 21, 2126–2143.Google Scholar
Karlovitz, B., Denniston, D. W. & Wells, F. E. 1951 Investigation of turbulent flames. J. Chem. Phys. 19, 541–547.Google Scholar
Kerstein, A. R. & Ashurst, W. T. 1992 Propagation rate of growing interfaces in stirred fluids. Phys. Rev. Lett. 68, 934–937.Google Scholar
Kerstein, A. R. & Ashurst, W. T. 1994 Passage rates of propagating interfaces in randomly advected media and heterogeneous media. Phys. Rev. E 50, 1100–1113.Google Scholar
Kerstein, A. R., Ashurst, W. T. & Williams, F. A. 1988 Field equation for interface propagation in an unsteady homogeneous flow field. Phys. Rev. A 37, 2728–2731.Google Scholar
Klimov, A. M. 1975 Flame propagation in intense turbulence. Dokl. Akad. Nauk SSSR 221 (1), 56–59; (in Russian).Google Scholar
Kuznetsov, V. R. 1975 Certain peculiarities of movement of a flame front in a turbulent flow of homogeneous fuel mixtures. Combust. Explos. Shock Waves 11, 487–493.Google Scholar
Landau, L. D. 1944 On the theory of slow combustion. Acta Physicochim. USSR 19, 77–85.Google Scholar
Launder, B. E. 1976 Heat and mass transfer. In Turbulence (ed. Bradshaw, P.), Topics in Applied Physics, vol. 12, pp. 232–287. Springer.Google Scholar
Libby, P. A. 1975 On the prediction of intermittent turbulent flows. J. Fluid Mech. 68, 273–295.CrossRefGoogle Scholar
Libby, P. A. & Bray, K. N. C. 1981 Countergradient diffusion in premixed turbulent flames. AIAA J. 19, 205–213.Google Scholar
Lieuwen, T. C. 2012 Unsteady Combustor Physics. Cambridge University Press.CrossRefGoogle Scholar
Lipatnikov, A. 2012 Fundamentals of Premixed Turbulent Combustion. CRC Press.Google Scholar
Lipatnikov, A. N. 2008 Conditionally averaged balance equations for modeling premixed turbulent combustion in flamelet regime. Combust. Flame 152, 529–547.Google Scholar
Lipatnikov, A. N. 2009a Can we characterize turbulence in premixed flames? Combust. Flame 156, 1242–1247.Google Scholar
Lipatnikov, A. N. 2009b Testing premixed turbulent combustion models by studying flame dynamics. Intl J. Spray Combust. Dyn. 1, 39–66.Google Scholar
Lipatnikov, A. N. 2011 Conditioned moments in premixed turbulent reacting flows. Proc. Combust. Inst. 33, 1489–1496.CrossRefGoogle Scholar
Lipatnikov, A. N. & Chomiak, J. 1997 A simple model of unsteady turbulent flame propagation. SAE transactions. J. Engines 106 (3), 2441–2452.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2002 Turbulent flame speed and thickness: phenomenology, evaluation, and application in multi-dimensional simulations. Prog. Energy Combust. Sci. 28, 1–74.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2005 Molecular transport effects on turbulent flame propagation and structure. Prog. Energy Combust. Sci. 31, 1–73.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2007 Global stretch effects in premixed turbulent combustion. Proc. Combust. Inst. 31, 1361–1368.Google Scholar
Lipatnikov, A. N. & Chomiak, J. 2010 Effects of premixed flames on turbulence and turbulent scalar transport. Prog. Energy Combust. Sci. 36, 1–102.CrossRefGoogle Scholar
Lipatnikov, A. N., Chomiak, J., Sabelnikov, V. A., Nishiki, S. & Hasegawa, T. 2015 Unburned mixture fingers in premixed turbulent flames. Proc. Combust. Inst. 35, 1401–1408.Google Scholar
Mayo, J. R. & Kerstein, A. R. 2007 Scaling of Huygens-front speedup in weakly random media. Phys. Lett. A 372, 5–11.Google Scholar
Mayo, J. R. & Kerstein, A. R. 2008 Fronts in randomly advected and heterogeneous media and nonuniversality of Burgers turbulence: theory and numerics. Phys. Rev. E 78, 056307.CrossRefGoogle ScholarPubMed
Monin, A. S. 1965 On the symmetry of turbulence in the surface layer of air. Atmos. Ocean. Phys. 1 (1), 45–54.Google Scholar
Moss, J. B. 1980 Simultaneous measurements of concentration and velocity in an open premixed turbulent flame. Combust. Sci. Technol. 22, 119–129.Google Scholar
Peters, N. 2000 Turbulent Combustion. Cambridge University Press.Google Scholar
Philips, O. M. 1972 The entrainment interface. J. Fluid Mech. 51, 97–118.Google Scholar
Pocheau, A. 1994 Scale invariance in turbulent front propagation. Phys. Rev. E 49, 1109–1122.Google Scholar
Poinsot, T. & Veynante, D. 2005 Theoretical and Numerical Combustion, 2nd edn. Edwards.Google Scholar
Poludnenko, A. Y. 2015 Pulsating instability and self-acceleration of fast turbulent flames. Phys. Fluids 27, 014106.Google Scholar
Poludnenko, A. Y. & Oran, E. S. 2011 The interaction of high-speed turbulence with flames: turbulent flame speed. Combust. Flame 158, 301–326.Google Scholar
Prudnikov, A. G. 1967 Burning of homogeneous fuel–air mixtures in a turbulent flow. In Physical Principles of the Working Process in Combustion Chambers of Jet Engines (ed. Raushenbakh, B. V.), pp. 244–336. Clearing House for Federal Scientific & Technical Information.Google Scholar
Robin, V., Mura, A. & Champion, M. 2011 Direct and indirect thermal expansion effects in turbulent premixed flames. J. Fluid Mech. 689, 149–182.CrossRefGoogle Scholar
Russo, G. & Smereka, P. 2000 A remark on computing distance functions. J. Comput. Phys. 163, 51–67.CrossRefGoogle Scholar
Scurlock, A. C. & Grover, J. H. 1953 Propagation of turbulent flames. Proc. Combust. Inst. 4, 645–658.CrossRefGoogle Scholar
Shchelkin, K. I.1947 On combustion in a turbulent flow. NACA TM, 1110.Google Scholar
Shin, D.-H. & Lieuwen, T. 2013 Flame wrinkle destruction processes in harmonically forced, turbulent premixed flames. J. Fluid Mech. 721, 484–513.Google Scholar
Siggia, E. D. 1981 Numerical study of small-scale intermittency in three-dimensional turbulence. J. Fluid Mech. 107, 375–406.Google Scholar
Sun, M. B., Wang, Z. G. & Bai, X. S. 2010 Assessment and modification of sub-cell-fix method for re-initialization of level-set distance function. Intl J. Numer. Meth. Fluids 62, 211–236.Google Scholar
Sussman, M., Smereka, P. & Osher, S. 1994 A level set approach for computing solutions to incompressible two-phase flow. J. Comput. Phys. 114, 146–159.CrossRefGoogle Scholar
Swaminathan, N. & Grout, R. W. 2006 Interaction of turbulence and scalar fields in premixed flames. Phys. Fluids 18, 045102.Google Scholar
Taylor, G. I. 1935 Statistical theory of turbulence. IV. Diffusion in a turbulent air stream. Proc. R. Soc. Lond. A 151, 421–478.Google Scholar
Treurniet, T. C., Nieuwstadt, F. T. M. & Boersma, B. J. 2006 Direct numerical simulation of homogeneous turbulence in combination with premixed combustion at low Mach number modelled by the $G$ -equation. J. Fluid Mech. 565, 25–62.Google Scholar
Troiani, G., Battista, F. & Picano, F. 2013 Turbulent consumption speed via local dilatation rate measurements in a premixed Bunsen jet. Combust. Flame 160, 2029–2037.Google Scholar
Troiani, G., Creta, F. & Matalon, M. 2015 Experimental investigation of Darrieus–Landau instability effects on turbulent premixed flames. Proc. Combust. Inst. 35, 1451–1459.CrossRefGoogle Scholar
Veynante, D. & Vervisch, L. 2002 Turbulent combustion modeling. Prog. Energy Combust. Sci. 28, 193–266.Google Scholar
Wang, P.2005 Large eddy simulation of turbulent swirling flows and premixed turbulent combustion. PhD thesis, Lund University.Google Scholar
Wenzel, H. & Peters, N. 2000 Direct numerical simulation and modeling of kinematic restoration, dissipation and gas expansion effects of premixed flames in homogeneous turbulence. Combust. Sci. Technol. 158, 273–297.Google Scholar
Wenzel, H. & Peters, N. 2005 Scaling of production, kinematic restoration, and dissipation of the mean flame surface area. Combust. Sci. Technol. 177, 1095–1107.Google Scholar
Williams, F. A. 1985 Combustion Theory, 2nd edn. Benjamin/Cummings.Google Scholar
Yakhot, V. 1988 Propagation velocity of premixed turbulent flames. Combust. Sci. Technol. 60, 191–214.Google Scholar
Yanagi, T. & Mimura, Y. 1981 Velocity–temperature correlation in premixed flame. Proc. Combust. Inst. 18, 1031–1039.CrossRefGoogle Scholar
Yenerdag, B., Fukushima, N., Shimura, M., Tanahashi, M. & Miyauchi, T. 2015 Turbulence–flame interaction and fractal characteristics of $\text{H}_{2}$ –air premixed flame under pressure rising condition. Proc. Combust. Inst. 35, 1277–1285.Google Scholar
Yeung, P. K., Girimaji, S. S. & Pope, S. B. 1990 Straining and scalar dissipation of material surfaces in turbulence: implications for flamelets. Combust. Flame 79, 340–365.Google Scholar
Yu, J., Yu, R., Fan, X. Q., Christensen, M., Konnov, A. A. & Bai, X. S. 2013 Onset of cellular flame instability in adiabatic $\text{CH}_{4}/\text{O}_{2}/\text{CO}_{2}$ and $\text{CH}_{4}$ /air laminar premixed flames stabilized on a flat-flame burner. Combust. Flame 160, 1276–1286.CrossRefGoogle Scholar
Yu, R. & Bai, X. S. 2013a Direct numerical simulation of lean hydrogen/air auto-ignition in a constant volume enclosure. Combust. Flame 160, 1706–1716.Google Scholar
Yu, R. & Bai, X. S. 2013b A semi-implicit scheme for large eddy simulation of piston engine flow and combustion. Intl J. Numer. Meth. Fluids 71, 13–40.Google Scholar
Yu, R. & Bai, X. S. 2014 A fully divergence-free method for generation of inhomogeneous and anisotropic turbulence with large spatial variation. J. Comput. Phys. 256, 234–253.Google Scholar
Yu, R., Lipatnikov, A. N. & Bai, X. S. 2014 Three-dimensional direct numerical simulation study of conditioned moments associated with front propagation in turbulent flows. Phys. Fluids 26, 085104.Google Scholar
Yu, R., Yu, J. & Bai, X. S. 2012 An improved high-order scheme for DNS of low Mach number turbulent reacting flows based on stiff chemistry solver. J. Comput. Phys. 231, 5504–5521.Google Scholar
Zhang, F., Yu, R. & Bai, X. S. 2012 Detailed numerical simulation of syngas combustion under partially premixed combustion engine conditions. Intl J. Hydrog. Energy 37, 17285–17293.Google Scholar
Zimont, V. L. 1979 Theory of turbulent combustion of a homogeneous fuel mixture at high Reynolds number. Combust. Explos. Shock Waves 15, 305–311.Google Scholar
Zimont, V. L. 2000 Gas premixed combustion at high turbulence. Turbulent flame closure combustion model. Exp. Therm. Fluid Sci. 21, 179–186.Google Scholar
Zimont, V. L. & Pagnini, G. 2011 Lagrangian properties of turbulent diffusion with passive chemical reaction in the framework of the premixed combustion theory. Phys. Fluids 23, 035101.Google Scholar