Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhu, Bihai
Ji, Zengqi
Lou, Zhengkun
and
Qian, Pengcheng
2018.
Torque scaling in small-gap Taylor-Couette flow with smooth or grooved wall.
Physical Review E,
Vol. 97,
Issue. 3,
Ezeta, Rodrigo
Huisman, Sander G.
Sun, Chao
and
Lohse, Detlef
2018.
Turbulence strength in ultimate Taylor–Couette turbulence.
Journal of Fluid Mechanics,
Vol. 836,
Issue. ,
p.
397.
Zhu, Xiaojue
Verschoof, Ruben A.
Bakhuis, Dennis
Huisman, Sander G.
Verzicco, Roberto
Sun, Chao
and
Lohse, Detlef
2018.
Wall roughness induces asymptotic ultimate turbulence.
Nature Physics,
Vol. 14,
Issue. 4,
p.
417.
Jiang, Hechuan
Zhu, Xiaojue
Mathai, Varghese
Verzicco, Roberto
Lohse, Detlef
and
Sun, Chao
2018.
Controlling Heat Transport and Flow Structures in Thermal Turbulence Using Ratchet Surfaces.
Physical Review Letters,
Vol. 120,
Issue. 4,
Zhu, Xiaojue
Phillips, Everett
Spandan, Vamsi
Donners, John
Ruetsch, Gregory
Romero, Joshua
Ostilla-Mónico, Rodolfo
Yang, Yantao
Lohse, Detlef
Verzicco, Roberto
Fatica, Massimiliano
and
Stevens, Richard J.A.M.
2018.
AFiD-GPU: A versatile Navier–Stokes solver for wall-bounded turbulent flows on GPU clusters.
Computer Physics Communications,
Vol. 229,
Issue. ,
p.
199.
Verschoof, Ruben A.
Zhu, Xiaojue
Bakhuis, Dennis
Huisman, Sander G.
Verzicco, Roberto
Sun, Chao
and
Lohse, Detlef
2018.
Rough-wall turbulent Taylor-Couette flow: The effect of the rib height.
The European Physical Journal E,
Vol. 41,
Issue. 10,
Berghout, Pieter
Zhu, Xiaojue
Chung, Daniel
Verzicco, Roberto
Stevens, Richard J. A. M.
and
Lohse, Detlef
2019.
Direct numerical simulations of Taylor–Couette turbulence: the effects of sand grain roughness.
Journal of Fluid Mechanics,
Vol. 873,
Issue. ,
p.
260.
Singh, Harminder
Bonnesoeur, Antoine
Besnard, Hugues
Houssin, Claude
Prigent, Arnaud
Crumeyrolle, Olivier
and
Mutabazi, Innocent
2019.
A large thermal turbulent Taylor-Couette (THETACO) facility for investigation of turbulence induced by simultaneous action of rotation and radial temperature gradient.
Review of Scientific Instruments,
Vol. 90,
Issue. 11,
Bullee, Pim A.
Bakhuis, Dennis
Ezeta, Rodrigo
Huisman, Sander G.
Sun, Chao
Lammertink, Rob G.H.
and
Lohse, Detlef
2020.
Effect of axially varying sandpaper roughness on bubbly drag reduction in Taylor–Couette turbulence.
International Journal of Multiphase Flow,
Vol. 132,
Issue. ,
p.
103434.
Berghout, Pieter
Bullee, Pim A.
Fuchs, Thomas
Scharnowski, Sven
Kähler, Christian J.
Chung, Daniel
Lohse, Detlef
and
Huisman, Sander G.
2021.
Characterizing the turbulent drag properties of rough surfaces with a Taylor–Couette set-up.
Journal of Fluid Mechanics,
Vol. 919,
Issue. ,
Xu, Fan
Zhao, Peng
Sun, Chao
He, Yurong
and
Wang, Junwu
2022.
Direct numerical simulation of Taylor-Couette flow: Regime-dependent role of axial walls.
Chemical Engineering Science,
Vol. 263,
Issue. ,
p.
118075.
Xu, Fan
Su, Jinghong
Lan, Bin
Zhao, Peng
He, Yurong
Sun, Chao
and
Wang, Junwu
2023.
Direct numerical simulation of Taylor–Couette flow with vertical asymmetric rough walls.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Zhu, Xiaojue
Chen, Yibo
Chong, Kai Leong
Lohse, Detlef
and
Verzicco, Roberto
2024.
A boundary condition-enhanced direct-forcing immersed boundary method for simulations of three-dimensional phoretic particles in incompressible flows.
Journal of Computational Physics,
Vol. 509,
Issue. ,
p.
113028.
Fu, Yifeng
Bader, Shujaut H.
Song, Jiaxing
and
Zhu, Xiaojue
2024.
Turbulent spherical Rayleigh–Bénard convection: radius ratio dependence.
Journal of Fluid Mechanics,
Vol. 1000,
Issue. ,
Suga, K.
Takeda, K.
Amano, Y.
Kuwata, Y.
and
Kaneda, M.
2024.
Torque and heat transfer characteristics in Taylor–Couette turbulence with an axially grooved cylinder.
International Journal of Heat and Fluid Flow,
Vol. 110,
Issue. ,
p.
109586.
Tang, Kun
Yin, Bin
Yang, Yizhu
Cao, Xiyuan
Zhao, Liang
Fu, Wei
and
Dong, Hui
2025.
Nusselt number predictive model for Taylor–Couette flow with circumferential grooves under different operating parameters.
International Journal of Thermal Sciences,
Vol. 215,
Issue. ,
p.
110009.
 $Ta=10^{10}$. The dimensionless torque
$Ta=10^{10}$. The dimensionless torque 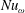 $Nu_{\unicode[STIX]{x1D714}}$ shows an effective scaling of
$Nu_{\unicode[STIX]{x1D714}}$ shows an effective scaling of  $Nu_{\unicode[STIX]{x1D714}}\propto Ta^{0.42\pm 0.01}$, which is steeper than the ultimate regime effective scaling
$Nu_{\unicode[STIX]{x1D714}}\propto Ta^{0.42\pm 0.01}$, which is steeper than the ultimate regime effective scaling  $Nu_{\unicode[STIX]{x1D714}}\propto Ta^{0.38}$ seen for smooth inner and outer walls. It is found that at the inner rough wall, the dominant contribution to the torque comes from the pressure forces on the radial faces of the rough elements; while viscous shear stresses on the rough surfaces contribute little to
$Nu_{\unicode[STIX]{x1D714}}\propto Ta^{0.38}$ seen for smooth inner and outer walls. It is found that at the inner rough wall, the dominant contribution to the torque comes from the pressure forces on the radial faces of the rough elements; while viscous shear stresses on the rough surfaces contribute little to 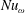 $Nu_{\unicode[STIX]{x1D714}}$. Thus, the log layer close to the rough wall depends on the roughness length scale, rather than on the viscous length scale. We then separate the torque contributed from the smooth inner wall and the rough outer wall. It is found that the smooth wall torque scaling follows
$Nu_{\unicode[STIX]{x1D714}}$. Thus, the log layer close to the rough wall depends on the roughness length scale, rather than on the viscous length scale. We then separate the torque contributed from the smooth inner wall and the rough outer wall. It is found that the smooth wall torque scaling follows 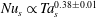 $Nu_{s}\propto Ta_{s}^{0.38\pm 0.01}$, in excellent agreement with the case where both walls are smooth. In contrast, the rough wall torque scaling follows
$Nu_{s}\propto Ta_{s}^{0.38\pm 0.01}$, in excellent agreement with the case where both walls are smooth. In contrast, the rough wall torque scaling follows  $Nu_{r}\propto Ta_{r}^{0.47\pm 0.03}$, very close to the pure ultimate regime scaling
$Nu_{r}\propto Ta_{r}^{0.47\pm 0.03}$, very close to the pure ultimate regime scaling 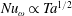 $Nu_{\unicode[STIX]{x1D714}}\propto Ta^{1/2}$. The energy dissipation rate at the wall of an inner rough cylinder decreases significantly as a consequence of the wall shear stress reduction caused by the flow separation at the rough elements. On the other hand, the latter shed vortices in the bulk that are transported towards the outer cylinder and dissipated. Compared to the purely smooth case, the inner wall roughness renders the system more bulk dominated and thus increases the effective scaling exponent.
$Nu_{\unicode[STIX]{x1D714}}\propto Ta^{1/2}$. The energy dissipation rate at the wall of an inner rough cylinder decreases significantly as a consequence of the wall shear stress reduction caused by the flow separation at the rough elements. On the other hand, the latter shed vortices in the bulk that are transported towards the outer cylinder and dissipated. Compared to the purely smooth case, the inner wall roughness renders the system more bulk dominated and thus increases the effective scaling exponent.