Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-28T00:11:42.280Z Has data issue: false hasContentIssue false
  • English
  • Français

Dissimilar turbulent heat transfer enhancement by Kelvin–Helmholtz rollers over high-aspect-ratio longitudinal ribs

Published online by Cambridge University Press:  24 November 2022

Y. Kuwata Open the ORCID record for Y. Kuwata [Opens in a new window]
Y. Kuwata*
Affiliation:
Department of Mechanical Engineering, Osaka Metropolitan University, Sakai, Osaka 599-8531, Japan
*
†Email address for correspondence: kuwata@me.osakafu-u.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

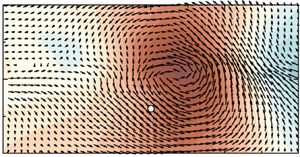
Passive heat transfer enhancement by spanwise rollers associated with the Kelvin–Helmholtz instability was studied through direct numerical simulations of high-aspect-ratio longitudinal ribs at the friction Reynolds number $300$. The temperature was treated as a passive scalar with Prandtl number unity to discuss the similarity between the heat and momentum transfer. The results reveal that the high-aspect-ratio longitudinal ribs lead to a favourable breakdown of the Reynolds analogy, that is, the enhancement of the heat transfer rate surpasses that of the frictional resistance. The favourable breakdown of the Reynolds analogy can be attributed to the enhanced turbulent heat flux compared with the Reynolds shear stress, whereas the rib-induced secondary flow plays a role in reducing the favourable breakdown of the Reynolds analogy. The conditional average statistics reveal that the high-pressure region accompanied by the spanwise rollers suppresses the spanwise roller-induced sweep and ejection motions, leading to smaller Reynolds shear stress than for the turbulent heat flux.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent convection Flow Control: Mixing enhancement
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 952 , 10 December 2022 , A21
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alben, S. 2017 Improved convection cooling in steady channel flows. Phys. Rev. Fluids 2 (10), 104501.CrossRefGoogle Scholar
Bons, J.P. 2002 St and $c_f$ augmentation for real turbine roughness with elevated freestream turbulence. In ASME Turbo Expo 2002: Power for Land, Sea, and Air, pp. 349–363. American Society of Mechanical Engineers.CrossRefGoogle Scholar
Brethouwer, G. 2021 Much faster heat/mass than momentum transport in rotating Couette flows. J.Fluid Mech. 912, A31.CrossRefGoogle Scholar
Breugem, W.P., Boersma, B.J. & Uittenbogaard, R.E. 2006 The influence of wall permeability on turbulent channel flow. J.Fluid Mech. 562, 35–72.CrossRefGoogle Scholar
Dipprey, D.F. & Sabersky, R.H. 1963 Heat and momentum transfer in smooth and rough tubes at various Prandtl numbers. Intl J. Heat Mass Transfer 6 (5), 329–353.CrossRefGoogle Scholar
Endrikat, S., Modesti, D., García-Mayoral, R., Hutchins, N. & Chung, D. 2021 Influence of riblet shapes on the occurrence of Kelvin–Helmholtz rollers. J.Fluid Mech. 913, A37.CrossRefGoogle Scholar
Forooghi, P., Stripf, M. & Frohnapfel, B. 2018 A systematic study of turbulent heat transfer over rough walls. Intl J. Heat Mass Transfer 127, 1157–1168.CrossRefGoogle Scholar
Garcia-Mayoral, R. & Jiménez, J. 2012 Scaling of turbulent structures in riblet channels up to $Re_{\tau } \simeq 550$. Phys. Fluids 24 (10), 105101.CrossRefGoogle Scholar
Hasegawa, Y. & Kasagi, N. 2011 Dissimilar control of momentum and heat transfer in a fully developed turbulent channel flow. J.Fluid Mech. 683, 57–93.CrossRefGoogle Scholar
Hinze, J.O. 1973 Experimental investigation on secondary currents in the turbulent flow through a straight conduit. Appl. Sci. Res. 28 (1), 453–465.CrossRefGoogle Scholar
Hwang, S., Dong, K., Hyun, G. & Cho, H.H. 2008 Heat transfer with dimple/protrusion arrays in a rectangular duct with a low Reynolds number range. Intl J. Heat Fluid Flow 29 (4), 916–926.CrossRefGoogle Scholar
Jin, Y. & Herwig, H. 2014 Turbulent flow and heat transfer in channels with shark skin surfaces: entropy generation and its physical significance. Intl J. Heat Mass Transfer 70, 10–22.CrossRefGoogle Scholar
Kaithakkal, A.J., Kametani, Y. & Hasegawa, Y. 2020 Dissimilarity between turbulent heat and momentum transfer induced by a streamwise travelling wave of wall blowing and suction. J.Fluid Mech. 886, A29.CrossRefGoogle Scholar
Kasagi, N., Hasegawa, Y., Fukagata, K. & Iwamoto, K. 2010 Control of turbulent transport: less friction and more heat transfer. In International Heat Transfer Conference, vol. 49439, pp. 309–324.Google Scholar
Katoh, K., Choi, K.-S. & Azuma, T. 2000 Heat-transfer enhancement and pressure loss by surface roughness in turbulent channel flows. Intl J. Heat Mass Transfer 43 (21), 4009–4017.CrossRefGoogle Scholar
Kays, W.M. & Crawford, M.E. 1993 Convective Heat and Mass Transfer, 3rd edn. McGraw-Hill.Google Scholar
Kuwata, Y. 2021 Direct numerical simulation of turbulent heat transfer on the Reynolds analogy over irregular rough surfaces. Intl J. Heat Fluid Flow 92, 108859.CrossRefGoogle Scholar
Kuwata, Y. 2022 Role of spanwise rollers by Kelvin–Helmholtz instability in turbulence over a permeable porous wall. Phys. Rev. Fluids 7 (8), 084606.CrossRefGoogle Scholar
Kuwata, Y. & Kawaguchi, Y. 2019 Direct numerical simulation of turbulence over systematically varied irregular rough surfaces. J.Fluid Mech. 862, 781–815.CrossRefGoogle Scholar
Kuwata, Y. & Nagura, R. 2020 Direct numerical simulation on the effects of surface slope and skewness on rough-wall turbulence. Phys. Fluids 32 (10), 105113.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2016 a Lattice Boltzmann direct numerical simulation of interface turbulence over porous and rough walls. Intl J. Heat Fluid Flow 61, 145–157.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2016 b Transport mechanism of interface turbulence over porous and rough walls. Flow Turbul. Combust. 97 (4), 1071–1093.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2017 Direct numerical simulation of turbulence over anisotropic porous media. J.Fluid Mech. 831, 41–71.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2019 Extensive investigation of the influence of wall permeability on turbulence. Intl J. Heat Fluid Flow 80, 108465.CrossRefGoogle Scholar
Kuwata, Y., Tsuda, K. & Suga, K. 2020 Direct numerical simulation of turbulent conjugate heat transfer in a porous-walled duct flow. J.Fluid Mech. 904, A9.CrossRefGoogle Scholar
Liou, T.-M., Hwang, J.-J. & Chen, S.-H. 1993 Simulation and measurement of enhanced turbulent heat transfer in a channel with periodic ribs on one principal wall. Intl J. Heat Mass Transfer 36 (2), 507–517.CrossRefGoogle Scholar
MacDonald, M., Hutchins, N. & Chung, D. 2019 Roughness effects in turbulent forced convection. J.Fluid Mech. 861, 138–162.CrossRefGoogle Scholar
Mahmood, G.I, Sabbagh, M.Z. & Ligrani, P.M. 2001 Heat transfer in a channel with dimples and protrusions on opposite walls. J.Thermophys. Heat Transfer 15 (3), 275–283.CrossRefGoogle Scholar
Motoki, S., Kawahara, G. & Shimizu, M. 2018 Optimal heat transfer enhancement in plane Couette flow. J.Fluid Mech. 835, 1157–1198.CrossRefGoogle Scholar
Murata, A. & Mochizuki, S. 2001 Comparison between laminar and turbulent heat transfer in a stationary square duct with transverse or angled rib turbulators. Intl J. Heat Mass Transfer 44 (6), 1127–1141.CrossRefGoogle Scholar
Nagano, Y., Hattori, H. & Houra, T. 2004 DNS of velocity and thermal fields in turbulent channel flow with transverse-rib roughness. Intl J. Heat Fluid Flow 25 (3), 393–403.CrossRefGoogle Scholar
Nakayama, A., Kuwahara, F. & Kodama, Y. 2006 An equation for thermal dispersion flux transport and its mathematical modelling for heat and fluid flow in a porous medium. J.Fluid Mech. 563, 81–96.CrossRefGoogle Scholar
Nishiyama, Y., Kuwata, Y. & Suga, K. 2020 Direct numerical simulation of turbulent heat transfer over fully resolved anisotropic porous structures. Intl J. Heat Fluid Flow 81, 108515.CrossRefGoogle Scholar
Peeters, J.W.R. & Sandham, N.D. 2019 Turbulent heat transfer in channels with irregular roughness. Intl J. Heat Mass Transfer 138, 454–467.CrossRefGoogle Scholar
Pirozzoli, S., Bernardini, M., Verzicco, R. & Orlandi, P. 2017 Mixed convection in turbulent channels with unstable stratification. J.Fluid Mech. 821, 482–516.CrossRefGoogle Scholar
Pokrajac, D., Finnigan, J.J., Manes, C., McEwan, I. & Nikora, V. 2006 On the definition of the shear velocity in rough bed open channel flows. In Proceedings of the International Conference on Fluvial Hydraulics, 6–8 September 2006, Lisbon, Portugal (ed. R.M.L. Ferreira, E.C.T.L. Alves, J.G.A.B. Leal & A.H. Cardoso), vol. 1, pp. 89–98.Google Scholar
Stalio, E. & Nobile, E. 2003 Direct numerical simulation of heat transfer over riblets. Intl J. Heat Fluid Flow 24 (3), 356–371.CrossRefGoogle Scholar
Stroh, A., Schäfer, K., Forooghi, P. & Frohnapfel, B. 2020 Secondary flow and heat transfer in turbulent flow over streamwise ridges. Intl J. Heat Fluid Flow 81, 108518.CrossRefGoogle Scholar
Suga, K., Chikasue, R. & Kuwata, Y. 2017 Modelling turbulent and dispersion heat fluxes in turbulent porous medium flows using the resolved LES data. Intl J. Heat Fluid Flow 68, 225–236.CrossRefGoogle Scholar
Suga, K., Matsumura, Y., Ashitaka, Y., Tominaga, S. & Kaneda, M. 2010 Effects of wall permeability on turbulence. Intl J. Heat Fluid Flow 31, 974–984.CrossRefGoogle Scholar
Suga, K., Okazaki, Y., HO, U. & Kuwata, Y. 2018 Anisotropic wall permeability effects on turbulent channel flows. J.Fluid Mech. 855, 983–1016.CrossRefGoogle Scholar
Yamamoto, A., Hasegawa, Y. & Kasagi, N. 2013 Optimal control of dissimilar heat and momentum transfer in a fully developed turbulent channel flow. J.Fluid Mech. 733, 189–220.CrossRefGoogle Scholar