Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T07:42:07.000Z Has data issue: false hasContentIssue false
  • English
  • Français

The dynamical states of a prolate spheroidal particle suspended in shear flow as a consequence of particle and fluid inertia

Published online by Cambridge University Press:  17 April 2015

T. Rosén ,
M. Do-Quang ,
C. K. Aidun  and
F. Lundell
T. Rosén
Affiliation:
Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden Wallenberg Wood Science Center, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
M. Do-Quang
Affiliation:
Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
C. K. Aidun
Affiliation:
George W. Woodruff School of Mechanical Engineering, and Parker H. Petit Institute for Bioengineering and Bioscience, 801 Ferst Drive, Georgia Institute of Technology, Atlanta, GA 30332-0405, USA
F. Lundell*
Affiliation:
Linné FLOW Centre, KTH Mechanics, Royal Institute of Technology, SE-100 44 Stockholm, Sweden Wallenberg Wood Science Center, Royal Institute of Technology, SE-100 44 Stockholm, Sweden
*
†Email address for correspondence: fredrik@mech.kth.se
Get access Rights & Permissions [Opens in a new window]

Abstract

The rotational motion of a prolate spheroidal particle suspended in shear flow is studied by a lattice Boltzmann method with external boundary forcing (LB-EBF). It has previously been shown that the case of a single neutrally buoyant particle is a surprisingly rich dynamical system that exhibits several bifurcations between rotational states due to inertial effects. It was observed that the rotational states were associated with either fluid inertia effects or particle inertia effects, which are always in competition. The effects of fluid inertia are characterized by the particle Reynolds number $\mathit{Re}_{p}=4Ga^{2}/{\it\nu}$, where $G$ is the shear rate, $a$ is the length of the particle major semi-axis and ${\it\nu}$ is the kinematic viscosity. Particle inertia is associated with the Stokes number $\mathit{St}={\it\alpha}\,\mathit{Re}_{p}$, where ${\it\alpha}$ is the solid-to-fluid density ratio. Previously, the neutrally buoyant case ($\mathit{St}=\mathit{Re}_{p}$) was studied extensively. However, little is known about how these results are affected when $\mathit{St}\neq \mathit{Re}_{p}$, and how the aspect ratio $r_{p}$ (major axis/minor axis) influences the competition between fluid and particle inertia in the absence of gravity. This work gives a full description of how prolate spheroidal particles in the range $2\leqslant r_{p}\leqslant 6$ behave depending on the chosen 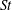$\mathit{St}$ and $\mathit{Re}_{p}$. Furthermore, consequences for the rheology of a dilute suspension containing such particles are discussed. Finally, grid resolution close to the particle is shown to affect the quantitative results considerably. It is suggested that this resolution is a major cause of quantitative discrepancies between different studies. Thus, the results of this work and previous direct numerical simulations of this problem should be regarded as qualitative descriptions of the physics involved, and more refined methods must be used to quantitatively pinpoint the transitions between rotational states.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Complex Fluids: Complex Fluids Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 771 , 25 May 2015 , pp. 115 - 158
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aidun, C. K., Lu, Y. & Ding, E.-J. 1998 Direct analysis of particulate suspensions with inertia using the discrete Boltzmann equation. J. Fluid Mech. 373, 287–311.CrossRefGoogle Scholar
Balkovsky, E., Falkovich, G. & Fouxon, A. 2001 Intermittent distribution of inertial particles in turbulent flows. Phys. Rev. Lett. 86, 2790–2793.CrossRefGoogle ScholarPubMed
Bayod, E. & Willers, E. P. 2002 Rheological and structural characterization of tomato paste and its influence on the quality of ketchup. LWT-Food Sci. Technol. 41, 1289–1300.CrossRefGoogle Scholar
Binder, R. C. 1939 The motion of cylindrical particles in viscous flow. J. Appl. Phys. 10, 711–713.Google Scholar
Crowe, C. T., Schwarzkopf, J. D., Sommerfeld, M. & Tsuji, Y. 2012 Multiphase Flows with Droplets and Particles, 2nd edn. CRC Press.Google Scholar
Ding, E.-J. & Aidun, C. K. 2000 The dynamics and scaling law for particles suspended in shear flow with inertia. J. Fluid Mech. 423, 317–344.CrossRefGoogle Scholar
Do-Quang, M., Amberg, G., Brethouwer, G. & Johansson, A. V. 2014 Simulation of finite-size fibers in turbulent channel flows. Phys. Rev. E 89, 013006.Google Scholar
Einstein, A. 1906 Eine neue Bestimmung der Moleküldimensionen. Ann. Phys. 324, 289–306.CrossRefGoogle Scholar
Einstein, A. 1911 Berichtigung zu meiner Arbeit: ‘Eine neue Bestimmung der Moleküldimensionen’. Ann. Phys. 339, 591–592.Google Scholar
Gavze, E., Pinsky, M. & Khain, A. 2012 The orientations of prolate ellipsoids in linear shear flows. J. Fluid Mech. 690, 51–93.Google Scholar
Hale, J. & Koçak, H. 1991 Dynamics and Bifurcations, Texts in Applied Mathematics, vol. 3. Springer.CrossRefGoogle Scholar
Hinch, E. J. & Leal, L. G. 1979 Rotation of small non-axisymmetric particles in a simple shear flow. J. Fluid Mech. 92, 591–607.CrossRefGoogle Scholar
Huang, H., Wu, Y.-F. & Lu, X.-Y. 2012a Shear viscosity of dilute suspensions of ellipsoidal particles with a lattice Boltzmann method. Phys. Rev. E 86, 046305.Google ScholarPubMed
Huang, H., Yang, X., Krafczyk, M. & Lu, X.-Y. 2012b Rotation of spheroidal particles in Couette flows. J. Fluid Mech. 692, 369–394.CrossRefGoogle Scholar
Jeffery, G. B. 1922 The motion of ellipsoidal particles immersed in a viscous fluid. Proc. R. Soc. Lond. A 102, 161–179.Google Scholar
Karnis, A., Goldsmith, H. L. & Mason, S. G. 1963 Axial migration of particles in Poiseuille flow. Nature 200, 159–160.Google Scholar
Latt, J.2007 Hydrodynamic limit of lattice Boltzmann equations. PhD thesis, University of Geneva.Google Scholar
Le, T. H., Dumont, P. J. J., Orgéas, L., Favier, D., Salvo, L. & Boller, E. 2008 X-ray phase contrast microtomography for the analysis of the fibrous microstructure of SMC composites. Composites A 39, 91–103.CrossRefGoogle Scholar
Li, Z., Zhu, J. & Zhang, C. 2005 Numerical simulations of ultrafine powder coating systems. Powder Technol. 150, 155–167.CrossRefGoogle Scholar
Lundell, F. 2011 The effect of particle inertia on triaxial ellipsoids in creeping shear: from drift toward chaos to a single periodic solution. Phys. Fluids 23, 011704.Google Scholar
Lundell, F. & Carlsson, A. 2010 Heavy ellipsoids in creeping shear flow: transitions of the particle rotation rate and orbit shape. Phys. Rev. E 81, 016323.Google ScholarPubMed
Lundell, F., Söderberg, L. D. & Alfredsson, P. H. 2011 Fluid mechanics of papermaking. Annu. Rev. Fluid Mech. 43, 195–217.Google Scholar
Miserocchi, G., Sancini, G., Mantegazza, F. & Chiappino, G. 2008 Translocation pathways for inhaled asbestos fibres. Environ. Health 7, 4.CrossRefGoogle Scholar
Mueller, S., Llewellin, E. W. & Mader, H. M. 2009 The rheology of suspensions of solid particles. Proc. R. Soc. Lond. A 466, 1201–1228.Google Scholar
Nilsen, C. & Andersson, H. I. 2013 Chaotic motion of inertial spheroids in oscillating shear flow. Phys. Fluids 25, 013303.Google Scholar
Pésceli, H. L., Trulsen, J. & Fiksen, Ø. 2012 Predator–prey encounter and capture rates for plankton in turbulent environments. Prog. Oceanogr. 101, 14–32.Google Scholar
Peskin, C. S. 2002 The immersed boundary method. Acta Numerica 11, 479–517.Google Scholar
Qi, D. & Luo, L.-S. 2002 Transitions in rotations of a nonspherical particle in a three-dimensional moderate Reynolds number Couette flow. Phys. Fluids 14, 4440.CrossRefGoogle Scholar
Qi, D. & Luo, L.-S. 2003 Rotational and orientational behaviour of three-dimensional spheroidal particles in Couette flows. J. Fluid Mech. 477, 201–213.CrossRefGoogle Scholar
Rosén, T., Lundell, F. & Aidun, C. K. 2014 Effect of fluid inertia on the dynamics and scaling of neutrally buoyant particles in shear flow. J. Fluid Mech. 738, 563–590.Google Scholar
Saffman, P. G. 1956 On the motion of small spheroidal particles in a viscous liquid. J. Fluid Mech. 1, 540–553.CrossRefGoogle Scholar
Strogatz, S. H. 1994 Nonlinear Dynamics and Chaos: with Applications to Physics, Biology, Chemistry, and Engineering. Westview.Google Scholar
Subramanian, G. & Koch, D. L. 2005 Inertial effects on fibre motion in simple shear flow. J. Fluid Mech. 535, 383–414.CrossRefGoogle Scholar
Subramanian, G. & Koch, D. L. 2006 Inertial effects on the orientation of nearly spherical particles in simple shear flow. J. Fluid Mech. 557, 257–296.CrossRefGoogle Scholar
Taylor, G. I. 1923 The motion of ellipsoidal particles in a viscous fluid. Proc. R. Soc. Lond. A 103, 58–61.Google Scholar
Wu, J. & Aidun, C. K. 2010 Simulating 3d deformable particle suspensions using lattice Boltzmann method with discrete external boundary force. Intl J. Numer. Meth. Fluids 62, 765–783.Google Scholar
Yarin, A. L., Gottlieb, O. & Roisman, I. V. 1997 Chaotic rotation of triaxial ellipsoids in simple shear flow. J. Fluid Mech. 340, 83–100.Google Scholar
Yu, Z., Phan-Thien, N. & Tanner, R. I. 2007 Rotation of a spheroid in a couette flow at moderate Reynolds numbers. Phys. Rev. E 76, 026310.Google Scholar
Zettner, C. M. & Yoda, M. 2001 Moderate aspect ratio elliptical cylinders in simple shear with inertia. J. Fluid Mech. 442, 241–266.Google Scholar

Rosén et al. supplementary movie

The movie illustrates the stable rotational states in each of the thirteen different regions in a Re_p/St-plane and the corresponding dynamical transitions and bifurcations between the regions.

Download Rosén et al. supplementary movie(Video)
Video 102.2 MB

Rosén et al. supplementary movie

The movie illustrates the seven different rotational states of a single prolate spheroidal particle in a linear shear flow.

Download Rosén et al. supplementary movie(Video)
Video 78.9 MB