Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-30T22:56:34.188Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of a hydrofoil free to oscillate in the wake of a fixed, constantly rotating or periodically rotating cylinder

Published online by Cambridge University Press:  02 August 2021

Todd M. Currier Open the ORCID record for Todd M. Currier [Opens in a new window] ,
Adrian G. Carleton  and
Yahya Modarres-Sadeghi Open the ORCID record for Yahya Modarres-Sadeghi [Opens in a new window]
Todd M. Currier
Affiliation:
Department of Mechanical and Industrial Engineering, University of Massachusetts, Amherst, MA01003, USA
Adrian G. Carleton
Affiliation:
Department of Mechanical and Industrial Engineering, University of Massachusetts, Amherst, MA01003, USA
Yahya Modarres-Sadeghi*
Affiliation:
Department of Mechanical and Industrial Engineering, University of Massachusetts, Amherst, MA01003, USA
*
†Email address for correspondence: modarres@engin.umass.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

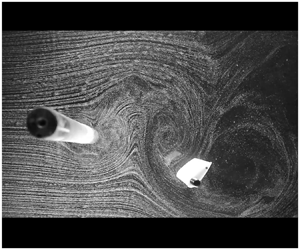
We present the dynamics of a hydrofoil free to oscillate in a plane as it interacts with vortices that are shed from a cylinder placed upstream. We consider cases where the cylinder is (i) fixed, (ii) forced to rotate constantly in one direction or (iii) forced to rotate periodically. When the upstream cylinder is fixed, at lower reduced velocities, the hydrofoil oscillates with a frequency equal to the frequency of vortices shed from the cylinder, and at higher reduced velocities with a frequency equal to half of the shedding frequency. When we force the cylinder to rotate in one direction, we control its wake and directly influence the response of the hydrofoil. When the rotation rate goes beyond a critical value, the vortex shedding in the cylinder's wake is suppressed and the hydrofoil is moved to one side and remains mainly static. When we force the cylinder to rotate periodically, we control the frequency of vortex shedding, which will be equal to the rotation frequency. Then at lower rotation frequencies, the hydrofoil interacts with one of the vortices in its oscillation path in the positive crossflow (transverse) direction, and with the second vortex in the negative crossflow direction, resulting in a 2:1 ratio between its inline and crossflow oscillations and a figure-eight trajectory. At higher rotation frequencies, the hydrofoil interacts with both shed vortices on its positive crossflow path and again in its negative crossflow path, resulting in a 1:1 ratio between its inline and crossflow oscillations and a linear trajectory.

JFM classification

Vortex Flows: Vortex shedding Wakes/Jets: Separated flows Wakes/Jets: Wakes
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 923 , 25 September 2021 , A21
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Assi, G.R.S., Bearman, P.W., Carmo, B.S., Meneghini, J.R., Sherwin, S.J. & Willden, R.H.J. 2013 The role of wake stiffness on the wake-induced vibration of the downstream cylinder of a tandem pair. J. Fluid Mech. 718, 210–245.CrossRefGoogle Scholar
Assi, G.R.S., Bearman, P.W. & Meneghini, J.R. 2010 On the wake-induced vibration of tandem circular cylinders: the vortex interaction excitation mechanism. J. Fluid Mech. 661, 365–401.CrossRefGoogle Scholar
Badr, H.M., Coutanceau, M., Dennis, S.C.R. & Menard, C. 1990 Unsteady flow past a rotating circular cylinder at Reynolds numbers 10 3 and 10 4. J. Fluid Mech. 220, 459–484.CrossRefGoogle Scholar
Bearman, P.W. 2011 Circular cylinder wakes and vortex-induced vibrations. J. Fluids Struct. 27 (5–6), 648–658.CrossRefGoogle Scholar
Chen, H. & Jaworski, J.W. 2020 Aeroelastic interactions and trajectory selection of vortex gusts impinging upon Joukowski airfoils. J. Fluids Struct. 96, 103026.CrossRefGoogle Scholar
Choi, H., Moin, P. & Kim, J. 1994 Active turbulence control for drag reduction in wall-bounded flows. J. Fluid Mech. 262, 75–110.CrossRefGoogle Scholar
Chou, M.-H. 1997 Synchronization of vortex shedding from a cylinder under rotary oscillation. Comput. Fluids 26 (8), 755–774.CrossRefGoogle Scholar
Currier, T. & Modarres-Sadeghi, Y. 2019 An experimental model with passively variable stiffness to investigate the effect of body stiffness on the fish fast-start maneuver. Exp. Fluids 60 (9), 147.CrossRefGoogle Scholar
Dahl, J.M., Hover, F.S. & Triantafyllou, M.S. 2006 Two-degree-of-freedom vortex-induced vibrations using a force assisted apparatus. J. Fluids Struct. 22 (6–7), 807–818.CrossRefGoogle Scholar
Dahl, J.M., Hover, F.S., Triantafyllou, M.S., Dong, S. & Karniadakis, G.E. 2007 Resonant vibrations of bluff bodies cause multivortex shedding and high frequency forces. Phys. Rev. Lett. 99 (14), 144503.CrossRefGoogle ScholarPubMed
Dahl, J.M., Hover, F.S., Triantafyllou, M.S. & Oakley, O.H. 2010 Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 643 (1), 395–424.CrossRefGoogle Scholar
Derakhshandeh, J.F., Arjomandi, M., Dally, B. & Cazzolato, B. 2016 Flow-induced vibration of an elastically mounted airfoil under the influence of the wake of a circular cylinder. Expl Therm. Fluid Sci. 74, 58–72.CrossRefGoogle Scholar
Diaz, F., Gavaldà, J., Kawall, J.G., Keffer, J.F. & Giralt, F. 1983 Vortex shedding from a spinning cylinder. Phys. Fluids 26 (12), 3454–3460.CrossRefGoogle Scholar
Du, L. & Dalton, C. 2013 Les calculation for uniform flow past a rotationally oscillating cylinder. J. Fluids Struct. 42, 40–54.CrossRefGoogle Scholar
Du, L., Jing, X. & Sun, X. 2014 Modes of vortex formation and transition to three-dimensionality in the wake of a freely vibrating cylinder. J. Fluids Struct. 49, 554–573.CrossRefGoogle Scholar
Fujisawa, N., Ikemoto, K. & Nagaya, K. 1998 Vortex shedding resonance from a rotationally oscillating cylinder. J. Fluids Struct. 12 (8), 1041–1053.CrossRefGoogle Scholar
Gad-el Hak, M. 2007 Flow Control: Passive, Active, and Reactive Flow Management. Cambridge University Press.Google Scholar
Gopalkrishnan, R., Triantafyllou, M.S., Triantafyllou, G.S. & Barrett, D. 1994 Active vorticity control in a shear flow using a flapping foil. J. Fluid Mech. 274, 1–21.CrossRefGoogle Scholar
Huera-Huarte, F.J. & Gharib, M. 2011 Vortex- and wake-induced vibrations of a tandem arrangement of two flexible circular cylinders with far wake interference. J. Fluids Struct. 27 (5–6), 824–828.CrossRefGoogle Scholar
Igarashi, T. 1997 Drag reduction of a square prism by flow control using a small rod. J. Wind Engng Ind. Aerodyn. 69, 141–153.CrossRefGoogle Scholar
Kumar, S., Cantu, C. & Gonzalez, B. 2011 Flow past a rotating cylinder at low and high rotation rates. J. Fluids Engng. 133 (4), 041201.CrossRefGoogle Scholar
Lachmann, G.V. 2014 Boundary Layer and Flow Control: Its Principles and Application. Elsevier.Google Scholar
Ma, P., Wang, Y., Xie, Y., Han, J., Sun, G. & Zhang, J. 2019 Effect of wake interaction on the response of two tandem oscillating hydrofoils. Energy Sci. Engng 7 (2), 431–442.CrossRefGoogle Scholar
Manela, A. 2013 Nonlinear effects of flow unsteadiness on the acoustic radiation of a heaving airfoil. J. Sound Vib. 332 (26), 7076–7088.CrossRefGoogle Scholar
Manela, A. & Huang, L. 2013 Point vortex model for prediction of sound generated by a wing with flap interacting with a passing vortex. J. Acoust. Soc. Am. 133 (4), 1934–1944.CrossRefGoogle ScholarPubMed
Mittal, S. & Kumar, B. 2003 Flow past a rotating cylinder. J. Fluid Mech. 476, 303–334.CrossRefGoogle Scholar
Mysa, R.C., Kaboudian, A. & Jaiman, R.K. 2016 On the origin of wake-induced vibration in two tandem circular cylinders at low Reynolds number. J. Fluids Struct. 61, 76–98.CrossRefGoogle Scholar
Rao, A., Radi, A., Leontini, J.S., Thompson, M.C., Sheridan, J. & Hourigan, K. 2015 A review of rotating cylinder wake transitions. J. Fluids Struct. 53, 2–14.CrossRefGoogle Scholar
Riso, C., Riccardi, G. & Mastroddi, F. 2016 Nonlinear aeroelastic modeling via conformal mapping and vortex method for a flat-plate airfoil in arbitrary motion. J. Fluids Struct. 62, 230–251.CrossRefGoogle Scholar
Rockwell, D. 1998 Vortex-body interactions. Annu. Rev. Fluid Mech. 30 (1), 199–229.CrossRefGoogle Scholar
Schouveiler, L., Hover, F.S. & Triantafyllou, M.S. 2005 Performance of flapping foil propulsion. J. Fluids Struct. 20 (7), 949–959.CrossRefGoogle Scholar
Seifert, A., Shtendel, T. & Dolgopyat, D. 2015 From lab to full scale active flow control drag reduction: how to bridge the gap? J. Wind Engng Ind. Aerodyn. 147, 262–272.CrossRefGoogle Scholar
Seifert, A., Stalnov, O., Sperber, D., Arwatz, G., Palei, V., David, S., Dayan, I. & Fono, I. 2009 Large trucks drag reduction using active flow control. In The Aerodynamics of Heavy Vehicles II: Trucks, Buses, and Trains (ed. F. Browand, R. McCallen & J. Ross), pp. 115–133. Springer.CrossRefGoogle Scholar
Seyed-Aghazadeh, B., Carlson, D.W. & Modarres-Sadeghi, Y. 2017 Vortex-induced vibration and galloping of prisms with triangular cross-sections. J. Fluid Mech. 817, 590–618.CrossRefGoogle Scholar
Seyed-Aghazadeh, B. & Modarres-Sadeghi, Y. 2015 An experimental investigation of vortex-induced vibration of a rotating circular cylinder in the crossflow direction. Phys. Fluids 27 (6), 067101.CrossRefGoogle Scholar
Shiels, D. & Leonard, A. 2001 Investigation of a drag reduction on a circular cylinder in rotary oscillation. J. Fluid Mech. 431, 297–322.CrossRefGoogle Scholar
Tokumaru, P.T. & Dimotakis, P.E. 1991 Rotary oscillation control of a cylinder wake. J. Fluid Mech. 224, 77–90.CrossRefGoogle Scholar
Tokumaru, P.T. & Dimotakis, P.E. 1993 The lift of a cylinder executing rotary motions in a uniform flow. J. Fluid Mech. 255, 1–10.CrossRefGoogle Scholar
Triantafyllou, M.S., Techet, A.H. & Hover, F.S. 2004 Review of experimental work in biomimetic foils. IEEE J. Ocean. Engng 29 (3), 585–594.CrossRefGoogle Scholar
Wang, L., Alam, M.M. & Zhou, Y. 2018 Two tandem cylinders of different diameters in cross-flow: effect of an upstream cylinder on wake dynamics. J. Fluid Mech. 836, 5–42.CrossRefGoogle Scholar
Zhu, Q. 2011 Optimal frequency for flow energy harvesting of a flapping foil. J. Fluid Mech. 675, 495–517.CrossRefGoogle Scholar

Currier et al. supplementary movie 1

The interactions of the hydrofoil with the vortices in the wake of the fixed cylinder in a sample case of Region I

Download Currier et al. supplementary movie 1(Video)
Video 734.1 KB

Currier et al. supplementary movie 2

The interactions of the hydrofoil with the vortices in the wake of the fixed cylinder in a sample case of Region III

Download Currier et al. supplementary movie 2(Video)
Video 1.1 MB

Currier et al. supplementary movie 3

See word file for movie caption

Download Currier et al. supplementary movie 3(Video)
Video 8.8 MB

Currier et al. supplementary movie 4

See word file for movie caption

Download Currier et al. supplementary movie 4(Video)
Video 11.1 MB
Supplementary material: File

Currier et al. supplementary material

Captions for movies 1-4

Download Currier et al. supplementary material(File)
File 72.3 KB