Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-14T17:37:28.285Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of a spherical body shedding from a hypersonic ramp. Part 1. Inviscid flow

Published online by Cambridge University Press:  16 November 2020

C. E. Sousa ,
R. Deiterding Open the ORCID record for R. Deiterding [Opens in a new window]  and
S. J. Laurence Open the ORCID record for S. J. Laurence [Opens in a new window]
C. E. Sousa
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
R. Deiterding
Affiliation:
School of Engineering, University of Southampton, Boldrewood Campus, SouthamptonSO16 7QF, UK
S. J. Laurence*
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
*
†Email address for correspondence: stuartl@umd.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

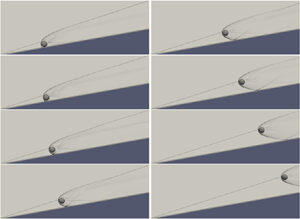
Numerical simulations are employed to investigate the dynamical separation of an initially stationary sphere from the surface of a two-dimensional ramp in hypersonic flow. We consider the inviscid limit, which is equivalent to assuming the sphere radius to be much larger than the ramp boundary-layer thickness; this assumption allows a range of Mach numbers and ramp angles to be explored efficiently. Of particular interest is determining how the shock-surfing phenomenon discovered by Laurence & Deiterding (J. Fluid Mech., vol. 676, 2011, pp. 396–431), in which a spherical body can stably oscillate about an oblique shock as it moves downstream, manifests itself in such a situation. First, the isolated interactions between a sphere and an oblique shock, and then between a sphere and an inviscid wall, are examined independently to elucidate relevant trends. Full trajectory predictions are subsequently performed using a decoupled model in which the shock and wall interactions are assumed to contribute independently to the aerodynamic forces. Three types of trajectories are found to be possible: surfing of the spherical body; initial expulsion outside the shock layer followed by re-entry and entrainment; or direct entrainment. At relatively low hypersonic Mach numbers, the latter two types of trajectories are predominant, but at higher Mach numbers ($M\gtrsim 10$), surfing becomes possible over an increasingly wide range of ramp angles and downstream release locations. By reparameterizing the release location as the initial lateral distance of the sphere from the shock, good collapse of the transition boundary delineating surfing from ejection/re-entrainment over various Mach numbers and ramp angles is obtained.

JFM classification

Aerodynamics: High-speed flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 906 , 10 January 2021 , A28
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Anderson, J. D. 2006 Hypersonic and High-Temperature Gas Dynamics, 2nd edn. AIAA Education Series.CrossRefGoogle Scholar
Artem'eva, N. A. & Shuvalov, V. V. 1996 Interaction of shock waves during the passage of a disrupted meteoroid through the atmosphere. Shock Waves 5, 359–367.CrossRefGoogle Scholar
Berger, M. & Colella, P. 1988 Local adaptive mesh refinement for shock hydrodynamics. J. Comput. Phys. 82, 64–84.CrossRefGoogle Scholar
Bertin, J. J. & Cummings, R. M. 2006 Critical hypersonic aerothermodynamic phenomena. Annu. Rev. Fluid Mech. 38, 129–157.CrossRefGoogle Scholar
Butler, C. S., Whalen, T. J., Sousa, C. E. & Laurence, S. J. 2021 Dynamics of a spherical body shedding from a hypersonic ramp. Part 2. Viscous flow. J. Fluid Mech. 906, A29.Google Scholar
Deiterding, R. 2003 Parallel adaptive simulation of multi-dimensional detonation structures. PhD thesis, Brandenburgische Technische Universität Cottbus.Google Scholar
Deiterding, R. 2005 Construction and application of an AMR algorithm for distributed memory computers. In Adaptive Mesh Refinement - Theory and Applications, Lecture Notes in Computational Science and Engineering, vol. 41, pp. 361–372. Springer.CrossRefGoogle Scholar
Deiterding, R. 2009 A parallel adaptive method for simulating shock-induced combustion with detailed chemical kinetics in complex domains. Comput. Struct. 87, 769–783.CrossRefGoogle Scholar
Deiterding, R. 2011 Block-structured adaptive mesh refinement - theory, implementation and application. ESAIM Proc. 34, 97–150.CrossRefGoogle Scholar
Edney, B. E. 1968 a Anomalous heat transfer and pressure distributions on blunt bodies at hypersonic speed in the presence of impinging shocks. FFA Rep. 115. The Aeronautical Research Institute of Sweden.CrossRefGoogle Scholar
Edney, B. E. 1968 b Effects of shock impingement on the heat transfer around blunt bodies. AIAA J. 6 (1), 15–21.CrossRefGoogle Scholar
Fedkiw, R. P., Aslam, T., Merriman, B. & Osher, S. 1999 A non-oscillatory Eulerian approach to interfaces in multimaterial flows (the ghost fluid method). J. Comput. Phys. 152, 457–492.CrossRefGoogle Scholar
Gnoffo, P. A., Weilmuenster, K. J., Braun, R. D. & Cruz, C. I. 1996 Influence of sonic-line location on Mars Pathfinder Probe aerothermodynamics. J. Spacecr. Rockets 33 (2), 169–177.CrossRefGoogle Scholar
Graham, W. J. & Davis, B. M. 1965 The change of Pitot pressure across oblique shock waves in a perfect gas. C.P. No. 783. Aeronautical Research Council.Google Scholar
Laurence, S. J. 2006 Proximal bodies in hypersonic flow. PhD thesis, California Institute of Technology.Google Scholar
Laurence, S. J. & Deiterding, R. 2011 Shock-wave surfing. J. Fluid Mech. 676, 396–431.CrossRefGoogle Scholar
Laurence, S. J., Deiterding, R. & Hornung, H. G. 2007 Proximal bodies in hypersonic flow. J. Fluid Mech. 590, 209–237.CrossRefGoogle Scholar
Laurence, S. J., Parziale, N. & Deiterding, R. 2012 Dynamical separation of spherical bodies in supersonic flow. J. Fluid Mech. 713, 159–182.CrossRefGoogle Scholar
Lees, L. 1955 Hypersonic flow. In Proceedings of 5th International Conference on Los Angeles, pp. 241–276. Institute of Aeronautical Society.Google Scholar
Park, S.-H. & Park, G. 2020 Separation process of multi-spheres in hypersonic flow. Adv. Space Res. 65 (1), 392–406.CrossRefGoogle Scholar
Register, P. J., Aftosmis, M. J., Stern, E. C., Brock, J. M., Seltner, P. M., Willems, S., Guelhan, A. & Mathias, D. L. 2020 Interactions between asteroid fragments during atmospheric entry. Icarus 337, 113468.CrossRefGoogle Scholar
Zeng, Y., Wang, D., Xiong, X., Zhang, X., Withers, P. J., Sun, W., Smith, M., Bai, M. & Xiao, P. 2017 Ablation-resistant carbide $Zr_{0.8}Ti_{0.2}{C}_{0.74}{B}_{0.26}$ for oxidizing environments up to 3,000$^{\circ }$C. Nat. Commun. 8, 15836.CrossRefGoogle ScholarPubMed
Ziegler, J. L., Deiterding, R., Shepherd, J. E. & Pullin, D. I. 2011 An adaptive high-order hybrid scheme for compressive, viscous flows with detailed chemistry. J. Comput. Phys. 230 (20), 7598–7630.CrossRefGoogle Scholar