Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-14T17:11:50.241Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of a spherical body shedding from a hypersonic ramp. Part 2. Viscous flow

Published online by Cambridge University Press:  16 November 2020

C. S. Butler ,
T. J. Whalen ,
C. E. Sousa  and
S. J. Laurence Open the ORCID record for S. J. Laurence [Opens in a new window]
C. S. Butler
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
T. J. Whalen
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
C. E. Sousa
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
S. J. Laurence*
Affiliation:
Department of Aerospace Engineering, University of Maryland, College Park, MD20742, USA
*
†Email address for correspondence: stuartl@umd.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

The separation dynamics of a sphere released from the surface of a ramp into a hypersonic flow is investigated, focusing on the influence of the ramp boundary layer on the sphere behaviour. First, numerical simulations are conducted of a sphere interacting with an isolated high-speed boundary layer to determine the influence on the sphere force coefficients as the sphere diameter and wall-normal location are varied. It is found that the lift coefficient is strongly affected by the near-wall interactions, becoming increasingly negative as the ratio of the sphere radius to boundary-layer thickness, $r/\delta$, is decreased. These results are combined with force coefficients derived from simulations of the sphere interacting with the ramp-generated oblique shock to enable numerical predictions of the sphere trajectories for a $10^{\circ }$ ramp at Mach 6 (using a similar decoupled approach to Part 1 of this work). It is found that the three trajectory types of the inviscid situation – shock surfing, ejection followed by re-entrainment within the shock layer and direct entrainment – also characterize the sphere behaviour here. Their relative prevalence, however, is influenced by the sphere size: for smaller values of $r/\delta$, direct entrainment dominates because of the wall suction, while shock surfing and then ejection/re-entrainment become increasingly likely at larger values of $r/\delta$. Increasing the ramp angle and/or the free-stream Mach number reduces the relative influence of the boundary-layer interactions. Finally, experiments are conducted using free-flying spheres released from a ramp surface in a hypersonic shock tunnel, confirming the major trends predicted numerically.

JFM classification

Aerodynamics: High-speed flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 906 , 10 January 2021 , A29
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bailey, A. B. & Hiatt, J. 1971 Free-flight measurements of sphere drag at subsonic, transonic, supersonic, and hypersonic speeds for continuum, transition, and near-free-molecular flow conditions. AEDC-TR-70-291.Google Scholar
Borker, R., Huang, D., Grimberg, S., Farhat, C., Avery, P. & Rabinovitch, J. 2019 Mesh adaptation framework for embedded boundary methods for computational fluid dynamics and fluid-structure interaction. Intl J. Numer. Meth. Fluids 90, 389–424.CrossRefGoogle Scholar
Britan, A., Elperin, T., Igra, O. & Jiang, P. 1995 Acceleration of a sphere behind planar shock waves. Exp. Fluids 20, 84–90.CrossRefGoogle Scholar
Butler, C. & Laurence, S. J. 2019 HyperTERP: a newly commissioned hypersonic shock tunnel at the University of Maryland. AIAA Paper 2019-2860.CrossRefGoogle Scholar
Candler, G. V. 2011 Numerical simulation of hypersonic shock wave-boundary-layer interactions. In Shock Wave-Boundary-Layer Interactions (ed. H. Babinsky & J. K. Harvey), pp. 314–335. Cambridge University Press.CrossRefGoogle Scholar
Farhat, C., Gerbeau, J. & Rallu, A. 2012 Fiver: a finite volume method based on exact two-phase Riemann problems and sparse grids for multi-material flows with large density jumps. J. Comput. Phys. 231, 6360–6379.CrossRefGoogle Scholar
Hung, F. T. & Clauss, J. M. 1981 Three-dimensional protuberance interference heating in high speed flow. Prog. Aeronaut. Astronaut. 77, 109–136.Google Scholar
Koren, B. 1993 A robust upwind discretization method for advection, diffusion and source terms. In Numerical Methods for Advection–Diffusion Problems (ed. C.B. Vreugdenhil & B. Koren), Notes on Numerical Fluid Mechanics, pp. 117–138. Vieweg.Google Scholar
Lakshmanan, B. & Tiwari, S. N. 1994 Investigation of three-dimensional separation at wing/body junctions in supersonic flows. AIAA J. 31, 64–71.Google Scholar
Laurence, S. J. 2012 On tracking the motion of rigid bodies through edge detection and least-squares fitting. Exp. Fluids 52, 387–401.CrossRefGoogle Scholar
Laurence, S. J. & Deiterding, R. 2011 Shock-wave surfing. J. Fluid Mech. 676, 396–431.CrossRefGoogle Scholar
Laurence, S. J., Deiterding, R. & Hornung, H. G. 2007 Proximal bodies in hypersonic flow. J. Fluid Mech. 590, 209–237.CrossRefGoogle Scholar
Laurence, S. J. & Karl, S. 2010 An improved visualization-based force-measurement technique for short-duration hypersonic facilities. Exp. Fluids 48, 949–965.CrossRefGoogle Scholar
Laurence, S. J., Parziale, N. & Deiterding, R. 2012 Dynamical separation of spherical bodies in supersonic flow. J. Fluid Mech. 713, 159–182.CrossRefGoogle Scholar
Ozawa, H. & Laurence, S. J. 2018 Experimental investigation of the shock-induced flow over a wall-mounted cylinder. J. Fluid Mech. 849, 1009–1042.CrossRefGoogle Scholar
Özkan, O. & Holt, M. 1984 Supersonic separated flow past a cylindrical obstacle on a flat plate. AIAA J. 22, 611–617.CrossRefGoogle Scholar
Sedney, R. & Kitchens, C. W. 1971 Survey of viscous interactions associated with high Mach number flight. AIAA J. 9, 771–784.Google Scholar
Sousa, C. E., Deiterding, R. & Laurence, S. J. 2021 Dynamics of a spherical body shedding from a hypersonic ramp. Part 1. Inviscid flow. J. Fluid Mech. 906, A28.Google Scholar
Sun, M., Saito, T., Takayama, K. & Tanno, H. 2005 Unsteady drag on a sphere by shock wave loading. Shock Waves 14, 3–9.CrossRefGoogle Scholar
Tanno, H., Itoh, K., Saito, T., Abe, A. & Takayama, K. 2003 Interaction of a shock with a sphere suspended in a vertical shock tube. Shock Waves 13, 191–200.CrossRefGoogle Scholar
Tutty, O. R., Roberts, G. T. & Schuricht, P. H. 2013 High-speed laminar flow past a fin-body junction. J. Fluid Mech. 737, 19–55.CrossRefGoogle Scholar
White, F. 1991 Viscous Fluid Flow, 2nd edn. McGraw-Hill.Google Scholar
White, J. A. & Morrison, J. H. 1999 A pseudo-temporal multi-grid relaxation scheme for solving the parabolized navier stokes equations. AIAA Paper 99-3360.CrossRefGoogle Scholar

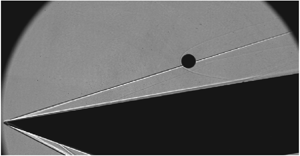
Butler et al. supplementary movie 1

Experimental shadowgraph movie of a 3.18-mm diameter sphere: x0/r=3.9, r/δ=5.6.

Download Butler et al. supplementary movie 1(Video)
Video 3 MB

Butler et al. supplementary movie 2

Experimental shadowgraph movie of a 3.18-mm diameter sphere: x0/r=7.2, r/δ=3.9.

Download Butler et al. supplementary movie 2(Video)
Video 2.7 MB

Butler et al. supplementary movie 3

Experimental shadowgraph movie of a 3.18-mm diameter sphere: x0/r=7.9, r/δ=3.7.

Download Butler et al. supplementary movie 3(Video)
Video 2.4 MB

Butler et al. supplementary movie 4

Experimental shadowgraph movie of a 3.18-mm diameter sphere: x0/r=9.1, r/δ=3.7.

Download Butler et al. supplementary movie 4(Video)
Video 2.1 MB