Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-10T06:50:16.013Z Has data issue: false hasContentIssue false
  • English
  • Français

Effect of surface contamination on interfacial mass transfer rate

Published online by Cambridge University Press:  29 September 2017

J. G. Wissink Open the ORCID record for J. G. Wissink [Opens in a new window] ,
H. Herlina ,
Y. Akar  and
M. Uhlmann Open the ORCID record for M. Uhlmann [Opens in a new window]
J. G. Wissink*
Affiliation:
Department of Mechanical, Aerospace and Civil Engineering, Brunel University London, Kingston Lane, Uxbridge UB8 3PH, UK
H. Herlina
Affiliation:
Institute for Hydromechanics, Karlsruhe Institute of Technology, Kaiserstr. 12, 76131 Karlsruhe, Germany
Y. Akar
Affiliation:
Department of Mechanical, Aerospace and Civil Engineering, Brunel University London, Kingston Lane, Uxbridge UB8 3PH, UK
M. Uhlmann
Affiliation:
Institute for Hydromechanics, Karlsruhe Institute of Technology, Kaiserstr. 12, 76131 Karlsruhe, Germany
*
†Email address for correspondence: jan.wissink@brunel.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

The influence of surface contamination upon the mass transfer rate of a low diffusivity gas across a flat surface is studied using direct numerical simulations. The interfacial mass transfer is driven by isotropic turbulence diffusing from below. Similar to Shen et al. (J. Fluid Mech., vol. 506, 2004, pp. 79–115) the surface contamination is modelled by relating the normal gradient of the horizontal velocities at the top to the horizontal gradients of the surfactant concentrations. A broad range of contamination levels is considered, including clean to severely contaminated conditions. The time-averaged results show a strong correlation between the gas transfer velocity and the clean surface fraction of the surface area. 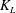In the presence of surface contamination the mass transfer velocity $K_{L}$ is found to scale as a power of the Schmidt number, i.e. 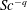$Sc^{-q}$, where 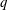$q$ smoothly transitions from $q=1/2$ for clean surfaces to 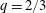$q=2/3$ for very dirty interfaces. A power law 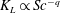$K_{L}\propto Sc^{-q}$ is proposed in which both the exponent 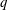$q$ and the constant of proportionality become functions of the clean surface fraction.

JFM classification

Geophysical and Geological Flows: Air/sea interactions Turbulent Flows: Turbulent convection Mixing: Turbulent mixing
Type
Papers
Information
Journal of Fluid Mechanics , Volume 830 , 10 November 2017 , pp. 5 - 34
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Asher, W. E. & Pankow, J. F. 1986 The interaction of mechanically generated turbulence and interfacial films with a liquid phase controlled gas/liquid transport process. Tellus 38B, 305–318.Google Scholar
Banerjee, S. & MacIntyre, S. 2004 The air–water interface: turbulence and scalar exchange. In Advances in Coastal and Ocean Engineering, pp. 181–237. World Scientific.Google Scholar
Banerjee, S., Scott, D. S. & Rhodes, E. 1968 Mass transfer to falling wavy liquid films in turbulent flow. Ind. Engng Chem. Fundam. 7 (1), 22–27.Google Scholar
Brumley, B. H. & Jirka, G. H. 1987 Near-surface turbulence in a grid-stirred tank. J. Fluid Mech. 183, 235–263.Google Scholar
Calmet, I. & Magnaudet, J. 2003 Statistical structure of high-Reynolds-number turbulence close to the free surface of an open-channel flow. J. Fluid Mech. 474, 355–378.Google Scholar
Coantic, M. 1986 A model of gas transfer across air–water interfaces with capillary waves. J. Geophys. Res. 91 (C3), 3925–3943.Google Scholar
Danckwerts, P. V. 1951 Significance of liquid-film coefficients in gas absorption. Ind. Engng Chem. 43 (6), 1460–1467.Google Scholar
Davies, J. T. 1972 Turbulence Phenomena. Academic.Google Scholar
Espedal, H. A., Johannessen, O. M. & Knulst, J. 1996 Satellite detection of natural films on the ocean surface. Geophys. Res. Lett. 23 (22), 3151–3154.Google Scholar
Fortescue, G. E. & Pearson, J. R. 1967 On gas absorption into a turbulent liquid. Chem. Engng Sci. 22 (9), 1163–1176.Google Scholar
Handler, R. A., Leighton, R. I., Smith, G. B. & Nagaosa, R. 2003 Surfactant effects on passive scalar transport in a fully developed turbulent flow. Intl J. Heat Mass Transfer 46 (12), 2219–2238.Google Scholar
Hasegawa, Y. & Kasagi, N. 2008 Systematic analysis of high Schmidt number turbulent mass transfer across clean, contaminated and solid interfaces. Intl J. Heat Fluid Flow 29 (3), 765–773.Google Scholar
Herlina, H. & Jirka, G. H. 2008 Experiments on gas transfer at the air–water interface induced by oscillating grid turbulence. J. Fluid Mech. 594, 183–208.CrossRefGoogle Scholar
Herlina, H. & Wissink, J. G. 2014 Direct numerical simulation of turbulent scalar transport across a flat surface. J. Fluid Mech. 744, 217–249.Google Scholar
Herlina, H. & Wissink, J. G. 2016 Isotropic-turbulence-induced mass transfer across severely contaminated water surface. J. Fluid Mech. 797, 665–682.Google Scholar
Hopfinger, E. J. & Toly, J.-A. 1976 Spatially decaying turbulence and its relation to mixing across density interfaces. J. Fluid Mech. 78 (1), 155–175.Google Scholar
Jähne, B. & Haussecker, H. 1998 Air–water gas exchange. Annu. Rev. Fluid Mech. 30, 443–468.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Kermani, A., Khakpour, H. R., Shen, L. & Igusa, T. 2011 Statistics of surface renewal of passive scalars in free-surface turbulence. J. Fluid Mech. 678, 379–416.CrossRefGoogle Scholar
Khakpour, H. R., Shen, L. & Yue, D. K. P. 2011 Transport of passive scalar in turbulent shear flow under a clean or surfactant-contaminated free surface. J. Fluid Mech. 670, 527–557.Google Scholar
Kubrak, B., Herlina, H., Greve, F. & Wissink, J. G. 2013 Low-diffusivity scalar transport using a WENO scheme and dual meshing. J. Comput. Phys. 240, 158–173.CrossRefGoogle Scholar
Lamont, J. C. & Scott, D. S. 1970 An eddy cell model of mass transfer into surface of a turbulent liquid. AIChE J. 16 (4), 513–519.CrossRefGoogle Scholar
Law, C. N. S. & Khoo, B. C. 2002 Transport across a turbulent air–water interface. AIChE J. 48 (9), 1856–1868.CrossRefGoogle Scholar
Ledwell, J. J. 1984 The variation of the gas transfer coefficient with molecular diffusity. In Gas Transfer at Water Surfaces, pp. 293–302. Springer.Google Scholar
Liu, X., Osher, S. & Chan, T. 1994 Weighted essentially non-oscillatory schemes. J. Comput. Phys. 115 (1), 200–212.Google Scholar
Magnaudet, J. & Calmet, I. 2006 Turbulent mass transfer through a flat shear-free surface. J. Fluid Mech. 553, 155–185.Google Scholar
McCready, M. J., Vassiliadou, E. & Hanratty, T. J. 1986 Computer-simulation of turbulent mass-transfer at a mobile interface. AIChE J. 32 (7), 1108–1115.Google Scholar
McKenna, S. P. & McGillis, W. R. 2004 The role of free-surface turbulence and surfactants in air–water gas transfer. Intl J. Heat Mass Transfer 47 (3), 539–553.Google Scholar
Na, Y. & Hanratty, T. J. 2000 Limiting behavior of turbulent scalar transport close to a wall. Intl J. Heat Mass Transfer 43 (10), 1749–1758.Google Scholar
Nagaosa, R. & Handler, R. A. 2003 Statistical analysis of coherent vortices near a free surface in a fully developed turbulence. Phys. Fluids 15 (2), 375–394.Google Scholar
Notter, R. H. & Sleicher, C. A. 1971 The eddy diffusivity in the turbulent boundary layer near a wall. Chem. Engng Sci. 26 (1), 161–171.Google Scholar
Peirson, W. L. & Banner, M. L. 2003 Aqueous surface layer flows induced by microscale breaking wind waves. J. Fluid Mech. 479, 1–38.Google Scholar
Perot, B. & Moin, P. 1995 Shear-free turbulent boundary layers. Part 1. Physical insights into near-wall turbulence. J. Fluid Mech. 295, 199–227.Google Scholar
Salter, M. E., Upstill-Goddard, R. C., Nightingale, P. D., Archer, S. D., Blomquist, B., Ho, D. T., Huebert, B., Schlosser, P. & Yang, M. 2011 Impact of an artificial surfactant release on air–sea gas fluxes during deep ocean gas exchange experiment II. J. Geophys. Res. 116, C11016.CrossRefGoogle Scholar
Shen, L., Yue, D. K. P. & Triantafyllou, G. S. 2004 Effect of surfactants on free-surface turbulent flows. J. Fluid Mech. 506, 79–115.Google Scholar
Theofanous, T. G., Houze, R. N. & Brumfield, L. K. 1976 Turbulent mass transfer at free, gas liquid interfaces with applications to open channel, bubble and jet flows. Intl J. Heat Mass Transfer 19, 613–624.Google Scholar
Tsai, W.-T. 1996 Impact of a surfactant on a turbulent shear layer under the air–sea interface. J. Geophys. Res. 101 (C12), 28557–28568.Google Scholar
Turney, D. E. 2016 Coherent motions and time scales that control heat and mass transfer at wind-swept water surfaces. J. Geophys. Res. 121 (12), 8731–8748.Google Scholar
Turney, D. E. & Banerjee, S. 2013 Air–water gas transfer and near-surface motions. J. Fluid Mech. 733, 588–624.Google Scholar
Walker, D. T., Leighton, R. I. & Garza-Rios, L. O. 1996 Shear-free turbulence near a flat free surface. J. Fluid Mech. 320, 19–51.CrossRefGoogle Scholar
Wissink, J. G. 2004 On unconditional conservation of kinetic energy by finite-difference discretisations of the linear and non-linear convection equation. Comput. Fluids 33, 315–343.Google Scholar
Wissink, J. G. & Herlina, H. 2016 Direct numerical simulation of gas transfer across the air–water interface driven by buoyant convection. J. Fluid Mech. 787, 508–540.Google Scholar