Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-14T23:15:11.888Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of rotation on the bulk turbulent convection

Published online by Cambridge University Press:  25 October 2019

Francesco Toselli Open the ORCID record for Francesco Toselli [Opens in a new window] ,
Stefano Musacchio  and
Guido Boffetta
Francesco Toselli*
Affiliation:
Dipartimento di Fisica and INFN, Università di Torino, via P. Giuria 1, 10125 Torino, Italy
Stefano Musacchio
Affiliation:
Dipartimento di Fisica and INFN, Università di Torino, via P. Giuria 1, 10125 Torino, Italy
Guido Boffetta
Affiliation:
Dipartimento di Fisica and INFN, Università di Torino, via P. Giuria 1, 10125 Torino, Italy
*
†Email address for correspondence: francesco.toselli@unito.it
Get access Rights & Permissions [Opens in a new window]

Abstract

We study rotating homogeneous turbulent convection forced by a mean vertical temperature gradient by means of direct numerical simulations in the Boussinesq approximation in a rotating frame. In the absence of rotation, our results are in agreement with the ‘ultimate regime of thermal convection’ for the scaling of the Nusselt and Reynolds numbers versus Rayleigh and Prandtl numbers. Rotation is found to increase both 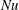$Nu$ and 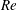$Re$ at fixed 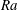$Ra$ with a maximum enhancement for intermediate values of the Rossby numbers, qualitatively similar, but with stronger intensity, to what is observed in Rayleigh–Bénard rotating convection. Our results are interpreted in terms of a quasi-bidimensionalization of the flow with the formation of columnar structures displaying strong correlation between the temperature and the vertical velocity fields.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Rotating turbulence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 881 , 25 December 2019 , pp. 648 - 659
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.Google Scholar
Baldwin, K. A., Scase, M. M. & Hill, R. J. 2015 The inhibition of the Rayleigh–Taylor instability by rotation. Sci. Rep. 5, 11706.Google Scholar
Biferale, L., Bonaccorso, F., Mazzitelli, I. M., van Hinsberg, M. A. T., Lanotte, A. S., Musacchio, S., Perlekar, P. & Toschi, F. 2016 Coherent structures and extreme events in rotating multiphase turbulent flows. Phys. Rev. X 6, 041036.Google Scholar
Bodenschatz, E., Pesch, W. & Ahlers, G. 2000 Recent developments in Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 32, 709–778.Google Scholar
Boffetta, G., De Lillo, F., Mazzino, A. & Vozella, L. 2012 The ultimate state of thermal convection in Rayleigh–Taylor turbulence. Physica D 241, 137–140.Google Scholar
Boffetta, G. & Mazzino, A. 2017 Incompressible Rayleigh–Taylor turbulence. Annu. Rev. Fluid Mech. 49, 119–143.Google Scholar
Boffetta, G., Mazzino, A. & Musacchio, S. 2011 Effects of polymer additives on Rayleigh–Taylor turbulence. Phys. Rev. E 83, 056318.Google Scholar
Boffetta, G., Mazzino, A. & Musacchio, S. 2016 Rotating Rayleigh–Taylor turbulence. Phys. Rev. Fluids 1, 054405.Google Scholar
Borue, V. & Orszag, S. A. 1997 Turbulent convection driven by a constant temperature gradient. J. Sci. Comput. 12, 305–351.Google Scholar
Brown, E., Nikolaenko, A., Funfschilling, D. & Ahlers, G. 2005 Heat transport in turbulent Rayleigh–Bénard convection: effect of finite top- and bottom-plate conductivities. Phys. Fluids 17, 075108.Google Scholar
Busse, F. H. 1994 Convection driven zonal flows and vortices in the major planets. Chaos 4, 123–134.Google Scholar
Calzavarini, E., Lohse, D., Toschi, F. & Tripiccione, R. 2005 Rayleigh and Prandtl number scaling in the bulk of Rayleigh–Bénard turbulence. Phys. Fluids 17, 055107.Google Scholar
Chandrasekhar, S. 1961 Hydromagnetic and Hydrodynamic Stability. Clarendon.Google Scholar
Chong, K. L., Yang, Y., Huang, S.-D., Zhong, J.-Q., Stevens, R. J. A. M., Verzicco, R., Lohse, D. & Xia, K.-Q. 2017 Confined Rayleigh–Bénard, rotating Rayleigh–Bénard, and double diffusive convection: a unifying view on turbulent transport enhancement through coherent structure manipulation. Phys. Rev. Lett. 119, 064501.Google Scholar
Gallet, B., Campagne, A., Cortet, P.-P. & Moisy, F. 2014 Scale-dependent cyclone-anticyclone asymmetry in a forced rotating turbulence experiment. Phys. Fluids 26, 035108.Google Scholar
Godeferd, F. S. & Moisy, F. 2015 Structure and dynamics of rotating turbulence: a review of recent experimental and numerical results. Appl. Mech. Rev. 67 (3), 030802.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16, 4462–4472.Google Scholar
Hartmann, D. L., Moy, L. A. & Fu, Q. 2001 Tropical convection and the energy balance at the top of the atmosphere. J. Clim. 14, 4495–4511.Google Scholar
Hopfinger, E. J., Browand, F. K. & Gagne, Y. 1982 Turbulence and waves in a rotating tank. J. Fluid Mech. 125, 505–534.Google Scholar
Johnston, J. P. 1998 Effects of system rotation on turbulence structure: a review relevant to turbomachinery flows. Intl J. Rotating Mach. 4, 97–112.Google Scholar
Julien, K., Legg, S., McWilliams, J. & Werne, J. 1996 Hard turbulence in rotating Rayleigh–Bénard convection. Phys. Rev. E 53, R5557.Google Scholar
King, E. M., Stellmach, S., Noir, J., Hansen, U. & Aurnou, J. M. 2009 Boundary layer control of rotating convection systems. Nature 457, 301–304.Google Scholar
Kraichnan, R. H. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5, 1374–1389.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2008 Breakdown of large-scale circulation in turbulent rotating convection. Eur. Phys. Lett. 84, 24001.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2010 Vortex statistics in turbulent rotating convection. Phys. Rev. E 82, 036306.Google Scholar
Kunnen, R. P. J., Stevens, R. J. A. M., Overkamp, J., Sun, C., van Heijst, G. F. & Clercx, H. J. H. 2011 The role of Stewartson and Ekman layers in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 422–442.Google Scholar
Lohse, D. & Toschi, F. 2003 Ultimate state of thermal convection. Phys. Rev. Lett. 90, 034502.Google Scholar
Marshall, J. & Schott, F. 1999 Open-ocean convection: observations, theory, and models. Rev. Geophys. 37, 1–64.Google Scholar
Miesch, M. S. 2000 The coupling of solar convection and rotation. In Helioseismic Diagnostics of Solar Convection and Activity, pp. 59–89. Springer.Google Scholar
Niemela, J. J., Babuin, S. & Sreenivasan, K. R. 2010 Turbulent rotating convection at high Rayleigh and Taylor numbers. J. Fluid Mech. 649, 509–522.Google Scholar
Rahmstorf, S. 2000 The thermohaline ocean circulation: a system with dangerous thresholds? Climatic Change 46 (3), 247–256.Google Scholar
Rossby, H. T. 1969 A study of Bénard convection with and without rotation. J. Fluid Mech. 36, 309–335.Google Scholar
Sprague, M., Julien, K., Knobloch, E. & Werne, J. 2006 Numerical simulation of an asymptotically reduced system for rotationally constrained convection. J. Fluid Mech. 551, 141–174.Google Scholar
Staplehurst, P. J., Davidson, P. A. & Dalziel, S. B. 2008 Structure formation in homogeneous freely decaying rotating turbulence. J. Fluid Mech. 598, 81–105.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2010a Optimal Prandtl number for heat transfer in rotating Rayleigh–Bénard convection. New J. Phys. 12, 075005.Google Scholar
Stevens, R. J. A. M., Clercx, H. J. H. & Lohse, D. 2013 Heat transport and flow structure in rotating Rayleigh–Bénard convection. Eur. J. Mech. (B/Fluids) 40, 41–49.Google Scholar
Stevens, R. J. A. M., Overkamp, J., Lohse, D. & Clercx, H. J. H. 2011 Effect of aspect ratio on vortex distribution and heat transfer in rotating Rayleigh–Bénard convection. Phys. Rev. E 84, 056313.Google Scholar
Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2010b Radial boundary layer structure and Nusselt number in Rayleigh–Bénard convection. J. Fluid Mech. 643, 495–507.Google Scholar
Stevens, R. J. A. M., Zhong, J.-Q., Clercx, H. J. H., Ahlers, G. & Lohse, D. 2009 Transitions between turbulent states in rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 103, 024503.Google Scholar
Yeung, P. K. & Zhou, Y. 1998 Numerical study of rotating turbulence with external forcing. Phys. Fluids 10, 2895–2909.Google Scholar
Yoshimatsu, K., Midorikawa, M. & Kaneda, Y. 2011 Columnar eddy formation in freely decaying homogeneous rotating turbulence. J. Fluid Mech. 677, 154–178.Google Scholar
Zhong, J.-Q., Stevens, R. J. A. M., Clercx, H. J. H., Verzicco, R., Lohse, D. & Ahlers, G. 2009 Prandtl-, Rayleigh-, and Rossby-number dependence of heat transport in turbulent rotating Rayleigh–Bénard convection. Phys. Rev. Lett. 102, 044502.Google Scholar