Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-29T12:41:25.141Z Has data issue: false hasContentIssue false
  • English
  • Français

Equilibrium shapes and floatability of static and vertically vibrated heavy liquid drops on the surface of a lighter fluid

Published online by Cambridge University Press:  19 July 2021

Andrey Pototsky Open the ORCID record for Andrey Pototsky [Opens in a new window] ,
Alexander Oron Open the ORCID record for Alexander Oron [Opens in a new window]  and
Michael Bestehorn Open the ORCID record for Michael Bestehorn [Opens in a new window]
Andrey Pototsky*
Affiliation:
Department of Mathematics, Swinburne University of Technology, Hawthorn, Victoria3122, Australia
Alexander Oron
Affiliation:
Department of Mechanical Engineering, Technion-Israel Institute of Technology, Haifa3200003, Israel
Michael Bestehorn
Affiliation:
Institute of Physics, Brandenburg University of Technology, 03013Cottbus-Senftenberg, Germany
*
†Email address for correspondence: apototskyy@swin.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

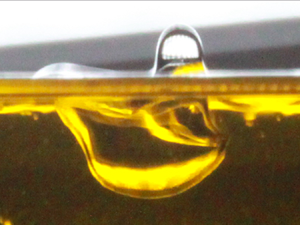
A small drop of a heavier fluid may float on the surface of a lighter fluid supported by surface tension forces. In equilibrium, the drop assumes a radially symmetric shape with a circular triple-phase contact line. We show that such a floating liquid drop with a sufficiently small volume has two distinct equilibrium shapes at terrestrial gravity: one with a larger and one with a smaller radius of the triple-phase contact line. Static stability analysis reveals that both shapes could be stable if the drop volume is below a certain critical value. Experiments conducted with $\mathrm {\mu }\textrm {L}$-sized water drops floating on commercial oil support the existence of multiple contact line radii for a drop with fixed volume. Next, we experimentally study the floatability of a less viscous water drop on the surface of a more viscous and less dense oil, subjected to a low-frequency (Hz-order) vertical vibration. We find that in a certain range of amplitudes, vibration helps heavy liquid drops to stay afloat. The physical mechanism of the increased floatability is explained by the horizontal elongation of the drop driven by subharmonic Faraday waves. The average length of the triple-phase contact line increases as the drop elongates that leads to a larger average lifting force produced by the surface tension.

JFM classification

Drops and Bubbles: Drops Waves/Free-surface Flows: Faraday waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 922 , 10 September 2021 , A31
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Apffel, B., Novkoski, F., Eddi, A. & Fort, E. 2015 Floating under a levitating liquid. Nature 585, 48–52.CrossRefGoogle Scholar
Benilov, E.S. 2016 Stability of a liquid bridge under vibration. Phys. Rev. E 93, 063118.CrossRefGoogle ScholarPubMed
Bestehorn, M. & Pototsky, A. 2016 Faraday instability and nonlinear pattern formation of a two-layer system: a reduced model. Phys. Rev. Fluids 1, 063905.CrossRefGoogle Scholar
Boucher, E.A., Evans, M.J.B. & Frank, F.C. 1975 Pendent drop profiles and related capillary phenomena. Proc. R. Soc. Lond. A 346 (1646), 349–374.Google Scholar
Bratukhin, Y.K. & Makarov, S.O. 1994 Interphase convection. Perm University Press.Google Scholar
Bratukhin, Y.K., Makarov, S.O. & Teplova, O.V. 2001 Equilibrium shapes and stability of floating drops. Fluid Dyn. 36, 529–537.CrossRefGoogle Scholar
Doedel, E.J., Fairgrieve, T.F., Sandstede, B., Champneys, A.R., Kuznetsov, Y.A. & Wang, X. 2007 Auto-07p: Continuation and bifurcation software for ordinary differential equations. Tech. Rep.Google Scholar
George, D., Damodara, S., Iqbal, R. & Sen, A.K. 2016 Flotation of denser liquid drops on lighter liquids in non-Neumann condition: role of line tension. Langmuir 40, 10276–10283.CrossRefGoogle Scholar
Hartland, S. & Burri, J. 1976 Das maximale volumen einer linse an einer fluid-flüssig grenzfläche. Chem. Engng J. 11 (1), 7–17.CrossRefGoogle Scholar
Hartland, S. & Robinson, J.D. 1971 The dynamic equilibrium of a rigid sphere at a deformable liquid-liquid interface. J. Colloid Interface Sci. 35 (3), 372–378.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 1987 Fluid Mechanics. Course of Theoretical Physics, vol. 6. Pergamon.Google Scholar
Lapuerta, V., Mancebo, F.J. & Vega, J.M. 2001 Control of Rayleigh–Taylor instability by vertical vibration in large aspect ratio containers. Phys. Rev. E 64, 016318.CrossRefGoogle ScholarPubMed
Lohnstein, T. 1906 Zur theorie des abtropfens mit besonderer rücksicht auf die bestimmung der kapillaritätskonstanten durch tropfversuche. Ann. Phys. 325 (7), 237–268.CrossRefGoogle Scholar
Nepomnyashchy, A. 2021 Droplet on a liquid substrate: wetting, dewetting, dynamics, instabilities. Curr. Opin. Colloid Interface Sci. 51, 101398.CrossRefGoogle Scholar
Ooi, C.H., Plackowski, C., Nguyen, A.N., Vadivelu, R.K., John, J.A.S., Dao, D.V. & Nguyen, N.-T. 2016 Floating mechanism of a small liquid marble. Sci. Rep. 6, 21777.CrossRefGoogle ScholarPubMed
Padday, J.F. & Pitt, A. 1973 The stability of axisymmetric menisci. Phil. Trans. R. Soc. Lond. A 275, 489–528.Google Scholar
Phan, C.M. 2014 Stability of a floating water droplet on an oil surface. Langmuir 30 (3), 768–773.CrossRefGoogle Scholar
Phan, C.M., Allen, B., Peters, L.B., Le, T.N. & Tade, M.O. 2012 Can water float on oil? Langmuir 28 (10), 4609–4613.CrossRefGoogle Scholar
Pototsky, A. & Bestehorn, M. 2016 Faraday instability of a two-layer liquid film with a free upper surface. Phys. Rev. Fluids 1, 023901.CrossRefGoogle Scholar
Pototsky, A. & Bestehorn, M. 2018 Shaping liquid drops by vibration. Europhys. Lett. 121 (4), 46001.CrossRefGoogle Scholar
Pototsky, A., Maksymov, I.S., Suslov, S.A. & Leontini, J. 2020 Intermittent dynamic bursting in vertically vibrated liquid drops. Phys. Fluids 32 (12), 124114.CrossRefGoogle Scholar
Pototsky, A., Oron, A. & Bestehorn, M. 2019 Vibration-induced floatation of a heavy liquid drop on a lighter liquid film. Phys. Fluids 31 (8), 087101.CrossRefGoogle Scholar
Princen, H.M. 1963 Shape of a fluid drop at a liquid–liquid interface. J. Colloid Sci. 18, 178–195.CrossRefGoogle Scholar
Princen, H.M. & Mason, S.G. 1965 Shape of a fluid drop at a fluid–liquid interface. I. Extension and test of two-phase theory. J. Colloid Sci. 20 (2), 156–172.CrossRefGoogle Scholar
Pucci, G., Ben Amar, M. & Couder, Y. 2013 Faraday instability in floating liquid lenses: the spontaneous mutual adaptation due to radiation pressure. J. Fluid Mech. 725, 402–427.CrossRefGoogle Scholar
Pucci, G., Ben Amar, M. & Couder, Y. 2015 Faraday instability in floating drops. Phys. Fluids 27 (9), 091107.CrossRefGoogle Scholar
Pucci, G., Fort, E., Ben Amar, M. & Couder, Y. 2011 Mutual adaptation of a faraday instability pattern with its flexible boundaries in floating fluid drops. Phys. Rev. Lett. 106, 024503.CrossRefGoogle ScholarPubMed
Sahasrabudhe, S.N., Rodriguez-Martinez, V., O'Meara, M. & Farkas, B.E. 2017 Density, viscosity, and surface tension of five vegetable oils at elevated temperatures: measurement and modeling. Intl J. Food Prop. 20 (sup2), 1965–1981.Google Scholar
Smith, J.D., Dhiman, R., Anand, S., Reza-Garduno, E., Cohen, R.E., McKinley, G.H. & Varanasi, K.K. 2013 Droplet mobility on lubricant-impregnated surfaces. Soft Matt. 9, 1772–1780.CrossRefGoogle Scholar
Sterman-Cohen, E., Bestehorn, M. & Oron, A. 2017 Rayleigh–Taylor instability in thin liquid films subjected to harmonic vibration. Phys. Fluids 29 (5), 052105.CrossRefGoogle Scholar
Wolf, G.H. 1969 The dynamic stabilization of the Rayleigh–Taylor instability and the corresponding dynamic equilibrium. Z. Phys. A 227 (3), 291–300.CrossRefGoogle Scholar
Wolf, G.H. 1970 Dynamic stabilization of the interchange instability of a liquid–gas interface. Phys. Rev. Lett. 24, 444–446.CrossRefGoogle Scholar
Wong, C.Y.H., Adda-Bedia, M. & Vella, D. 2017 Non-wetting drops at liquid interfaces: from liquid marbles to leidenfrost drops. Soft Matt. 13, 5250–5260.CrossRefGoogle ScholarPubMed

Pototsky et al. supplementary movie 1

Two stable shapes of a 5 micro liter water drop floating on the surface of commercial vegetable oil. All fluid parameters are as in Fig.5 in the main.

Download Pototsky et al. supplementary movie 1(Video)
Video 1.8 MB

Pototsky et al. supplementary movie 2

200fps slow motion video of a 0.12 milliliter water drop floating on the surface of olive oil vibrated at 60 Hz with amplitude a=5.5g. Drop shape and dimensions are described in Fig.7 in the main text.

Download Pototsky et al. supplementary movie 2(Video)
Video 1.1 MB

Pototsky et al. supplementary movie 3

200 fps slow motion video of a 0.15 milliliter water puddle in 5 mm layer of olive oil layer vibrated at 60 Hz with amplitude of a=5g. As the puddle undergoes a spontaneous horizontal elongation, driven by Faraday waves, the water is drawn by the increased surface tension forces towards the oil-air interface

Download Pototsky et al. supplementary movie 3(Video)
Video 5.2 MB

Pototsky et al. supplementary movie 4

5-6 micro-litter water drop floating on the surface of commercial cooking oil. After a needle is immersed into the upper part of the drop, the contact radius is increased. Horizontal bar is 5 mm.

Download Pototsky et al. supplementary movie 4(Video)
Video 72.7 KB

Pototsky et al. supplementary movie 5

0.12 milliliter water drop floating on the surface of olive oil vibrated at 50 Hz with amplitude a=5.5g. The first 11 seconds of the video are in slow motion (200 fps) the remaining part of the video is in real time. When vibration stops, the drop can no longer be supported by surface tension forces and sinks.

Download Pototsky et al. supplementary movie 5(Video)
Video 385.3 KB