Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-28T17:18:47.742Z Has data issue: false hasContentIssue false
  • English
  • Français

Evolution of fluid-like granular ejecta generated by sphere impact

Published online by Cambridge University Press:  01 May 2012

J. O. Marston ,
E. Q. Li  and
S. T. Thoroddsen
J. O. Marston*
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
E. Q. Li
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
S. T. Thoroddsen
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
*
†Email address for correspondence: jeremy.marston@kaust.edu.sa
Get access Rights & Permissions [Opens in a new window]

Abstract

We present results from an experimental study of the speed and shape of the ejecta formed when a solid sphere impacts onto a granular bed. We use high-speed imaging at frame rates up to 100 000 f.p.s. to provide direct measurement of individual grain velocities and ejecta angles as well as the overall evolution of the granular ejecta. For larger grain sizes, the emergence velocities of the grains during the ‘early stage flow’, i.e. before the main ejecta ‘curtain’ forms, increase with the kinetic energy of the impacting sphere but are inversely proportional to the time from impact. We also observe that the fastest grains, which can obtain velocities up to five times that of the impacting sphere (), generally emerge at the earliest times and with the lowest ejection angles. As the grain size is decreased, a more ‘fluid-like’ behaviour is observed whereby the ejected material first emerges as a thin sheet of grains between the sphere and the bed surface, which is also seen when a sphere impacts a liquid pool. In this case, the sheet velocity is approximately double that of the impacting sphere (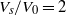) and independent of the bulk packing fraction. For the finest grains we provide evidence of the existence of a vortex ring inside the ejecta curtain where grains following the air flow are entrained through the curtain. In contrast to predictions from previous studies, we find that the temporal evolution of the ejecta neck radius is not initially quadratic but rather approaches a square-root dependence on time, for the finest grains with the highest impact kinetic energy. The evolution therefore approaches that seen for the crown evolution in liquid drop impacts. By using both spherical glass beads and coarse sands, we show that the size and shape distribution are critical in determining the post-impact dynamics whereby the sands exhibit a qualitatively different response to impact, with grains ejected at lower speeds and at later times than for the glass beads.

JFM classification

Multiphase and Particle-laden Flows: Fluidized beds Granular media: Granular media Wakes/Jets: Jets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 704 , 10 August 2012 , pp. 5 - 36
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Ambroso, M. A., Santore, C. R., Abate, A. R. & Durian, D. J. 2005 Penetration depth for shallow impact cratering. Phys. Rev. E 71, 051305.CrossRefGoogle ScholarPubMed
2. Barnouin-Jha, O. S. & Schultz, P. 1999 Interactions between an impact generated ejecta curtain and an atmosphere. J. Impact Engng 23, 51–62.CrossRefGoogle Scholar
3. Beladjine, D., Ammi, M., Oger, L & Alexandre, V. 2007 Collision process between an incident bead and a three-dimensional granular packing. Phys. Rev. E 75, 061305.CrossRefGoogle Scholar
4. Boudet, J. F., Amarouchene, Y., Bonnier, B. & Kellay, H. 2007 The granular jump. J. Fluid Mech. 413, 413–431.CrossRefGoogle Scholar
5. Boudet, J. F., Amarouchene, Y. & Kellay, H. 2006 Dynamics of impact cratering in shallow sand layers. Phys. Rev. Lett. 96, 158001.CrossRefGoogle ScholarPubMed
6. Caballero, G., Bergmann, R., van der Meer, D., Prosperetti, A. & Lohse, D. 2007 Role of air in granular jet formation. Phys. Rev. Lett. 99, 018001.CrossRefGoogle ScholarPubMed
7. Cheng, X., Varas, G., Citron, D., Jaeger, H. M. & Nagel, S. R. 2007 Collective behaviour in a granular jet: emergence of a liquid with zero surface tension. Phys. Rev. Lett. 99, 188001.CrossRefGoogle Scholar
8. Colwell, J. E., Sture, S., Cintala, M., Durda, D., Hendrix, A., Goudie, T., Curtis, D., Ashcom, D. J., Kanter, M., Keohane, T., Lemos, A., Lupton, M. & Route, M. 2008 Ejecta from impacts at 0.2–2.3 m s−1 in low gravity. Icarus 195, 908–917.CrossRefGoogle Scholar
9. Cossali, G. E., Marengo, M., Coghe, A. & Zhdanov, S. 2004 The role of time in single drop splash on thin film. Exp. Fluids 36, 888–900.CrossRefGoogle Scholar
10. Deboeuf, S., Gondret, P. & Rabaud, M. 2009 Dynamics of grain ejection by sphere impact on a granular bed. Phys. Rev. E 79, 041306.CrossRefGoogle ScholarPubMed
11. Eggers, J. & Villermaux, E. 2008 Physics of liquid jets. Rep. Prog. Phys. 71, 036601.CrossRefGoogle Scholar
12. Forterre, Y. & Pouliquen, O. 2008 Flows of dense granular media. Annu. Rev. Fluid Mech. 40, 1–24.CrossRefGoogle Scholar
13. Grace, J. R. 1970 The viscosity of fluidised beds. Can. J. Chem. Engng 48, 30.CrossRefGoogle Scholar
14. Goldman, D. I. & Umbahnowar, P. 2008 Scaling and dynamics of sphere and disk impact into granular media. Phys. Rev. E. 77, 021308.CrossRefGoogle ScholarPubMed
15. Hapgood, K. P., Lister, J. D., Biggs, S. R. & Howes, T. 2002 Drop penetration into porous powder beds. J. Colloid Interface Sci. 253, 353–366.CrossRefGoogle ScholarPubMed
16. Hartmann, W. K. 1985 Impact Experiments 1. Ejecta velocity distributions and related results from regolith targets. Icarus 63, 69–98.CrossRefGoogle Scholar
17. Hermalyn, B. & Schultz, P. H. 2010 Early-stage ejecta velocity distribution for vertical hypervelocity impacts into sand. Icarus 209, 866–870.CrossRefGoogle Scholar
18. Hermalyn, B. & Schultz, P. H. 2011 Time-resolved studies of hypervelocity impacts into porous particulate targets: effects of projectile density on early-time coupling and crater growth. Icarus 216, 269–279.CrossRefGoogle Scholar
19. Holsapple, K. A. 1993 The scaling of impact processes in planetary sciences. Annu. Rev. Earth Planet. Sci. 21, 333–373.CrossRefGoogle Scholar
20. Housen, K. R. & Holsapple, K. A. 2011 Ejecta from impact craters. Icarus 211, 856–875.CrossRefGoogle Scholar
21. Jop, P., Forterre, Y. & Pouliquen, O. 2006 A constitutive law for dense granular flows. Nature 441, 727–730.CrossRefGoogle ScholarPubMed
22. von Kann, S., Joubaud, S., Caballero-Robledo, G. A., Lohse, D. & van der Meer, D. 2010 Effect of finite container size on granular jet formation. Phys. Rev. E 81, 041306.CrossRefGoogle ScholarPubMed
23. King, D. F., Mitchell, F. R. G. & Harrison, D. 1981 Dense phase viscosities of fluidised beds at elevated pressures. Powder Technol. 28, 55–58.CrossRefGoogle Scholar
24. Lacaze, L. & Kerswell, R. 2009 Axisymmetric granular collapse: a transient 3D test of viscoplasticity. Phys. Rev. Lett. 102, 108305.CrossRefGoogle ScholarPubMed
25. Lohse, D., Bergmann, R., Mikkelsen, R., Zeilstra, C., van der Meer, D., Versluis, M., van der Weele, K., van der Hoef, M. & Kuipers, H. 2004 Impact on soft sand: void collapse and jet formation. Phys. Rev. Lett. 93 (19), 198003.CrossRefGoogle ScholarPubMed
26. Lun, C. K., Savage, S. B., Jeffrey, D. J. & Chepurniy, N 1984 Kinetic theories for granular flow: inelastic particles in Couette flow and slightly inelastic particles in a general flow field. J. Fluid Mech. 140, 223.CrossRefGoogle Scholar
27. Marston, J. O., Seville, J. P. K., Cheun, Y.-V., Ingram, A., Decent, S. P. & Simmons, M. J. H. 2008 Effect of packing fraction on granular jetting from solid sphere entry into aerated and fluidised beds. Phys. Fluids 20, 023301.CrossRefGoogle Scholar
28. Michikami, T., Moriguchi, K., Hasegawa, S. & Fujiwara, A. 2007 Ejecta velocity distribution for impact cratering experiments on porous and low strength targets. Planet. Space Sci. 55, 70–88.CrossRefGoogle Scholar
29. Mobius, M. E. 2006 Clustering instability in a freely falling granular jet. Phys. Rev. E 74, 051304.CrossRefGoogle Scholar
30. O’keefe, J. D. & Ahrens, T. J. 1985 Impact and explosion crater ejecta, fragmentation size, and velocity. Icarus 62, 328–338.CrossRefGoogle Scholar
31. Royer, J. R., Corwin, E. I., Conyers, B., Flior, A., Rivers, M. L., Eng, P. J. & Jaeger, H. M. 2008 Birth and growth of a granular jet. Phys. Rev. E 78, 011305.CrossRefGoogle ScholarPubMed
32. Royer, J. R., Corwin, E. I., Eng, P. J. & Jaeger, H. M. 2007 Gas-mediated impact dynamics in fine-grained granular materials. Phys. Rev. Lett. 99, 038003.CrossRefGoogle ScholarPubMed
33. Royer, J. R., Evans, D. J., Oyarte, L., Guo, Q., Kapit, E., Mobius, M. E., Waitukaitis, S. R. & Jaeger, H. M. 2009 High-speed tracking of rupture and clustering in freely falling granular streams. Nature 459, 1110–1113.CrossRefGoogle ScholarPubMed
34. Savage, S. B. 1989 Flow of granular materials. In Theoretical and Applied Mechanics, pp. 241–266. Elsevier.CrossRefGoogle Scholar
35. Savage, S. B. & Sayed, M. 1984 Stresses developed by dry cohesionless granular materials sheared in an annular shear cell. J. Fluid Mech. 142, 391–430.CrossRefGoogle Scholar
36. Seguin, A., Bertho, Y. & Gondret, P. 2008 Influence of confinement on granular penetration by impact. Phys. Rev. E 78, 010301.CrossRefGoogle ScholarPubMed
37. Stephensen, R. 2000 Shallow foundations. In Practical Foundation Engineering Handbook, 2nd edn (ed. Brown, R. ), pp. 135–164. McGraw-Hill Publishing.Google Scholar
38. Stickel, J. J. & Powell, R. L. 2005 Fluid mechanics and rheology of dense suspensions. Annu. Rev. Fluid Mech. 37, 129–149.CrossRefGoogle Scholar
39. Thoroddsen, S. T., Etoh, T. G., Takehara, K. & Takano, Y. 2004 Impact jetting by a solid sphere. J. Fluid Mech. 499, 139–148.CrossRefGoogle Scholar
40. Thoroddsen, S. T. & Shen, A. Q. 2001 Granular jets. Phys. Fluids 13 (1), 4–6.CrossRefGoogle Scholar
41. Thoroddsen, S. T., Thoraval, M.-J., Takehara, K. & Etoh, T. G. 2011 Droplet splashing by a slingshot mechanism. Phys. Rev. Lett. 106, 034501.CrossRefGoogle ScholarPubMed
42. Uehara, J. S., Ambroso, M. A., Ojha, R. P. & Durian, D. J. 2003 Low-speed impact crater in loose granular media. Phys. Rev. Lett. 90, 194301.CrossRefGoogle ScholarPubMed
43. Umbanhowar, P. & Goldman, D. I. 2010 Granular impact and the critical packing state. Phys. Rev. E 82, 010301.CrossRefGoogle ScholarPubMed
44. de Vet, S. J. & de Bruyn, J. R. 2007 Shape of impact craters in granular media. Phys. Rev. E. 76, 041306.CrossRefGoogle ScholarPubMed
45. Walsh, A. M., Holloway, K. E., Habdas, P. & de Bruyn, J. R. 2003 Morphology and scaling of impact craters in granular media. Phys. Rev. Lett. 91 (10), 104301.CrossRefGoogle ScholarPubMed
46. Weiss, D. A. & Yarin, A. L. 1999 Single drop impact onto liquid films: neck distortion, jetting, tiny bubble entrapment, and crown formation. J. Fluid Mech. 385, 229–254.CrossRefGoogle Scholar
47. Yamamoto, S., Kadono, T., Sugita, S. & Matsui, T. 2005 Velocity distributions of high-velocity ejecta from regolith targets. Icarus 178, 264–273.CrossRefGoogle Scholar
48. Yamamoto, S., Wada, K., Okabe, N. & Matsui, T. 2006 Transient crater growth in granular targets: an experimental study of low velocity impacts into glass sphere targets. Icarus 183, 215–224.CrossRefGoogle Scholar
49. Zeilstra, C. 2007Granular dynamics in vibrated beds. PhD thesis, University of Twente.Google Scholar

Marston et al. supplementary movie

Movie 1. Sequences from video clips taken at 12,000 fps. Four different media are shown in this clip. The first 3 are glass beads with diameters 520, 178 and 31 μm and the final panel is water. In each sequence, the impacting sphere has a diameter of 25 mm and velocity of 9.8m/s prior to impact.

Download Marston et al. supplementary movie(Video)
Video 1.3 MB

Marston et al. supplementary movie

Movie 2. Video clip taken at 100,000 fps showing the emergence of a fluid-like granular sheet for fine grains. The sphere diameter is 50 mm, the impact velocity is 2.6 m/s and the grains are 31 μm.

Download Marston et al. supplementary movie(Video)
Video 91.5 KB

Marston et al. supplementary movie

Movie 3. Video clip taken at 1000,000 fps showing the high-speed ejecta generated during the impact of a 50 mm sphere onto a pool of water. The impact velocity was 9.45 m/s.

Download Marston et al. supplementary movie(Video)
Video 384.1 KB

Marston et al. supplementary movie

Movie 4. Video clip taken at 12,000 fps showing the collapse of the granular ejecta for the fine grains (31 μm). The sphere diameter is 25 mm and the impact velocity is 9.6 m/s.

Download Marston et al. supplementary movie(Video)
Video 23.8 MB

Marston et al. supplementary movie

Movie 5. Video clip taken at 12,000 fps showing fine grains (31 μm) flowing back through the porous ejecta, followed by the emergence of the granular jet. The sphere diameter is 25 mm and the impact velocity is 9.6 m/s.

Download Marston et al. supplementary movie(Video)
Video 11 MB