Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gallet, Basile
2015.
Exact two-dimensionalization of rapidly rotating large-Reynolds-number flows.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
p.
412.
Seshasayanan, Kannabiran
and
Alexakis, Alexandros
2016.
Critical behavior in the inverse to forward energy transition in two-dimensional magnetohydrodynamic flow.
Physical Review E,
Vol. 93,
Issue. 1,
Ecke, R. E.
2017.
From 2D to 3D in fluid turbulence: unexpected critical transitions.
Journal of Fluid Mechanics,
Vol. 828,
Issue. ,
p.
1.
Benavides, Santiago Jose
and
Alexakis, Alexandros
2017.
Critical transitions in thin layer turbulence.
Journal of Fluid Mechanics,
Vol. 822,
Issue. ,
p.
364.
Sundar, Sita
Verma, Mahendra K.
Alexakis, Alexandros
and
Chatterjee, Anando G.
2017.
Dynamic anisotropy in MHD turbulence induced by mean magnetic field.
Physics of Plasmas,
Vol. 24,
Issue. 2,
p.
022304.
Pothérat, Alban
and
Klein, Rico
2017.
Do magnetic fields enhance turbulence at low magnetic Reynolds number?.
Physical Review Fluids,
Vol. 2,
Issue. 6,
Biferale, L.
Buzzicotti, M.
and
Linkmann, M.
2017.
From two-dimensional to three-dimensional turbulence through two-dimensional three-component flows.
Physics of Fluids,
Vol. 29,
Issue. 11,
p.
111101.
Linkmann, Moritz
Buzzicotti, Michele
and
Biferale, Luca
2018.
Nonuniversal behaviour of helical two-dimensional three-component turbulence.
The European Physical Journal E,
Vol. 41,
Issue. 1,
Sagaut, Pierre
and
Cambon, Claude
2018.
Homogeneous Turbulence Dynamics.
p.
583.
Zhai, X. M.
and
Yeung, P. K.
2018.
Evolution of anisotropy in direct numerical simulations of MHD turbulence in a strong magnetic field on elongated periodic domains.
Physical Review Fluids,
Vol. 3,
Issue. 8,
Alexakis, Alexandros
2018.
Three-dimensional instabilities and negative eddy viscosity in thin-layer flows.
Physical Review Fluids,
Vol. 3,
Issue. 11,
Alexakis, A.
and
Biferale, L.
2018.
Cascades and transitions in turbulent flows.
Physics Reports,
Vol. 767-769,
Issue. ,
p.
1.
van Kan, Adrian
Nemoto, Takahiro
and
Alexakis, Alexandros
2019.
Rare transitions to thin-layer turbulent condensates.
Journal of Fluid Mechanics,
Vol. 878,
Issue. ,
p.
356.
van Kan, Adrian
and
Alexakis, Alexandros
2019.
Condensates in thin-layer turbulence.
Journal of Fluid Mechanics,
Vol. 864,
Issue. ,
p.
490.
Baklouti, F. S.
Khlifi, A.
Salhi, A.
Godeferd, F.
Cambon, C.
and
Lehner, T.
2019.
Kinetic-magnetic energy exchanges in rotating magnetohydrodynamic turbulence.
Journal of Turbulence,
Vol. 20,
Issue. 4,
p.
263.
Pouquet, A.
Rosenberg, D.
Stawarz, J.E.
and
Marino, R.
2019.
Helicity Dynamics, Inverse, and Bidirectional Cascades in Fluid and Magnetohydrodynamic Turbulence: A Brief Review.
Earth and Space Science,
Vol. 6,
Issue. 3,
p.
351.
Xie, Jin-Han
and
Bühler, Oliver
2019.
Third-order structure functions for isotropic turbulence with bidirectional energy transfer.
Journal of Fluid Mechanics,
Vol. 877,
Issue. ,
Poujol, Basile
van Kan, Adrian
and
Alexakis, Alexandros
2020.
Role of the forcing dimensionality in thin-layer turbulent energy cascades.
Physical Review Fluids,
Vol. 5,
Issue. 6,
Lin, Hongxia
Ji, Ruihong
Wu, Jiahong
and
Yan, Li
2020.
Stability of perturbations near a background magnetic field of the 2D incompressible MHD equations with mixed partial dissipation.
Journal of Functional Analysis,
Vol. 279,
Issue. 2,
p.
108519.
Linkmann, Moritz
Hohmann, Manuel
and
Eckhardt, Bruno
2020.
Non-universal transitions to two-dimensional turbulence.
Journal of Fluid Mechanics,
Vol. 892,
Issue. ,
 $\mathit{Rm}\ll 1$: we prove that the flow becomes exactly 2-D asymptotically in time, regardless of the initial condition and provided that the interaction parameter
$\mathit{Rm}\ll 1$: we prove that the flow becomes exactly 2-D asymptotically in time, regardless of the initial condition and provided that the interaction parameter 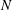 $N$ is larger than a threshold value. We call this property absolute two-dimensionalization: the attractor of the system is necessarily a (possibly turbulent) 2-D flow. We then consider the full magnetohydrodynamic (MHD) equations and prove that, for low enough
$N$ is larger than a threshold value. We call this property absolute two-dimensionalization: the attractor of the system is necessarily a (possibly turbulent) 2-D flow. We then consider the full magnetohydrodynamic (MHD) equations and prove that, for low enough 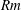 $\mathit{Rm}$ and large enough
$\mathit{Rm}$ and large enough 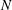 $N$, the flow becomes exactly 2-D in the long-time limit provided the initial vertically dependent perturbations are infinitesimal. We call this phenomenon linear two-dimensionalization: the (possibly turbulent) 2-D flow is an attractor of the dynamics, but it is not necessarily the only attractor of the system. Some 3-D attractors may also exist and be attained for strong enough initial 3-D perturbations. These results shed some light on the existence of a dissipation anomaly for MHD flows subject to a strong external magnetic field.
$N$, the flow becomes exactly 2-D in the long-time limit provided the initial vertically dependent perturbations are infinitesimal. We call this phenomenon linear two-dimensionalization: the (possibly turbulent) 2-D flow is an attractor of the dynamics, but it is not necessarily the only attractor of the system. Some 3-D attractors may also exist and be attained for strong enough initial 3-D perturbations. These results shed some light on the existence of a dissipation anomaly for MHD flows subject to a strong external magnetic field.


