Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-28T16:59:08.503Z Has data issue: false hasContentIssue false
  • English
  • Français

Feedback control for form-drag reduction on a bluff body with a blunt trailing edge

Published online by Cambridge University Press:  03 July 2012

Jeremy A. Dahan ,
A. S. Morgans  and
S. Lardeau
Jeremy A. Dahan*
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
A. S. Morgans
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
S. Lardeau
Affiliation:
CD-adapco, 200 Shepherds Bush Road, London W6 7NL, UK
*
†Email address for correspondence: jeremy.dahan05@imperial.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

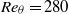The objective of this numerical study is to increase the base pressure on a backward-facing step via linear feedback control, to be ultimately translated to a drag reduction on a blunt-based bluff body. Two backward-facing step cases are simulated: a laminar two-dimensional (2D) flow at a Reynolds number of , and a turbulent three-dimensional (3D) flow at 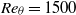using large-eddy simulation. The control is effected by a full-span slot jet with zero-net-mass-flux, and two jet locations are examined. Linear system identification is performed to characterize the flow response to actuation, used to synthesize a control law. The control strategy is based on the premise that an attenuation of the instantaneous pressure fluctuations on the base of the step should lead to an increase in the time-averaged base pressure. Open-loop harmonic forcing is examined within a broad frequency range for both the 2D and 3D flows, which are found to respond differently to actuation. The controllers based on disturbance attenuation lead to sensible increases in base pressure (up to 70 % in 2D and 20 % in 3D) with higher efficiency than the best results achieved in open-loop. The results support the conjecture about the link between the base pressure fluctuations and mean, although it is shown that such a black-box model approach is not suitable for optimization without further physical insight.

JFM classification

Flow Control: Control theory Flow Control: Drag reduction Wakes/Jets: Shear layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 704 , 10 August 2012 , pp. 360 - 387
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Aram, E., Mittal, R. & Cattafesta, L. 2010 Simple representations of zero-net-mass-flux jets in grazing flow for flow-control simulations. Intl J. Flow Control 2 (2), 109–125.CrossRefGoogle Scholar
2. Bhattacharjee, S., Scheelke, B. & Troutt, T. R. 1986 Modifications of vortex interactions in a reattaching separated flow. AIAA J. 24 (4), 623–629.CrossRefGoogle Scholar
3. Bradshaw, P. & Wong, F. Y. F. 1972 The reattachment and relaxation of a turbulent shear layer. J. Fluid Mech. 52, 113–135.CrossRefGoogle Scholar
4. Brosco, E. 2011 Experimental investigation on separation control by high frequency forcing. PhD thesis, University of Rome, La Sapienza.Google Scholar
5. Cattafesta, L. N. & Sheplak, M. 2011 Actuators for active flow control. Annu. Rev. Fluid Mech. 43, 247–272.CrossRefGoogle Scholar
6. Cattafesta, L. N., Song, Q., Williams, D. R., Rowley, C. W. & Alvi, F. S. 2008 Active control of flow-induced cavity oscillations. Prog. Aerosp. Sci. 44, 479–502.CrossRefGoogle Scholar
7. Chun, K. B. & Sung, H. J. 1996 Control of turbulent separated flow over a backward-facing step by local forcing. Exp. Fluids 25, 133–142.CrossRefGoogle Scholar
8. Cohen, K., Siegel, S. & McLaughlin, T. 2006 A heuristic approach to effective sensor placement for modelling of a cylinder wake. Comput. Fluids 35, 103–120.CrossRefGoogle Scholar
9. Dandois, J., Garnier, E. & Sagaut, P. 2007 Numerical simulation of active separation control by a synthetic jet. J. Fluid Mech. 574, 25–58.CrossRefGoogle Scholar
10. Dejoan, A. & Leschziner, M. A. 2004 Large eddy simulation of periodically perturbed separated flow over a backward-facing step. Intl J. Heat Transfer Fluid Flow 25, 581–592.CrossRefGoogle Scholar
11. Dowling, A. P. & Morgans, A. S. 2005 Feedback control of combustion oscillations. Annu. Rev. Fluid Mech. 37, 151–182.CrossRefGoogle Scholar
12. Driver, D. M. & Seegmiller, H. L. 1985 Features of a reattaching turbulent shear layer in divergent channel flow. AIAA J. 23 (2), 163–171.CrossRefGoogle Scholar
13. Eaton, J. K. & Johnston, J. P. 1981 A review of research on subsonic turbulent flow reattachment. AIAA J. 19 (9), 1093–1100.CrossRefGoogle Scholar
14. Englar, R. J. 2000 Development of pneumatic aerodynamic devices to improve the performance, economics, and safety of heavy vehicles. SAE Technical Paper Series, 2000-01-2208.Google Scholar
15. Fishpool, G. M. & Leschziner, M. A. 2009 Stability bounds for explicit fractional-step schemes for the Navier–Stokes equations at high Reynolds number. Comput. Fluids 38, 1289–1298.CrossRefGoogle Scholar
16. Gad-el Hak, M., Pollard, A. & Bonnet, J.-P. 1998 Flow Control, Fundamentals and Practices. Springer.Google Scholar
17. Gelb, A. & Vander Velde, W. E. 1968 Multiple-Input Describing Functions and Nonlinear System Design. McGraw-Hill.Google Scholar
18. Hasan, M. A. Z. 1992 The flow over a backward-facing step under controlled perturbation: laminar separation. J. Fluid Mech. 238, 73–96.CrossRefGoogle Scholar
19. Hasan, M. A. Z. & Khan, A. S. 1992 On the instability characteristics of a reattaching shear layer with nonlaminar separation. Intl J. Heat Transfer Fluid Flow 13 (3), 224–231.CrossRefGoogle Scholar
20. Heenan, A. F. & Morrison, J. F. 1998 Passive control of pressure fluctuations generated by separated flow. AIAA J. 36 (6), 1014–1022.CrossRefGoogle Scholar
21. Henning, L. & King, R. 2005 Drag reduction by closed-loop control of a separated flow over a bluff body with a blunt trailing edge. In Proceedings of the 44th IEEE Conference on Decision and Control, and the European Control Conference 2005, pp. 494–499. IEEE.Google Scholar
22. Henning, L. & King, R. 2007 Robust multivariable closed-loop control of a turbulent backward-facing step flow. J. Aircraft 44 (1), 201–208.CrossRefGoogle Scholar
23. Illingworth, S. J. 2009 Feedback control of oscillations in combustion and cavity flows. PhD thesis, Magdalene College, University of Cambridge.Google Scholar
24. Jimenez, J., Hoyas, S., Simens, M. P. & Mizuno, Y. 2010 Turbulent boundary layers and channels at moderate Reynolds numbers. J. Fluid Mech. 657, 335–360.CrossRefGoogle Scholar
25. Juang, J.-N. & Pappa, R. S. 1985 An eigensystem realization algorithm for modal parameter identification and model reduction. J. Guid. Control Dyn. 8 (5), 620–627.CrossRefGoogle Scholar
26. Kim, J., Hahn, S., Kim, J., Lee, D., Choi, J., Jeon, W. & Choi, H. 2004 Active control of turbulent flow over a model vehicle for drag reduction. J. Turbul. 5, 019.CrossRefGoogle Scholar
27. Kim, K., Kerr, M., Beskok, A. & Jayasuriya, S. 2006 Frequency-domain based feedback control of flow separation using synthetic jets. In Proceedings of the 2006 American Control Conference, pp. 5318–5323. IEEE.Google Scholar
28. Lardat, R. & Leschziner, M. A. 1998 A Navier–Stokes solver for LES on parallel computers. Tech. Rep. Department of Mechanical Engineering, UMIST.Google Scholar
29. Le, H., Moin, P. & Kim, J. 1997 Direct numerical simulation of turbulent flow over a backward-facing step. J. Fluid Mech. 330, 349–374.CrossRefGoogle Scholar
30. Leschziner, M. A. & Lardeau, S. 2011 Simulation of slot and round synthetic jets in the context of boundary layer separation. Phil. Trans. R. Soc. A 369, 1495–1512.CrossRefGoogle ScholarPubMed
31. Ljung, L. 1999 System Identification: Theory for the User. Prentice Hall.Google Scholar
32. Lund, T. S., Wu, X. & Squires, K. D. 1998 Generation of turbulent inflow data for spatially-developing boundary layer simulations. J. Comput. Phys. 140, 233–258.CrossRefGoogle Scholar
33. Ma, Z., Ahuja, S. & Rowley, C. W. 2009 Reduced order models for control of fluids using the eigensystem realization algorithm. Theor. Comput. Fluid Dyn. 25, 233–247.CrossRefGoogle Scholar
34. McFarlane, D. C. & Glover, K. 1989 Robust Controller Design Using Normalized Coprime Factor Plant Descriptions. Springer.Google Scholar
35. Modi, V. J., Hill, S. St. & Yokomizo, T. 1995 Drag reduction of trucks through boundary-layer control. J. Wind Engng Ind. Aerodyn. 54/55, 583–594.CrossRefGoogle Scholar
36. Nicoud, F. & Ducros, F. 1999 Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 62, 183, 200.CrossRefGoogle Scholar
37. Park, H., Lee, D., Jeon, W.-P., Hahn, S., Kim, J., Kim, J., Choi, J. & Choi, H. 2006 Drag reduction in flow over a two-dimensional bluff body with a blunt trailing edge using a new passive device. J. Fluid Mech. 563, 389–414.CrossRefGoogle Scholar
38. Pastoor, M., Henning, L., Noack, B. R., King, R. & Tadmor, G. 2008 Feedback shear layer control for bluff body drag reduction. J. Fluid Mech. 608, 161–196.CrossRefGoogle Scholar
39. Qubain, A. 2009 Active control of a turbulent bluff body wake. PhD thesis, Imperial College London.Google Scholar
40. Roshko, A. 1954 On the drag and shedding frequency of two-dimensional bluff bodies. Tech. Rep. National Advisory Committee for Aeronautics.Google Scholar
41. Seifert, A., Stalnov, O., Sperber, D., Arwatz, G., Palei, V., David, S., Dayan, I. & Fono, I. 2009 Large trucks drag reduction using active flow control. In The Aerodynamics of Heavy Vehicles II: Trucks, Buses, and Trains (ed. Browand, F., McCallen, R. & Ross, J. ). Lecture Notes in Applied and Computational Mechanics, vol. 41 , pp. 115–133. Springer.CrossRefGoogle Scholar
42. Siegel, S., Cohen, K. & McLaughlin, T. 2006 Numerical simulations of a feedback-controlled circular cylinder wake. AIAA J. 44 (6), 1266–1276.CrossRefGoogle Scholar
43. Simpson, R. L. 1989 Turbulent boundary-layer separation. Annu. Rev. Fluid Mech. 21, 205–234.CrossRefGoogle Scholar
44. Spazzini, P. G., Iuso, G., Onorato, M., Zurlo, N. & Di Cicca, G. M. 2001 Unsteady behaviour of back-facing step flow. Exp. Fluids 30, 551–561.CrossRefGoogle Scholar
45. Stalnov, O., Fono, I. & Seifert, A. 2011 Closed-loop bluff-body wake stabilization via fluidic excitation. Theor. Comput. Fluid Dyn. 25, 209–219.CrossRefGoogle Scholar
46. Tanner, M. 1972 A method for reducing the base drag of wings with blunt trailing edge. Aeronaut. Q. 23, 15–23.CrossRefGoogle Scholar
47. Temmerman, L. 2004 Large eddy simulation of separating flows from curved surfaces. PhD thesis, University of London.Google Scholar
48. Troutt, T. R., Scheelke, B. & Norman, T. R. 1984 Organized structures in a reattaching separated flow field. J. Fluid Mech. 143, 413–427.CrossRefGoogle Scholar
49. Uruba, V., Jonáš, P. & Mazur, O. 2007 Control of a channel-flow behind a backward-facing step by suction/blowing. Intl J. Heat Transfer Fluid Flow 28, 665–672.CrossRefGoogle Scholar
50. Wengle, H., Huppertz, A., Bärwolff, G. & Janke, G. 2001 The manipulated transitional backward-facing step flow: an experimental and direct numerical simulation investigation. Eur. J. Mech. B 20 (1), 25–46.CrossRefGoogle Scholar
51. Wood, C. J. 1967 Visualization of an incompressible wake with base bleed. J. Fluid Mech. 29 (2), 259–272.CrossRefGoogle Scholar
52. Yoshioka, S., Obi, S. & Masuda, S. 2001 Organized vortex motion in periodically perturbed turbulent separated flow over a backward-facing step. Intl J. Heat Transfer Fluid Flow 22, 301–307.CrossRefGoogle Scholar