No CrossRef data available.
Published online by Cambridge University Press: 05 December 2024
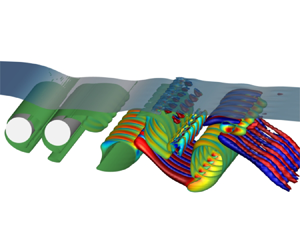
This paper presents a numerical study on the flow around two tandem circular cylinders beneath a free surface at a Reynolds number of  $180$. The free-surface effects on the wake dynamics and hydrodynamic forces are investigated through a parametric study, covering a parameter space of gap ratios from
$180$. The free-surface effects on the wake dynamics and hydrodynamic forces are investigated through a parametric study, covering a parameter space of gap ratios from  $0.20$ to
$0.20$ to  $2.00$, spacing ratios from
$2.00$, spacing ratios from  $1.50$ to
$1.50$ to  $4.00$ and Froude numbers from
$4.00$ and Froude numbers from  $0.2$ to
$0.2$ to  $0.8$. A jet-like flow accompanied by a shear layer of positive vorticity separating from the free surface is formed in the wake at small gap ratios, which significantly alters the wake pattern through its dynamic behaviours. At shallow submergence depths, the three-dimensional wake transitions from mode B to mode A as the distance between the cylinders increases. As submergence depth increases, the wavy deformation of the primary vortex cores disappears in the wake, and the flow transitions to a two-dimensional state. Higher Froude numbers can extend the effect of the free surface to deeper submergence depths. The critical spacing ratio tends to be larger at higher Froude numbers. Furthermore, the free-surface deformation is examined. The free-surface profile typically comprises a hydraulic jump immediately ahead of the upstream cylinder, trapped waves in the vicinity of the two tandem cylinders and well-defined travelling waves on the downstream side. The frequencies of the waves cluster around the vortex shedding frequency, indicating a close association between the generation of waves and the vortex shedding process.
$0.8$. A jet-like flow accompanied by a shear layer of positive vorticity separating from the free surface is formed in the wake at small gap ratios, which significantly alters the wake pattern through its dynamic behaviours. At shallow submergence depths, the three-dimensional wake transitions from mode B to mode A as the distance between the cylinders increases. As submergence depth increases, the wavy deformation of the primary vortex cores disappears in the wake, and the flow transitions to a two-dimensional state. Higher Froude numbers can extend the effect of the free surface to deeper submergence depths. The critical spacing ratio tends to be larger at higher Froude numbers. Furthermore, the free-surface deformation is examined. The free-surface profile typically comprises a hydraulic jump immediately ahead of the upstream cylinder, trapped waves in the vicinity of the two tandem cylinders and well-defined travelling waves on the downstream side. The frequencies of the waves cluster around the vortex shedding frequency, indicating a close association between the generation of waves and the vortex shedding process.