No CrossRef data available.
Published online by Cambridge University Press: 10 February 1997
In premixed turbulent combustion, the modelling of the turbulent flux of the mean reaction progress variable 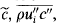 remains somewhat controversial. Classical gradient transport assumptions based on the eddy viscosity concept are often used while both experimental data and theoretical analysis have pointed out the existence of countergradient turbulent diffusion. Direct numerical simulation (DNS) is used in this paper to provide basic information on the turbulent flux of
remains somewhat controversial. Classical gradient transport assumptions based on the eddy viscosity concept are often used while both experimental data and theoretical analysis have pointed out the existence of countergradient turbulent diffusion. Direct numerical simulation (DNS) is used in this paper to provide basic information on the turbulent flux of 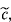 and study the occurrence of counter-gradient transport. The numerical configuration corresponds to twoor three-dimensional premixed flames in isotropic turbulent flow. The simulations correspond to various flame and flow conditions that are representative of flamelet combustion. They reveal that different flames will feature different turbulent transport properties and that these differences can be related to basic dynamical differences in the flame-flow interactions: counter-gradient diffusion occurs when the flow field near the flame is dominated by thermal dilatation due to chemical reaction, whereas gradient diffusion occurs when the flow field near the flame is dominated by the turbulent motions. The DNS-based analysis leads to a simple expression to describe the turbulent flux of
and study the occurrence of counter-gradient transport. The numerical configuration corresponds to twoor three-dimensional premixed flames in isotropic turbulent flow. The simulations correspond to various flame and flow conditions that are representative of flamelet combustion. They reveal that different flames will feature different turbulent transport properties and that these differences can be related to basic dynamical differences in the flame-flow interactions: counter-gradient diffusion occurs when the flow field near the flame is dominated by thermal dilatation due to chemical reaction, whereas gradient diffusion occurs when the flow field near the flame is dominated by the turbulent motions. The DNS-based analysis leads to a simple expression to describe the turbulent flux of 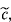 , which in turn leads to a simple criterion to delineate between the gradient and counter-gradient turbulent diffusion regimes. This criterion suggests that the occurrence of one regime or the other is determined primarily by the ratio of turbulence intensity divided by the laminar flame speed,
, which in turn leads to a simple criterion to delineate between the gradient and counter-gradient turbulent diffusion regimes. This criterion suggests that the occurrence of one regime or the other is determined primarily by the ratio of turbulence intensity divided by the laminar flame speed, 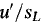 and by the flame heat release factor, τ ≡ (Tb — Tu)/Tu, where Tu and Tb are respectively the temperature within unburnt and burnt gas. Consistent with the Bray-Moss-Libby theory, counter-gradient (gradient) diffusion is promoted by low (high) values
and by the flame heat release factor, τ ≡ (Tb — Tu)/Tu, where Tu and Tb are respectively the temperature within unburnt and burnt gas. Consistent with the Bray-Moss-Libby theory, counter-gradient (gradient) diffusion is promoted by low (high) values 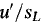 and high (low) values of τ. DNS also shows that these results are not restricted to the turbulent transport of
and high (low) values of τ. DNS also shows that these results are not restricted to the turbulent transport of 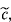 . Similar results are found for the turbulent transport of flame surface density, Σ. The turbulent fluxes of
. Similar results are found for the turbulent transport of flame surface density, Σ. The turbulent fluxes of 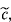 and Σ are strongly correlated in the simulated flames and counter-gradient (gradient) diffusion of
and Σ are strongly correlated in the simulated flames and counter-gradient (gradient) diffusion of 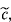 always coincides with counter-gradient (gradient) diffusion of Σ.
always coincides with counter-gradient (gradient) diffusion of Σ.