Published online by Cambridge University Press: 26 December 2024
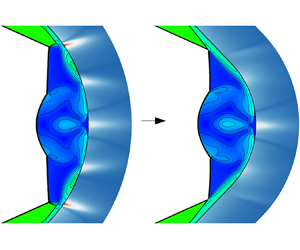
This study investigates the heating issue associated with a V-shaped blunt leading edge (VBLE) in a hypersonic flow. The heat flux generation on the VBLE is highly correlated with the shock interaction configurations in the crotch region, determined by the relative position of the triple point T and the curved shock (CS). The primary Mach reflection (MR), accompanied by a series of secondary shock–shock interactions and shock wave–boundary layer interactions, can produce extremely high heating peaks on the crotch. To configure the shock wave structures and reduce the heat flux, a shock-controllable design approach is developed based on the simplified continuity method. The strategy involves the inverse design of the crotch sweep path, according to the location of the triple point and the contour of the CS. The comparisons between the pre-designed shock configurations and the numerical results demonstrate the reliability of the design approach across various free stream Mach numbers ranging from 6 to 10. A VBLE model designed with the shock configuration of regular reflection from the same family (sRR) at a free stream Mach number of 8 is examined. Under the design conditions, the outermost heat flux peak is reduced by 80 % compared with the baseline case. The heating reduction capabilities of the model under varying free stream Mach numbers and sideslip angles are also evaluated, confirming its robustness under undesigned operating scenarios.
Article updated 7 January 2025.
Please note a has been issued for this article.